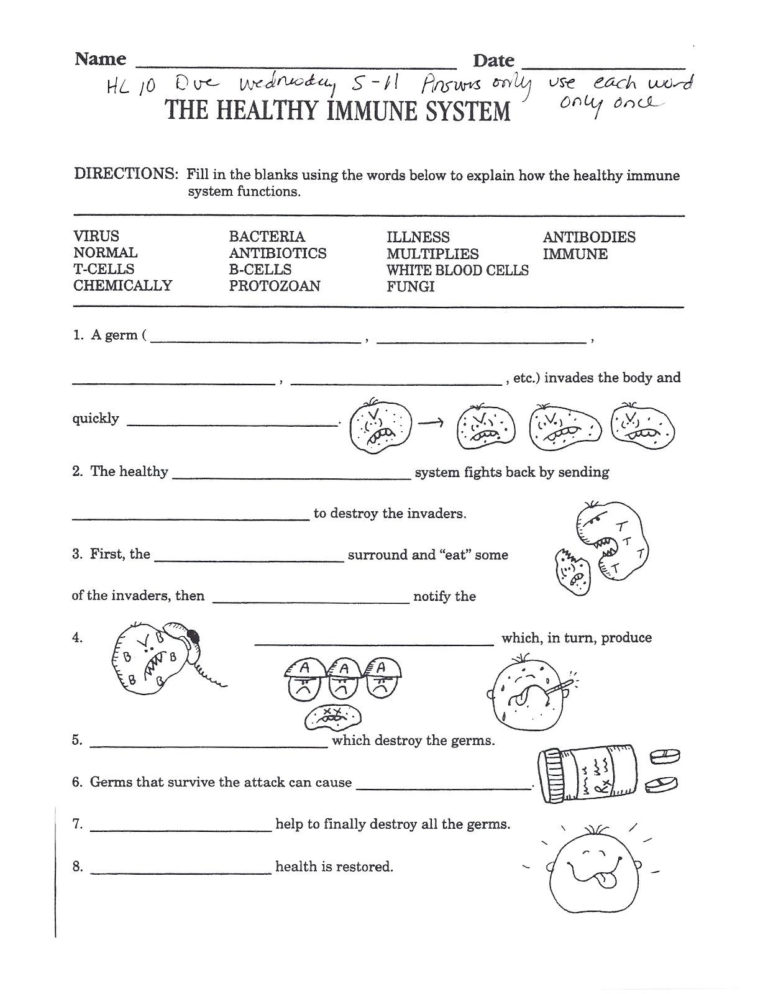
Exponential Growth and Decay Problems: Algebra 2 Worksheet

In mathematics, exponential functions are essential for understanding many natural phenomena, from population growth to radioactive decay. This post will delve into exponential growth and decay, focusing on problems commonly encountered in Algebra 2. Whether you're a student studying for your Algebra 2 exams or someone looking to brush up on your algebra skills, this post will provide comprehensive insights into these concepts, along with examples and solutions to common problems.
Understanding Exponential Functions

Exponential functions have the general form:
y = abx
- a: initial value or y-intercept.
- b: base of the exponential function, where b > 0 and b ≠ 1.
- x: the exponent, usually representing time or any independent variable.
The behavior of the function depends largely on the value of b:
- If b > 1, the function represents exponential growth.
- If 0 < b < 1, it represents exponential decay.
Exponential Growth

Exponential growth occurs when a quantity increases at a constant percentage rate per unit of time. Common applications include:
- Population growth.
- Compound interest.
- Spread of diseases.
Example Problem: Population Growth

A bacterial population starts with 1000 bacteria and doubles every two hours. Write an equation for the population as a function of time.
| Time (t) | Population (P) |
|---|---|
| 0 | 1000 |
| 2 | 2000 |
| 4 | 4000 |

The growth factor (b) here is 2 because the population doubles every two hours. Since t is in terms of hours, and doubling occurs every two hours, we define our time unit as two-hour intervals:
- Initial population, a = 1000
- Growth factor, b = 2
The equation would be:
P(t) = 1000 * 2t/2
📝 Note: Here, t/2 represents the number of doubling periods in hours.
Exponential Decay
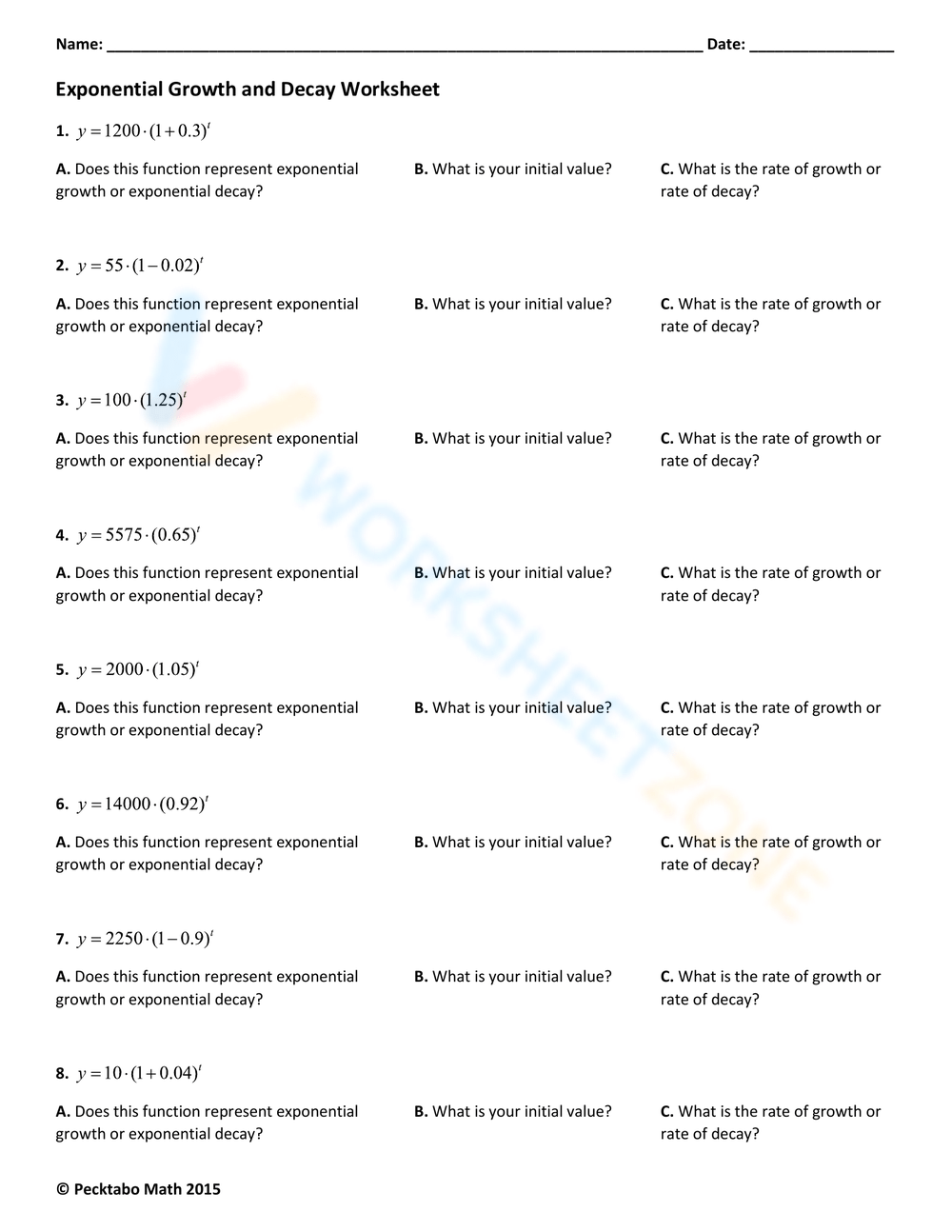
Exponential decay occurs when a quantity decreases at a constant percentage rate per unit of time. Typical examples include:
- Radioactive decay.
- Depreciation of assets.
- Carbon dating.
Example Problem: Radioactive Decay

Carbon-14 decays at an approximate rate of 5730 years for half-life. If a sample starts with 50 grams, how much remains after 10,000 years?
- Initial quantity, a = 50g
- Decay factor, b = 0.5
- Time, t = 10,000 years
- Half-life, k = 5730 years
Using the decay equation:
Q(t) = a * (b)t/k
Substitute the values:
Q(10000) = 50 * (0.5)10000⁄5730 ≈ 5.6 grams
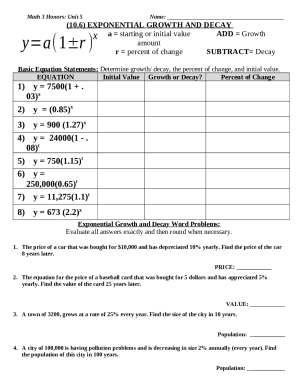
Problem Solving with Exponential Functions

Here are some general steps to solve exponential growth and decay problems:
- Identify the initial quantity, a.
- Determine the rate of change, b.
- Set up the exponential equation based on whether it’s growth or decay.
- Substitute given values for time and solve for the unknown.
Applications in Real Life
Exponential functions are not just theoretical; they’re critical for:
- Finance: Calculating compound interest.
- Biology: Understanding population dynamics.
- Medicine: Decay of medication in the body.
- Economics: Growth of economies.
In summary, exponential growth and decay play a fundamental role in various fields, modeling changes that occur at a constant rate relative to the current amount. By understanding these functions, students can better grasp how quantities evolve over time and how natural processes can be quantified and predicted. Whether it's the growth of a bacterial culture or the decay of a radioactive isotope, the principles remain the same.
To enhance your understanding and preparation for Algebra 2, practice solving different types of exponential problems, focusing on the relationships between the growth/decay factor, the initial value, and the passage of time.
What is the difference between exponential growth and exponential decay?

+
Exponential growth represents an increase in quantity where the rate of change is proportional to the current amount, typically with a base (growth factor) greater than 1. Exponential decay, conversely, models a decrease, with the rate of change being inversely proportional to the amount, with a base (decay factor) between 0 and 1.
How do you calculate the time for a quantity to double or halve?

+
For doubling time (growth), use the formula t = ln(2) / ln(b), where ‘b’ is the growth factor. For halving time (decay), it’s t = ln(0.5) / ln(b), where ‘b’ is the decay factor.
Can exponential functions be used to model real-world phenomena?

+
Absolutely, exponential functions are vital in modeling phenomena like population growth, radioactive decay, investment growth, and the spread of diseases among others.