5 Ways to Master Radical Arithmetic with Worksheets
Mastering radical arithmetic can initially seem daunting, especially if you are new to algebra or have struggled with mathematical concepts in the past. Radicals, or roots, are fundamental to understanding higher mathematical principles and are indispensable for fields like engineering, physics, and computer science. This guide introduces five engaging methods to learn and master radical arithmetic, focusing on both understanding and application through well-designed worksheets.
1. Understanding the Basics

Before diving into complex operations, one must firmly grasp the basics:
- Square Roots: The process of finding a number which when squared gives the original number. For example, √16 = 4 because 4 x 4 = 16.
- Cube Roots: Finding a number which when cubed gives the original. Example: 3√27 = 3 because 3 x 3 x 3 = 27.
- Higher Order Roots: Such as the fourth or fifth roots, though less common, still crucial in some fields.
Worksheets at this stage would involve identifying the root of numbers, performing basic operations with roots, and recognizing radical expressions.
✅ Note: When working on worksheets, use a calculator for verification but try solving problems manually to enhance understanding.
2. Simplifying Radical Expressions
Once the basics are clear, the next step is simplifying radicals. Here are some strategies:
- Factorization: Breaking numbers into their prime factors to simplify the expression.
- Rationalizing the Denominator: In fractions, eliminating radicals from the denominator through multiplication by the conjugate.
- Distributive Property: Using this property to simplify expressions where radicals are being multiplied.
Worksheets should challenge learners with increasingly complex expressions to practice and solidify these skills.
| Expression | Simplified Form |
|---|---|
| √50 | √2 * √25 = 5√2 |
| (2√3)(4√3) | 8 * √(3 * 3) = 8 * 3 = 24 |
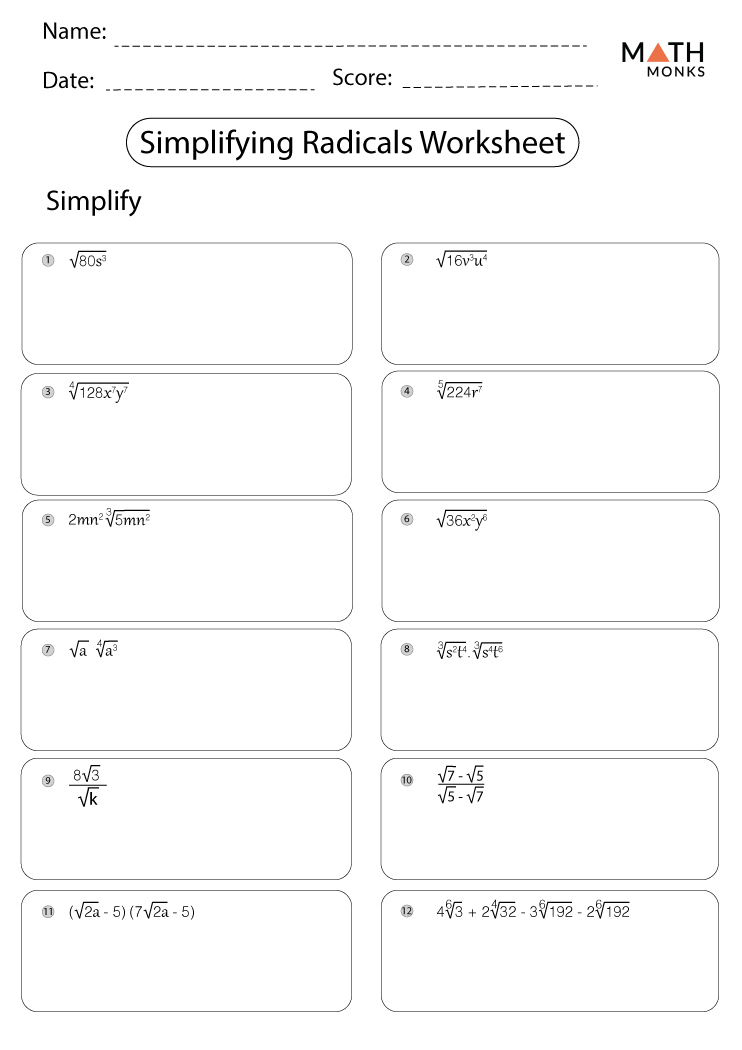
3. Solving Equations with Radicals

Here, the focus shifts to equations where radicals are involved:
- Isolate the Radical: Move all terms with radicals to one side of the equation.
- Square or Cube Both Sides: Depending on the degree of the radical, to eliminate the radical.
- Check Solutions: Since squaring or cubing can sometimes introduce extraneous solutions, verify each potential answer.
Worksheets in this category should include both simple and complex equations for comprehensive learning.
4. Operations with Radicals

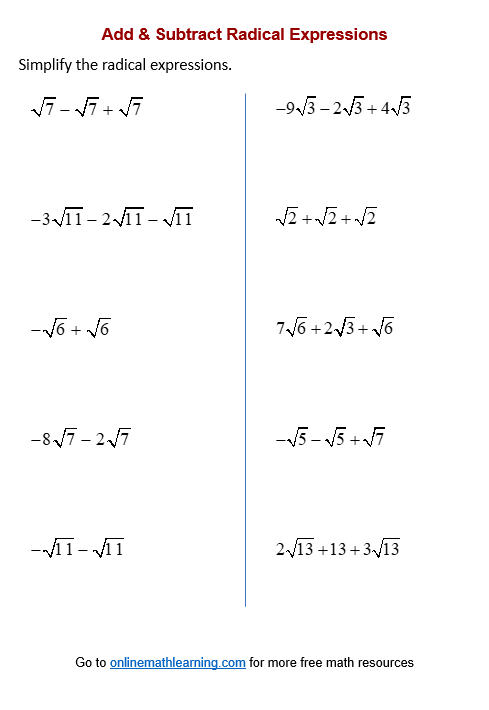
This step involves performing arithmetic operations like addition, subtraction, multiplication, and division with radicals:
- Addition/Subtraction: Only possible when the terms inside the radicals are the same (like combining like terms).
- Multiplication/Division: Use the rules of exponents or factorization to simplify.
Worksheets here would focus on various types of operations, helping students understand when and how to apply these rules.
5. Applications and Problem Solving

Understanding radicals in a real-world context or within mathematical problems enhances comprehension:
- Geometry: Finding lengths of sides or diagonals in geometric shapes.
- Physics: Calculations involving force, energy, and velocity often use radical expressions.
- Word Problems: From engineering to finance, problems often require radicals for solutions.
Worksheets would simulate real-world scenarios where radical arithmetic becomes essential, fostering critical thinking and problem-solving skills.
By now, the journey through these steps has not only provided a technical understanding of radicals but has also built confidence in handling these expressions. Mastering radical arithmetic is about more than just solving equations; it's about developing an intuitive understanding of how numbers relate to each other in complex, beautiful patterns. This proficiency with radicals will serve as a strong foundation for tackling more advanced algebraic concepts and enhancing problem-solving abilities across various fields of study.
What is a radical expression?

+
A radical expression is an expression that involves a root of a number or variable, typically denoted by the symbol √ for square roots and higher roots like 3√ for cube roots.
How can you simplify radicals?

+
Radicals can be simplified by factoring the radicand (the number inside the radical) into its prime factors, then pulling out any factor that can be raised to the power of the root outside the radical.
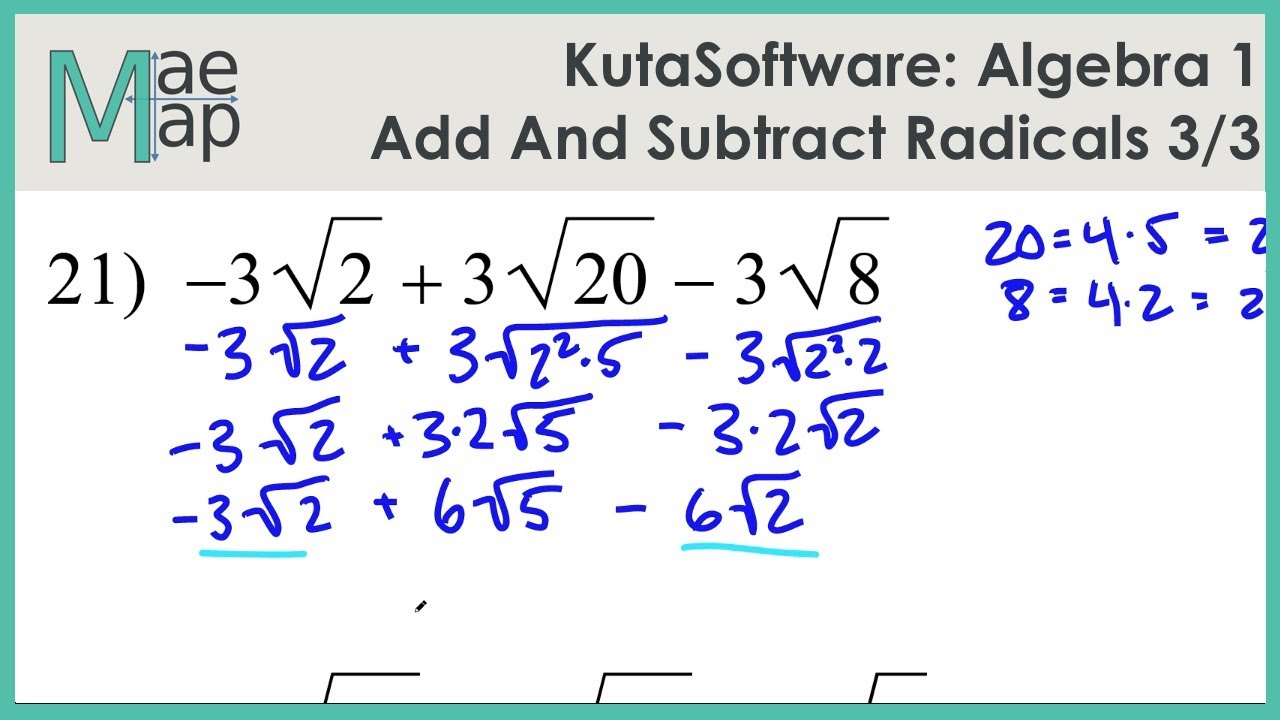
Can radicals be added or subtracted directly?
+
Radicals can only be added or subtracted if the terms inside the radicals are identical. Otherwise, they must be simplified or converted into a common form before addition or subtraction can occur.
Why is it important to check solutions when solving equations with radicals?

+
Squaring both sides of an equation can introduce extraneous solutions, which are not solutions to the original equation. Checking the answers ensures that only the correct solutions are accepted.
How are radicals used in real life?
+
Radicals are used in various applications including engineering (calculating stress and strain), architecture (determining distances and heights), finance (calculating interest rates), and physics (equations involving velocities, forces, and energy).