5 Essential Tips for Solving Exponential Problems

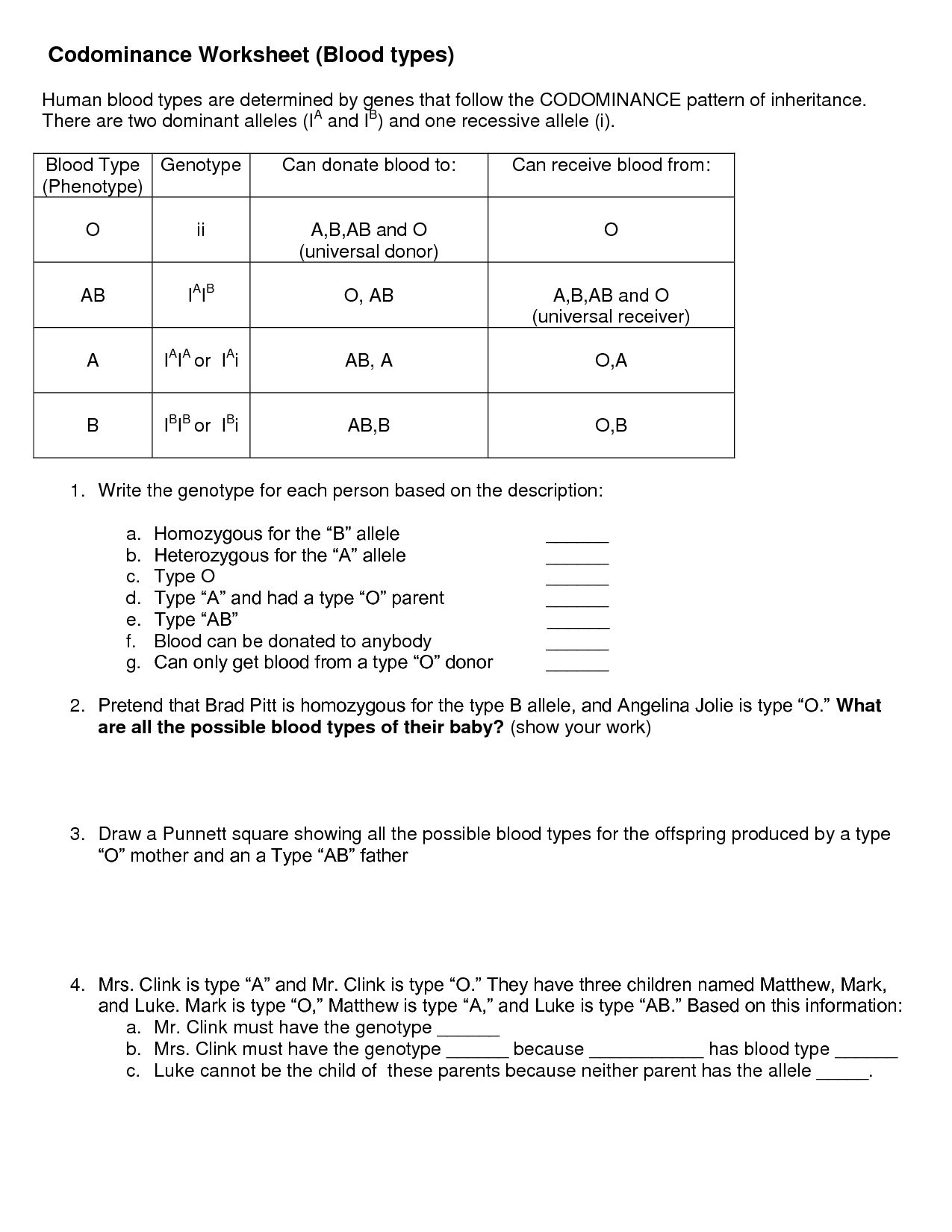
Exponential functions are prevalent in various fields of study, from mathematics and physics to finance and biology. Understanding how to work with and solve these problems is crucial for anyone looking to delve into complex systems or predictive modeling. Here are five essential tips to help you tackle exponential problems effectively, ensuring you have the tools and knowledge to handle these mathematical challenges with confidence.
Understanding the Exponential Function
Before we jump into solving, it’s essential to grasp what an exponential function is. At its core, an exponential function is of the form f(x) = ab^x, where:
- a is the initial value or the y-intercept when x = 0.
- b is the base, which must be positive and not equal to 1. It determines the growth rate (if b > 1) or decay rate (if 0 < b < 1).
- x is the independent variable, often representing time in real-world problems.
📌 Note: The exponential function’s property of having a constant ratio between consecutive values makes it particularly useful for modeling phenomena where quantities increase or decrease at a constant rate.
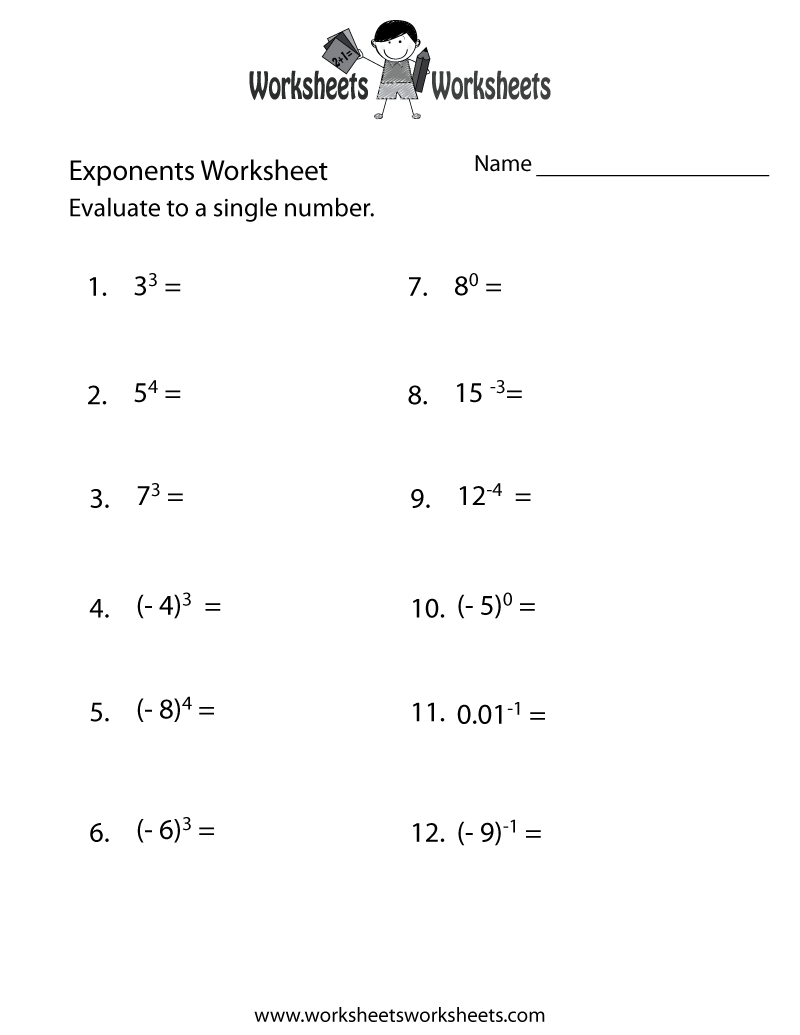
Mastering the Properties of Exponents

To solve exponential problems, you need to be familiar with the following properties:
| Property | Description |
|---|---|
| (a^m)(a^n) = a^(m+n) | When multiplying like bases, add the exponents. |
| (a^m)/(a^n) = a^(m-n) | When dividing like bases, subtract the exponents. |
| (a^m)^n = a^(mn) | When raising a power to a power, multiply the exponents. |
| (ab)^m = a^m * b^m | When raising a product to a power, raise each factor separately. |

Understanding these properties allows you to simplify and solve exponential expressions more efficiently.
Logarithms: A Key to Unlocking Exponential Equations
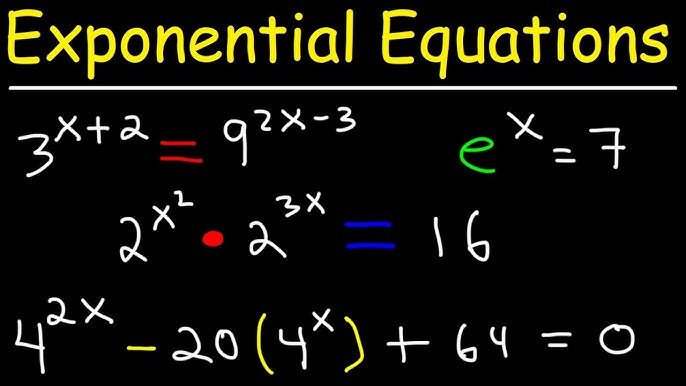
Logarithms and exponential functions are inverse operations; you can use logarithms to solve equations where the variable is in the exponent:
- Use log_a(x) = y to mean a^y = x.
- Remember that log_a(a^x) = x, which often helps in isolating variables.
By applying logarithms, you can transform exponential equations into linear equations, making them easier to solve:
Example: To solve 5^x = 32, take the logarithm on both sides:
log(5^x) = log(32) x * log(5) = log(32) x = log(32) / log(5)
Solving Exponential Growth and Decay Problems
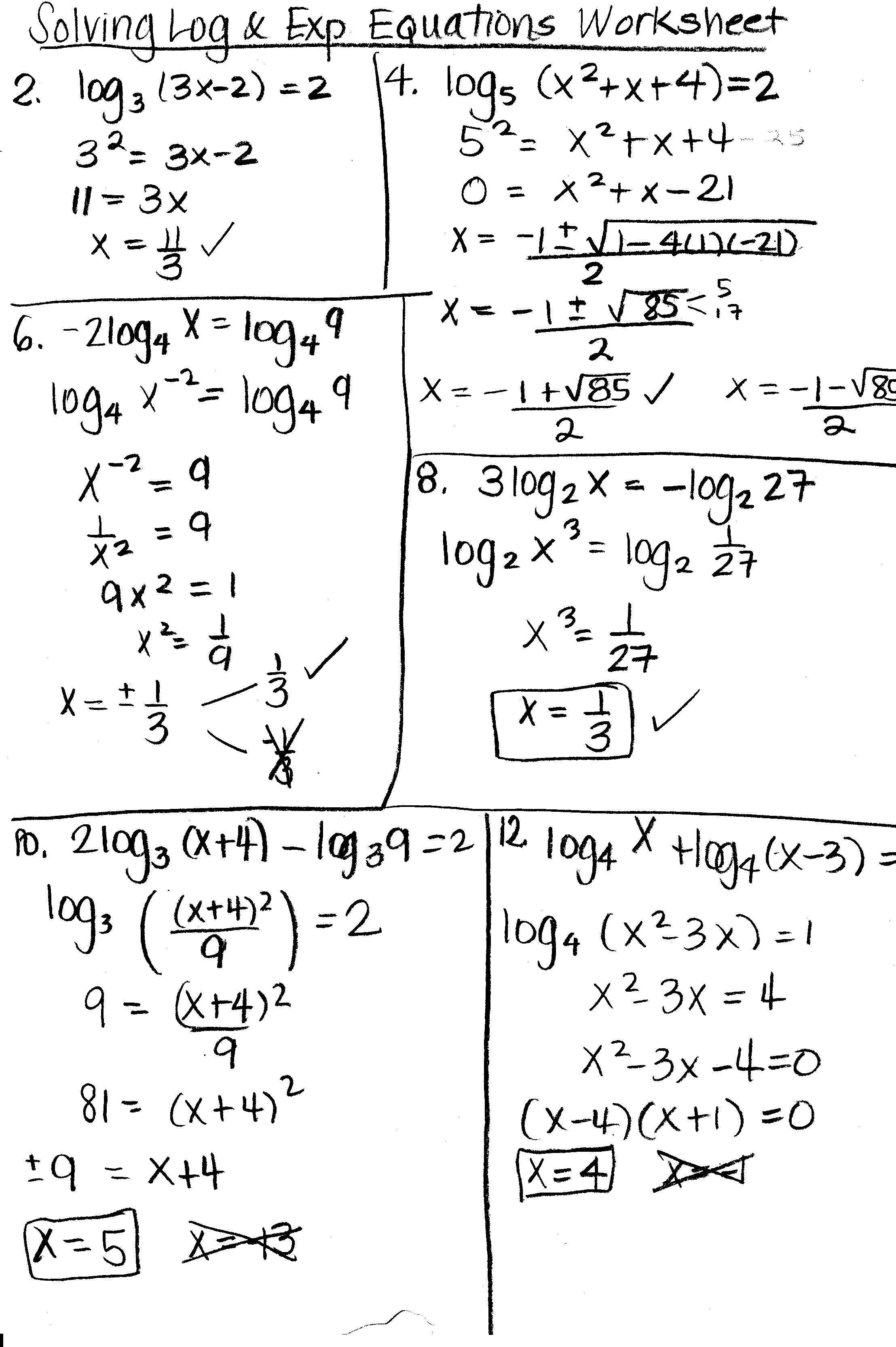
Exponential growth and decay problems are common in real-world applications, especially when dealing with populations, investments, and chemical reactions. Here are key steps:
- Identify the initial value a and the rate b.
- If dealing with continuous growth or decay, use e^x instead of b^x (where e ≈ 2.71828).
- Set up the equation based on whether it’s growth or decay. For growth, Q(t) = Q_0 * e^(rt), and for decay, Q(t) = Q_0 * e^(-rt).
- Compound Interest: Use the formula A = P(1 + r/n)^(nt) where A is the amount of money accumulated, P is the principal amount, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time the money is invested or borrowed for.
- Population Growth: Use logistic models like P(t) = K / (1 + e^(-rt)) where K is the carrying capacity of the environment.
🌱 Note: For exponential decay, ensure you use a negative sign in the exponent, as this reflects the decreasing nature of the function.
Practice with Real-World Applications
Exponential problems are not just theoretical exercises; they’re vital for real-world applications:
Applying these formulas in practical scenarios not only reinforces your understanding but also prepares you for real-life problem-solving.
In closing, these five tips are a comprehensive toolkit for anyone looking to improve their proficiency with exponential functions. From recognizing the core structure of an exponential function to leveraging properties of exponents and logarithms, through to practical application, mastering these concepts will significantly boost your mathematical prowess. Exponential problems are not just about number manipulation; they’re about understanding how rapid changes occur and using this knowledge to predict and model various scenarios in the real world.
What is the significance of the base in exponential functions?

+
The base in an exponential function represents the growth or decay rate. If the base is greater than 1, the function models exponential growth, whereas if it’s between 0 and 1, it models exponential decay.
Why are logarithms useful in solving exponential equations?

+
Logarithms allow us to transform exponential equations into linear equations. This is because logarithms and exponentials are inverse operations; you can take the logarithm of both sides to isolate the exponent, simplifying the problem-solving process.
How can you determine if a problem involves exponential growth or decay?

+
To determine this, examine the context of the problem. If the quantity is increasing at a constant rate, it’s exponential growth. If it’s decreasing at a constant rate, it’s exponential decay. Additionally, check if the base in the exponential function is greater than 1 (growth) or between 0 and 1 (decay).
Can exponential functions model real-world phenomena accurately?

+
Exponential functions are extremely useful for modeling phenomena that increase or decrease at a fixed rate over time, like population growth, compound interest, chemical reactions, or radioactive decay. However, real-world scenarios often require adjustments like logistic models for more accurate representations due to limitations or environmental constraints.