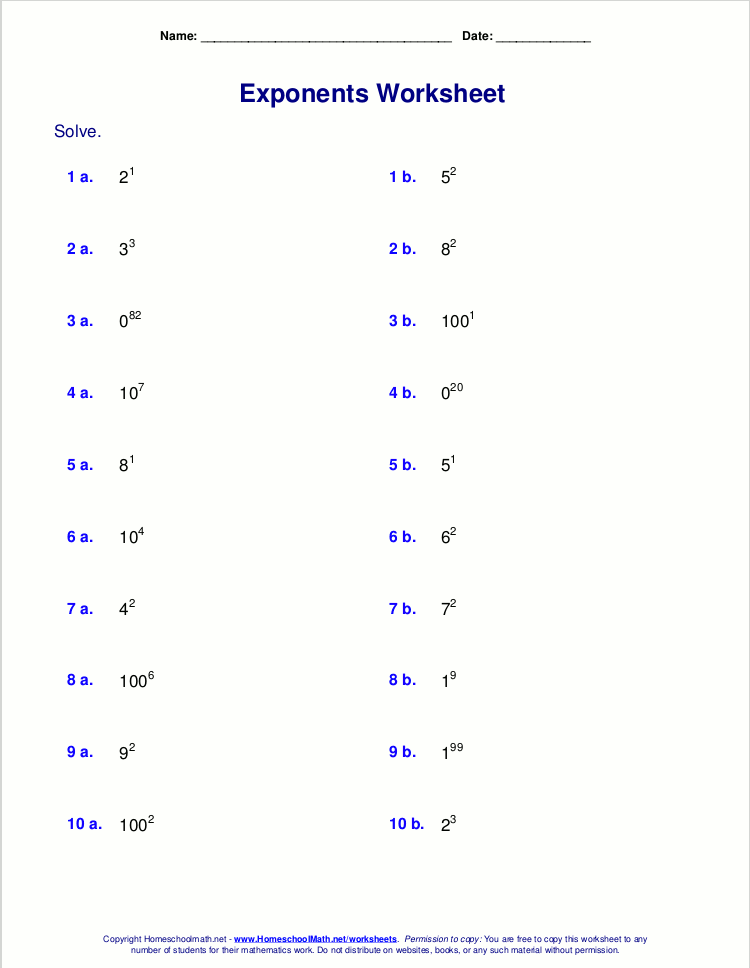
Master Exponent Rules with Our Free Worksheets

Understanding exponent rules is fundamental in various branches of mathematics, from algebra to calculus. While the concept of exponents might appear straightforward, mastering these rules can significantly enhance your proficiency in problem-solving and mathematical fluency. Let's delve deep into the realm of exponents, exploring their rules, and offering free worksheets to practice these rules effectively.
What are Exponents?

Exponents are a shorthand method to indicate repeated multiplication of a number by itself. If you see the expression an, a is called the base, and n is the exponent or power. Essentially, it tells you to multiply a by itself n times. For instance:
- 23 = 2 × 2 × 2 = 8
- (-3)4 = (-3) × (-3) × (-3) × (-3) = 81
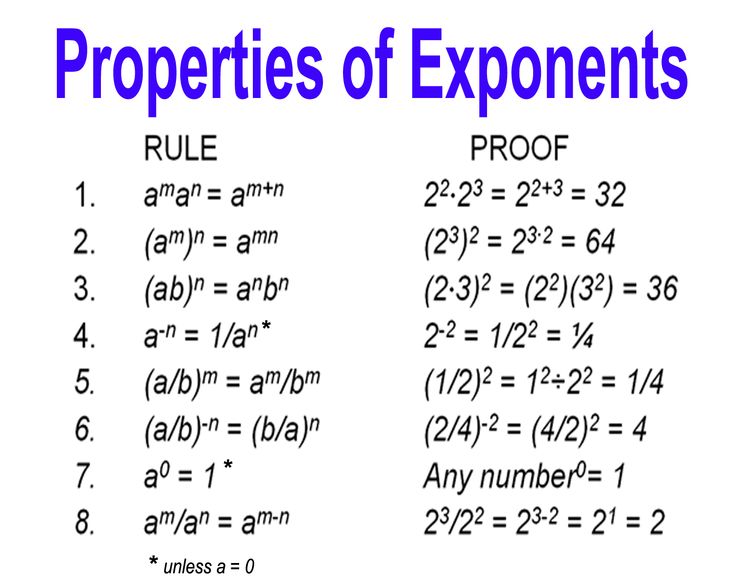
Basic Rules of Exponents

Here are the essential rules you need to understand:
1. The Product Rule:
When multiplying numbers with the same base, you add the exponents:
[ a^m \times a^n = a^{m+n} ]For example, 32 × 33 = 32+3 = 35
2. The Quotient Rule:

When dividing numbers with the same base, you subtract the exponents:
[ \frac{a^m}{a^n} = a^{m-n} ]So, (\frac{5^6}{5^4} = 5^{6-4} = 5^2).
3. The Power Rule:

When raising an exponent to another power, multiply the exponents:
[ (a^m)^n = a^{m \times n} ]As an example, (23)2 = 23 \times 2 = 26
4. Zero Exponent Rule:

Any non-zero number to the power of 0 equals 1:
[ a^0 = 1 ]This rule does not apply to zero itself (00 is an indeterminate form).
5. Negative Exponents:

A negative exponent means the reciprocal of the number with a positive exponent:
[ a^{-n} = \frac{1}{a^n} ]For example, 4-2 = (\frac{1}{4^2}) = (\frac{1}{16}).
6. Fractional Exponents:

A fractional exponent indicates taking a root:
[ a^{m/n} = \sqrt[n]{a^m} ]Here, (3^{1⁄2}) represents the square root of 3.
7. Distribution of Exponents:

When raising a product or quotient to an exponent, each part is raised to that exponent:
[ (ab)^n = a^n \cdot b^n ] [ \left(\frac{a}{b}\right)^n = \frac{a^n}{b^n} ]Using Our Free Exponents Worksheets

Our free exponents worksheets are designed to reinforce these rules through varied practice:
- Identify and Apply Rules: These exercises help you spot which rule applies to a given problem.
- Simplification: Practice simplifying expressions using the rules of exponents.
- Problem Solving: Apply exponents in context by solving word problems.
🔍 Note: Each worksheet includes a legend that summarizes the exponent rules, making it a handy reference tool while practicing.
Strategies for Learning Exponent Rules
Here are some strategies to master exponent rules:
- Memorize the Rules: Use mnemonic devices or visual aids to remember the formulas.
- Practice Regularly: Consistent practice with worksheets enhances understanding and speed.
- Conceptual Understanding: Relate rules to real-life contexts or algebra for deeper insight.
- Use Technology: Explore online tools and games for interactive learning.
As we wrap up, remember that mastering exponents rules is a journey of consistent practice and understanding. They are not just mathematical shortcuts but a crucial part of understanding higher mathematics. These free worksheets provide a structured path to proficiency, ensuring that you're well-equipped to tackle problems involving exponents.
Lastly, here are some common queries related to exponents:
Why do exponents change when multiplying or dividing numbers?

+
Exponents represent repeated multiplication, so when you multiply or divide numbers with the same base, you essentially adjust the number of times you multiply that base by itself, hence adding or subtracting the exponents respectively.
How can negative exponents be positive?

+
Negative exponents indicate moving from the numerator to the denominator in a fraction, essentially making the exponent positive by expressing the base in terms of its reciprocal.
What’s the point of learning exponents?

+
Exponents simplify expressing very large or very small numbers and are foundational for algebraic manipulations, scientific notation, and many other mathematical operations in fields like physics, chemistry, and finance.



