5 Essential Exponent Laws to Master Quickly

Exponents, also known as powers, are fundamental in algebra and higher mathematics. They provide a shorthand way of expressing multiplication operations that involve repeated factors. Understanding exponent laws is crucial for simplifying expressions, solving equations, and working with complex mathematical concepts. Here, we dive into five essential exponent laws that can help you quickly master the basics:
1. The Product Rule


The product rule of exponents states that when multiplying two exponents with the same base, you add their exponents:
- If am * an = am+n
Where a is any real number and m and n are positive integers. Here are some examples:
- 23 * 24 = 23+4 = 27 = 128
- x5 * x2 = x5+2 = x7
🔄 Note: This rule applies even if the base is a variable or a negative number.
2. The Quotient Rule


The quotient rule tells us how to divide two exponents with the same base by subtracting their exponents:
- am / an = am-n (provided a ≠ 0)
Here are a couple of examples:
- 108 / 103 = 108-3 = 105 = 100000
- (x6 / x4) = x6-4 = x2
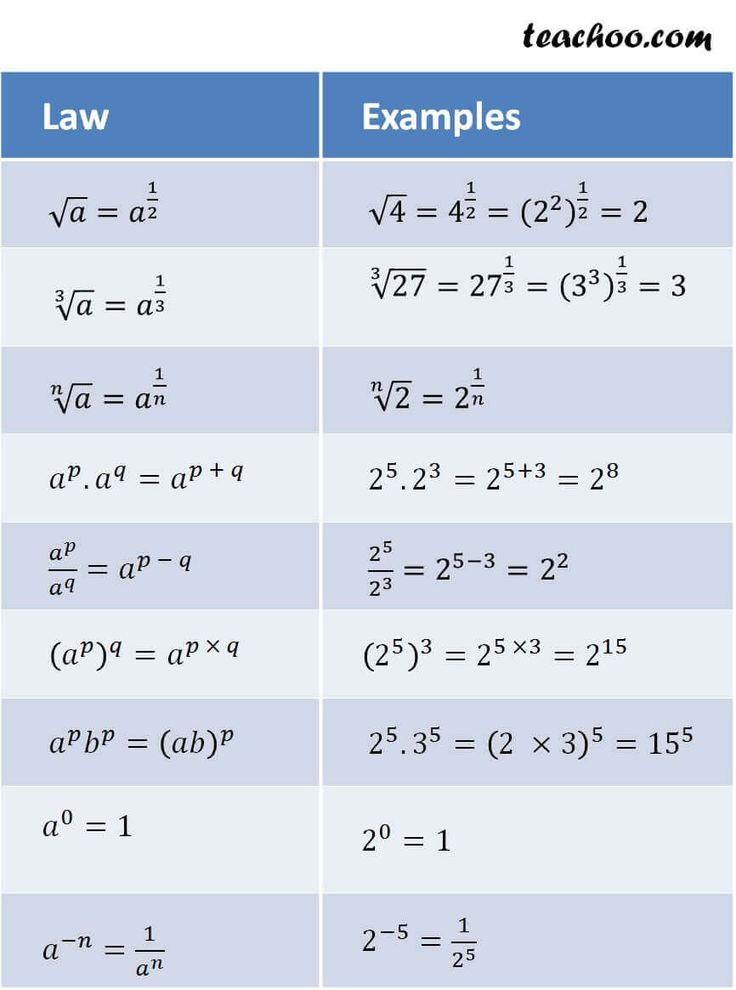
3. The Power Rule


This law involves raising an exponent to another power:
- (am)n = amn
Consider these examples:
- (32)3 = 32*3 = 36 = 729
- (xy)z = xyz
This rule can be visualized with the table below:
| Base | Exponent | New Exponent | Result |
|---|---|---|---|
| 3 | 2 | 3 | 36 = 729 |
| x | y | z | xyz |

4. The Zero Exponent Law


Any non-zero number raised to the power of zero equals one:
- a0 = 1 (provided a ≠ 0)
This law can be counterintuitive but simplifies expressions significantly:
- 50 = 1
- x0 = 1 (for x ≠ 0)
5. The Negative Exponent Law


Negative exponents indicate a reciprocal:
- a-n = 1/an (where a ≠ 0)
Examples:
- 2-3 = 1⁄23 = 1⁄8
- x-y = 1/xy
Having explored these five essential exponent laws, we can see how they provide a framework for understanding and simplifying complex expressions. These laws are not only fundamental in algebra but are also essential in calculus, physics, and other scientific fields. By mastering these rules, one can simplify calculations and grasp the underlying principles of exponentiation. As you move forward, remember that:
- Combining these laws can result in even more powerful algebraic manipulation techniques.
- Understanding exponent laws also helps in deciphering logarithms, their inverses.
Wrap-Up: To really master exponents, you'll need to practice applying these laws, often in combination. Whether you're simplifying, solving for variables, or understanding the mechanics behind exponential growth, these exponent laws are your key to success.
What is the purpose of exponent laws in algebra?

+
Exponent laws provide a systematic way to manipulate and simplify expressions involving powers, making algebra more manageable and intuitive.
Can the exponent laws be applied to negative bases?

+
Yes, the laws apply to negative bases, but you must be careful with signs when raising to a power or when dealing with the zero exponent.
Why does any number raised to the power of zero equal one?
+
This rule stems from the consistency of exponents when considering division by powers of the same base. It ensures that a0/an = a-n holds true.
What are some real-world applications of these exponent laws?

+
Exponent laws are used in finance (compound interest), population growth models, physics (exponential decay), and computer science for algorithm analysis.