5 Tips for Mastering Exponential Functions Worksheet
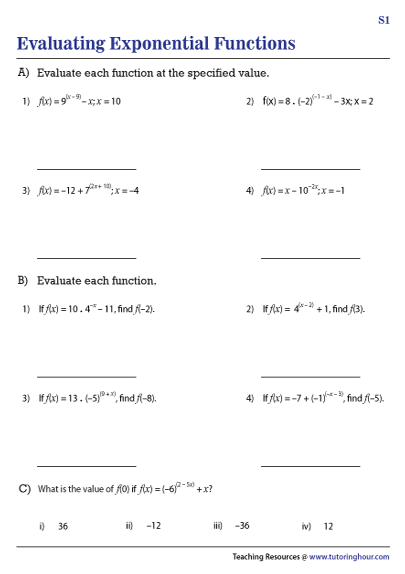
Exponential functions are a fundamental part of mathematical and scientific studies, often encountered in physics, biology, economics, and more. Whether you're a student tackling your curriculum or a professional dealing with predictive analytics, understanding exponential functions is not just about solving equations but also about grasping the underlying principles of growth and decay. Here are five tips to help you master the exponential functions worksheet.
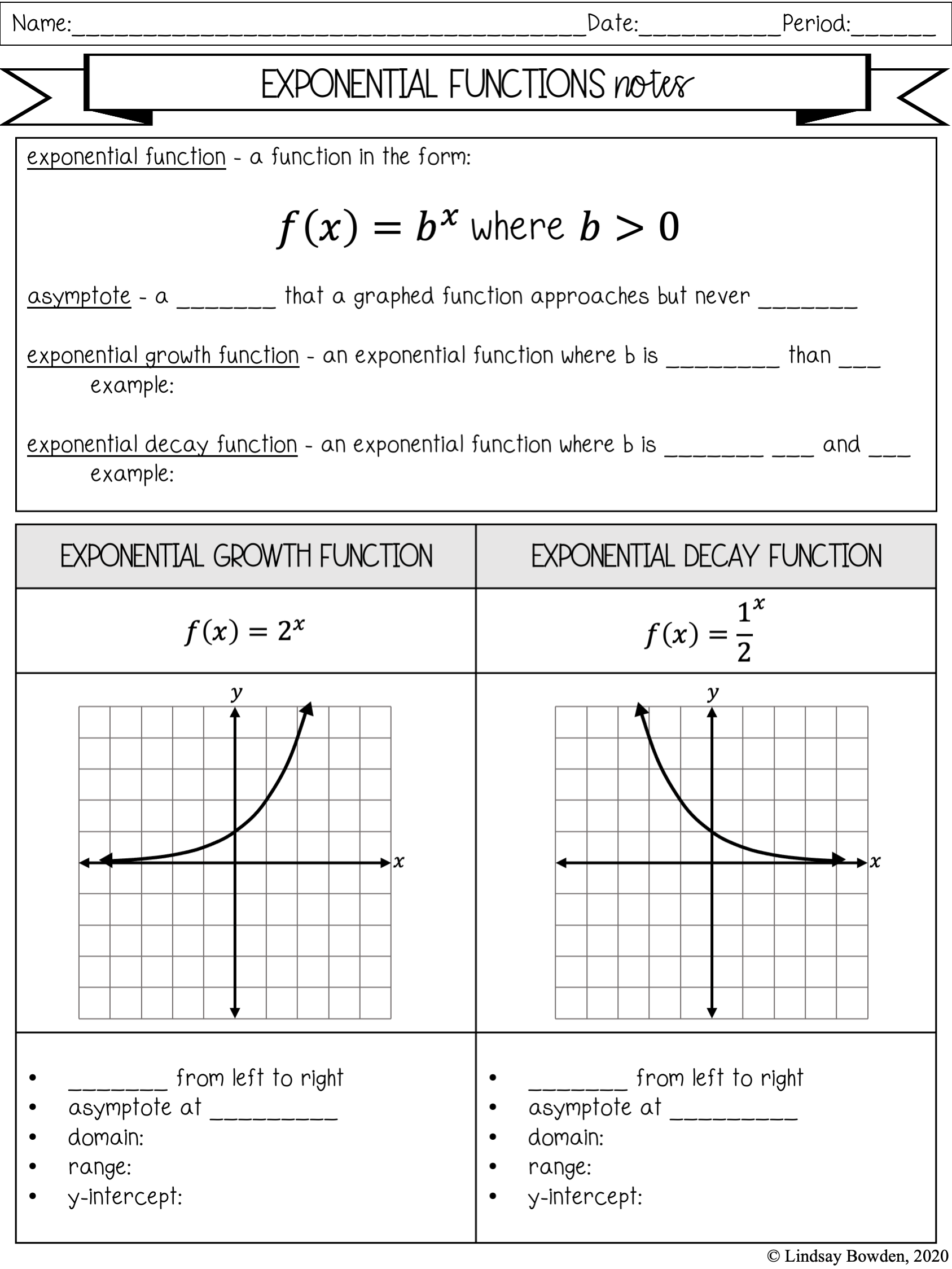
Understand the Basics

Start with understanding what an exponential function is. It’s typically expressed in the form (y = ab^x), where:
- a is the initial amount
- b is the base or growth factor
- x is the exponent or time variable
The function describes how a quantity grows or decays at a rate proportional to its current value. Here’s what you need to do:
- Get comfortable with exponents. Practice operations with exponents to handle exponential expressions with ease.
- Learn the properties of exponents, such as (a^m \cdot a^n = a^{m+n}), (\frac{a^m}{a^n} = a^{m-n}), and ((a^m)^n = a^{mn}).
💡 Note: Remember that (b > 0) and (b \neq 1) for the function to be exponential.
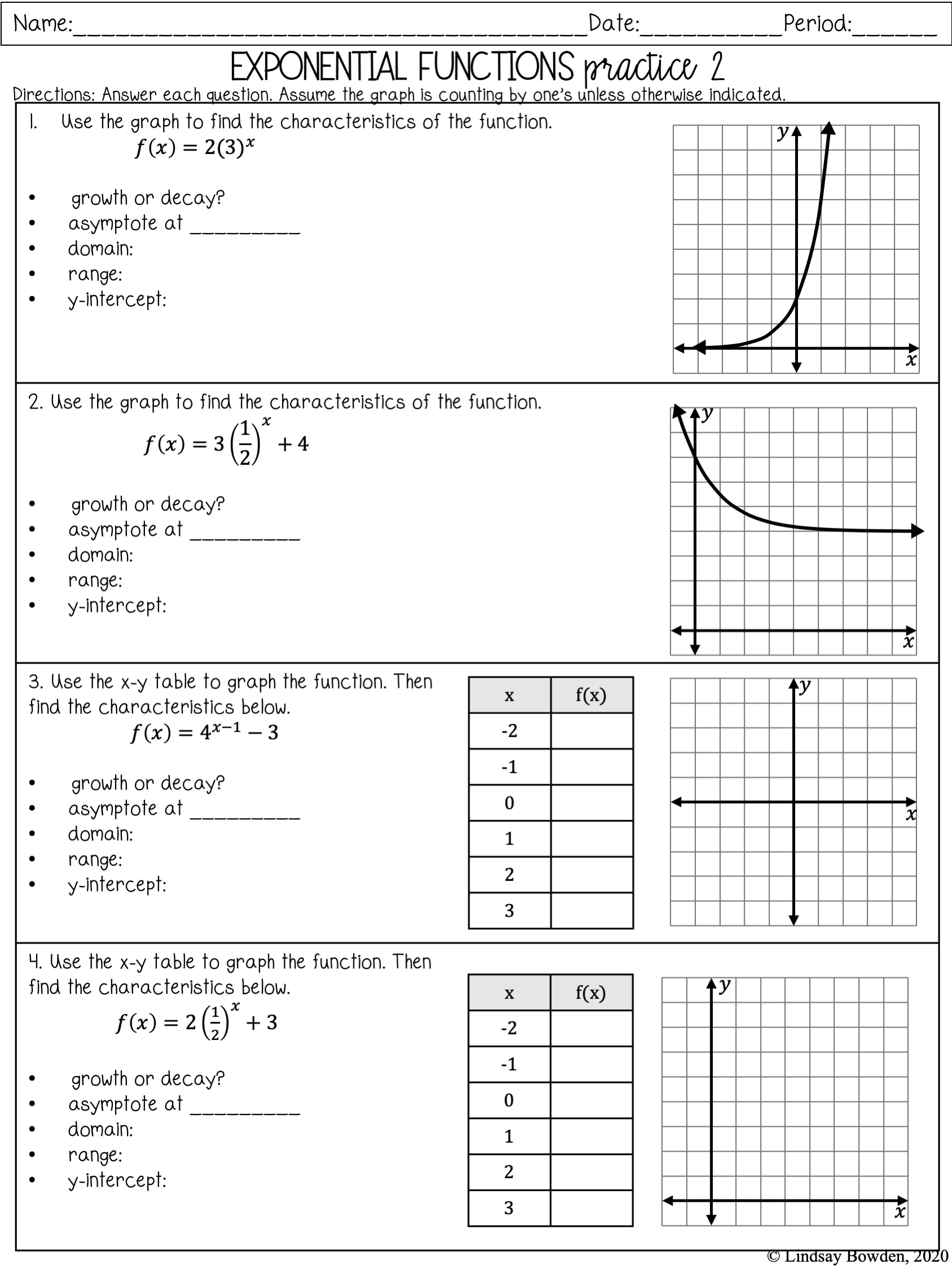
Graphing Exponential Functions


Visual representation can be incredibly enlightening:
- Identify the asymptote: Typically, for functions like (y = ab^x), there will be a horizontal asymptote at (y = 0) (if (a \neq 0)), and for functions like (y = \frac{a}{x}), there’s a vertical asymptote at (x = 0).
- Plot key points: Find (y)-intercepts, points of growth, and any significant points that help in sketching the curve. For instance, if (a = 2) and (b = 3), plot (y = 2 \cdot 3^0 = 2), (y = 2 \cdot 3^1 = 6), etc.
- Recognize patterns: Exponential growth forms an S-curve when (b > 1) and a decay curve when (0 < b < 1).
Visualizing functions helps in understanding their behavior over time.
Solve Exponential Equations
When solving exponential equations, you’ll often need to:
- Take logarithms on both sides to isolate the exponent, e.g., to solve (2^x = 16), you can take (\log_2) of both sides to get (x = \log_2(16) = 4).
- Use logarithmic properties. For example, (\log (a^b) = b \log a), which can simplify solving equations.
- If dealing with compound interest or population growth, understanding and using the continuous exponential model (e^{kx}) can be helpful.
| Exponential Form | Logarithmic Form |
|---|---|
| [a = b^c] | [c = \log_b(a)] |
🔍 Note: Keep in mind that logarithms are inverses of exponential functions; understanding their relationship is key.
Analyze Exponential Growth and Decay

Exponential functions aren’t just about solving equations; they’re about understanding phenomena:
- Growth: If (b > 1), the function models growth scenarios like population increase, investment growth, or bacterial reproduction.
- Decay: If (0 < b < 1), the function models decay scenarios like radioactive decay, cooling, or population decline.
- Be able to identify the half-life or doubling time, which are key in understanding the rate of change.
Practice with Real-World Examples

Here are some real-world scenarios you can work on:
- Investment Growth: Calculate the future value of an investment or savings account using the compound interest formula (A = P(1 + \frac{r}{n})^{nt}), where (A) is the amount of money accumulated after n years, including interest, (P) is the principal amount, (r) is the annual interest rate (decimal), (n) is the number of times interest is compounded per year, and (t) is the time the money is invested for in years.
- Population Dynamics: Model population growth with the exponential growth model (P = P_0 e^{rt}), where (P) is the population after time (t), (P_0) is the initial population, (r) is the growth rate, and (t) is time.
- Decay Scenarios: Use exponential decay to model phenomena like radioactive decay ((N = N_0 e^{-\lambda t})), where (N) is the quantity of substance remaining after time (t), (N_0) is the initial quantity, (\lambda) is the decay constant, and (t) is time.
📚 Note: Real-world applications give context to the mathematical concepts, making them more relatable and easier to remember.
In summary, mastering the exponential functions worksheet involves a thorough understanding of exponents, the ability to graph these functions, problem-solving skills in solving exponential equations, analysis of growth and decay, and practical application through real-world examples. By keeping these tips in mind, you can navigate through exponential functions with more ease and proficiency, making this branch of mathematics not only manageable but also enjoyable. This understanding not only helps in excelling in your worksheet but also in grasping the essence of how our world changes exponentially.
What are the most common mistakes in solving exponential equations?

+
Common mistakes include not isolating the exponent, forgetting to apply the change of base formula correctly, and misusing logarithms. Always ensure to simplify both sides of the equation and apply the correct logarithmic operation before solving for (x).
How do I find the growth rate from an exponential function?

+
To find the growth rate, compare the function values at two different time periods. The formula for growth rate (r) is (\frac{P_t - P_0}{P_0}), where (P_0) is the initial value and (P_t) is the value after time (t).
Can exponential functions be applied to decrease?

+
Yes, exponential functions can model decay scenarios where the base (b) is between 0 and 1 ((0 < b < 1)). This represents a decrease in value over time, often seen in radioactive decay, depreciation, or population decline.
How do I differentiate an exponential function?

+
The derivative of an exponential function (f(x) = e^{ax}) is (f’(x) = a \cdot e^{ax}), where (a) is a constant.
What are some common exponential function applications?

+
Exponential functions are applied in:
- Compound interest calculations in finance
- Modeling population growth or decay
- Understanding radioactive decay in physics
- Describing growth patterns in biology
- Analyzing technological progress



