Function Table Answer Key: Unlock Your Math Skills
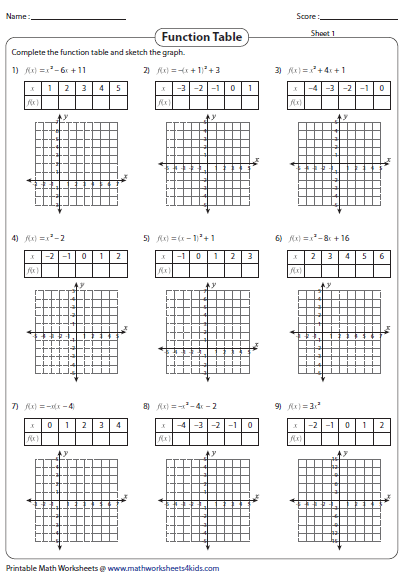
In the journey of mastering mathematics, one essential tool often overlooked is the function table. Function tables provide a clear and organized method to visualize and understand the relationship between input and output values in functions. This comprehensive guide will delve into the intricacies of function tables, explaining their setup, benefits, and various applications, ensuring that by the end, you'll have unlocked a key skill in your mathematical toolkit.
Understanding Function Tables
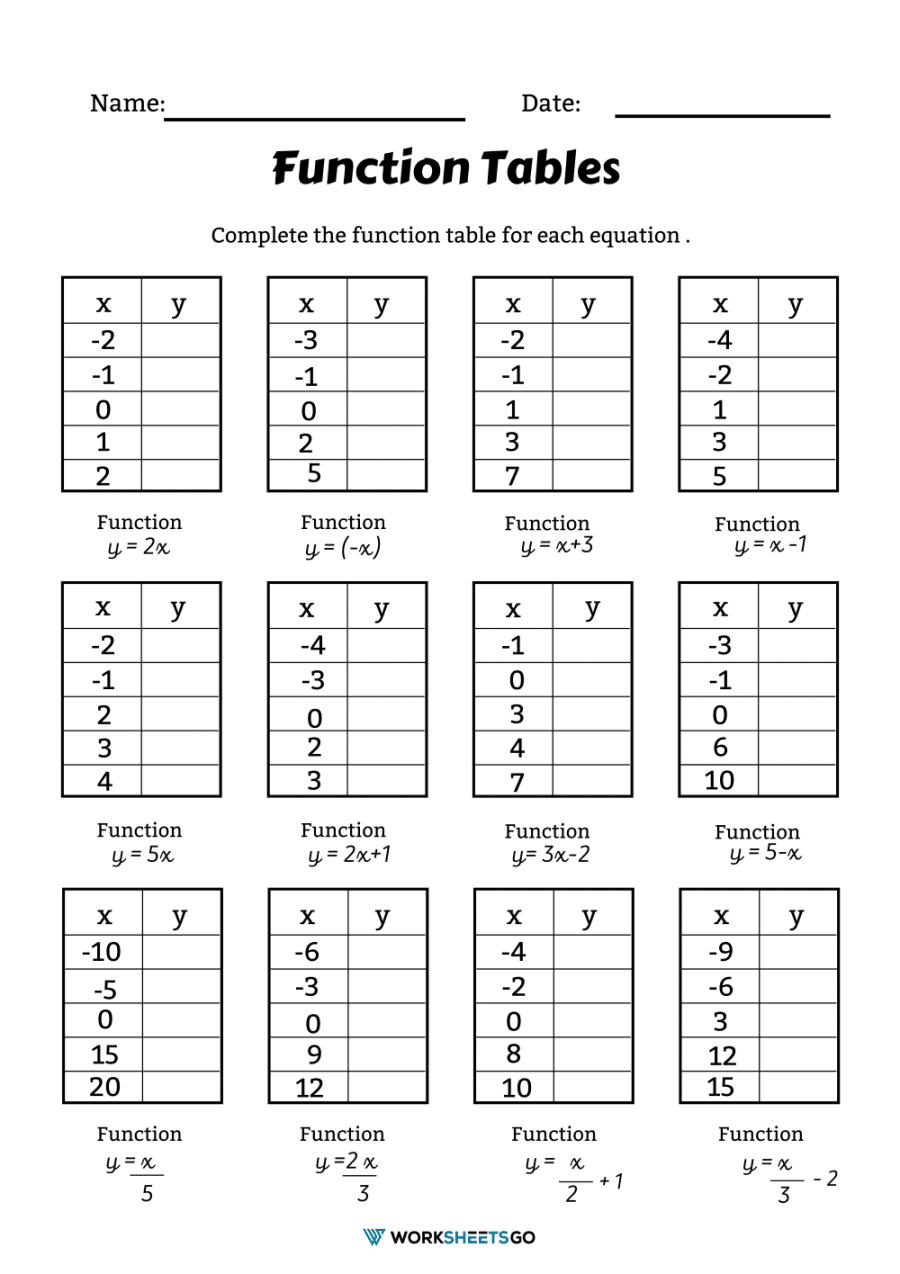
Function tables are a tabular representation where each row typically shows:
- An input value (or argument)
- The corresponding output (or function value) based on a given function
They help in:
- Visualizing how a function behaves for different inputs
- Understanding the patterns within the function's domain and range
- Quickly checking or predicting outputs for specific inputs without needing to solve the function manually each time
Setting Up a Function Table

Here's how to construct a basic function table:
- Choose a Function: Start with a mathematical function like f(x) = 2x + 3.
- Select Input Values: Pick a series of x-values, often starting from a low number up to a reasonable high number or in a pattern like 0, 1, 2, etc.
- Create the Table:
- First Column: Label as "x" for input values.
- Second Column: Label as "f(x)" for the output.
- Calculate Outputs: For each chosen input, calculate the corresponding output by applying the function.
| x | f(x) = 2x + 3 |
|---|---|
| 0 | 3 |
| 1 | 5 |
| 2 | 7 |
| 3 | 9 |
| 4 | 11 |

📝 Note: Ensure that the input values you select are within the domain of the function to avoid undefined or erroneous results.
Benefits of Using Function Tables

Function tables offer several advantages:
- Pattern Recognition: Easily spot the behavior of linear and non-linear functions.
- Error Detection: Quickly identify mistakes by seeing if results follow expected patterns.
- Enhanced Understanding: Grasping the relationship between variables becomes more intuitive.
Applications of Function Tables

Function tables are not just an academic exercise; they have practical uses:
- Education: Ideal for teaching students about functions, variables, and patterns.
- Engineering: For predicting outcomes of complex systems or control functions.
- Finance: Understanding the growth or decay models.
- Computer Science: In programming for function-based problems or algorithms.
Tips for Creating Effective Function Tables

To maximize the utility of your function tables:
- Select Relevant Inputs: Choose x-values that reflect real-world scenarios or important points in the function.
- Keep it Organized: Make sure your table is well-structured for easy reading and comparison.
- Use Color Coding or Highlighting: For visual learners, use colors or highlighting to distinguish inputs from outputs.
- Check Your Work: Always verify your calculations to ensure accuracy.
By consistently practicing with function tables, you'll develop a deeper understanding of mathematical relationships, predict function outcomes, and apply this knowledge to various fields of study or work. This skill not only simplifies complex problems but also makes you more proficient and confident in dealing with mathematical tasks.
Why should I use function tables?

+
Function tables visually represent how different inputs affect the outputs of a function, making it easier to understand and predict function behavior, detect patterns, and ensure accurate calculations.
What are common mistakes when creating function tables?

+
Common mistakes include miscalculating outputs, selecting inputs outside the function’s domain, or poor organization of the table leading to confusion in reading the data.
How do function tables help in real-world applications?

+
In real-world scenarios, function tables can be used to model growth or decay, predict financial outcomes, understand the behavior of control systems, and in numerous other applications where understanding how inputs affect outputs is crucial.



