5 Essential Tips for Mastering Angular Kinematics

Angular kinematics is a pivotal branch of physics and engineering that deals with the motion of objects in a circular path. Whether you're studying the physics behind the orbital motion of celestial bodies, the rotation of mechanical systems, or even the pivotal moments in a gymnastics routine, understanding angular kinematics is crucial. Here are five essential tips to help you master this intriguing subject:
1. Understand the Basics of Angular Motion

Angular motion isn't as straightforward as linear motion, but with the right conceptual framework, it becomes manageable. Here's what you need to grasp:
- Angular Displacement: This measures how much an object has moved from its initial angular position. Angular displacement, often symbolized by θ, is measured in radians or degrees.
- Angular Velocity: Given by the rate at which an object changes its angular displacement, symbolized by ω. It's commonly measured in radians per second.
- Angular Acceleration: This represents how quickly the angular velocity changes, denoted by α. Angular acceleration has units of radians per second squared.
- Relationships with Linear Motion: Understanding how angular measurements relate to linear ones via relationships like v = rω, where v is linear velocity, r is radius, and ω is angular velocity.
💡 Note: Understanding these fundamental concepts is key to moving forward with angular kinematics effectively.
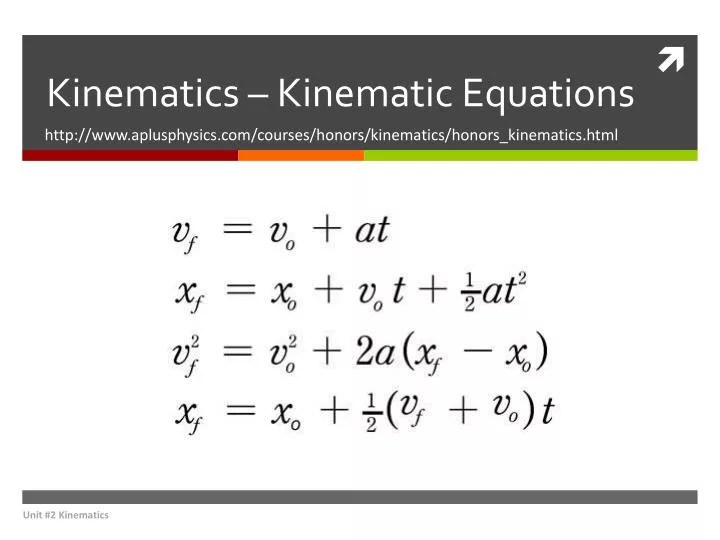
2. Learn and Apply the Kinematic Equations

Just as linear motion has its kinematic equations, angular motion does too. Here are the essential angular kinematic equations you should be familiar with:
- Angular Displacement:

- Angular Velocity:

- Angular Acceleration:

🔧 Note: Remember these equations are just like linear kinematic equations but adapted for rotational motion.
3. Visualize with Real-Life Examples

Angular kinematics becomes more accessible when you visualize real-life scenarios:
- Rotating Ferris Wheel: The angular velocity of the wheel directly correlates with the time it takes to complete one full rotation.
- Ice Skater Spinning: Angular momentum conservation explains why an ice skater spins faster when they pull in their arms.
- Cars Taking a Turn: The need for centripetal force in circular motion can be demonstrated by the feeling of being pushed outwards in a car when it turns.
🎯 Note: Connecting abstract concepts to tangible examples aids in comprehension and retention.
4. Develop Problem Solving Skills

Angular kinematics often involves solving complex problems. Here are some strategies:
- Choose the Right Equation: Depending on what you know and what you're solving for, choose the appropriate kinematic equation.
- Use Diagrams: Sketch out the situation to visualize which angles, velocities, and accelerations are in play.
- Understand Dimensional Analysis: Ensure your units align, which helps prevent common calculation errors.
- Practice with Different Problems: The more variety in problems you solve, the better you'll understand how to apply angular kinematics.
📊 Note: Consistent practice will refine your problem-solving abilities in angular kinematics.
5. Explore Digital Tools and Simulations

Modern technology offers tools that can significantly enhance your learning:
- Physics Simulations: Websites like PhET or Physics Classroom provide interactive simulations of angular motion.
- Apps for Kinematics: There are various apps designed to help you understand and practice kinematics problems.
- Virtual Labs: Many educational platforms offer virtual labs where you can experiment with angular motion principles without the need for physical equipment.
These tools allow you to experiment, test hypotheses, and observe the principles of angular kinematics in action, which can be much more engaging than static textbook learning.
As we've navigated through these tips for mastering angular kinematics, remember that each point builds upon the last. A solid foundation in the basics, paired with problem-solving practice and real-world applications, will significantly enhance your understanding. Angular kinematics isn't just about equations; it's about seeing the world in terms of rotation and understanding the beauty of motion in all its forms. Whether you're an engineer designing a better ride for a theme park or a student trying to ace your physics exam, these tips will serve as your guide to mastering the fascinating world of angular motion.
What are the primary units used in angular kinematics?

+
The primary units are radians or degrees for angular displacement, radians per second for angular velocity, and radians per second squared for angular acceleration.
How do linear and angular motion relate?

+
Linear velocity (v) is related to angular velocity (ω) by the equation v = rω, where r is the radius of the circular path. Similarly, linear acceleration relates to angular acceleration through a = rα.
Can you give an example of angular momentum conservation?
+
Yes, when an ice skater pulls their arms in while spinning, their moment of inertia decreases. To conserve angular momentum, their angular velocity must increase, causing them to spin faster.