7 Easy Steps to Write Inequalities from Word Problems

Understanding how to write inequalities from word problems can seem daunting at first, but once you master a few key concepts, it becomes much more manageable. This process is integral in mathematics, economics, and even daily life where we often face situations needing us to express or solve for unknown values within a certain range. Here, we'll guide you through 7 easy steps to translate word problems into mathematical inequalities effectively.
Step 1: Identify the Unknown Variable

The first step in solving any word problem involving inequalities is to identify what you're looking for. This could be:
- A quantity like "the number of apples John can buy."
- A condition like "the amount of time Sue has to get to work."
Let this unknown quantity be your variable. Represent it with letters like x , y , or any other suitable symbol.
Step 2: Understand the Relationship Between Variables

Next, you need to determine how different quantities in the problem relate to each other. Look for phrases like:
- "at least," which implies \geq
- "at most," indicating \leq
- "greater than," using >
- "less than," with <
These keywords will help you set up your inequality correctly.
Step 3: Convert the English Sentences into Mathematical Expressions

Translate each part of the word problem into an algebraic expression:
- "The price of a shirt is at most $30" translates to x \leq 30 .
- "Tom needs to save at least $500 for a trip" translates to y \geq 500 .
Step 4: Formulate the Inequality

Combine these expressions to form a single inequality:
- "Jack has $25 and wants to buy gifts for at least 5 friends with $5 each." This can be translated to 25 - 5x \geq 0 .
Step 5: Solve the Inequality (if necessary)

If your goal is to find the range of possible values for your variable, solve the inequality:
- Solving 25 - 5x \geq 0 gives x \leq 5 , meaning Jack can buy gifts for up to 5 friends.
Step 6: Check Your Solution

Always verify your solution to ensure it makes sense in the context of the original problem. Make sure your inequality provides a feasible solution:
- If Jack has only $25, he cannot buy gifts for 6 friends, hence the solution is correct.
Step 7: State the Result in Context

Finally, interpret your mathematical solution back into the context of the word problem:
- Jack can buy gifts for at most 5 friends given his $25 budget.
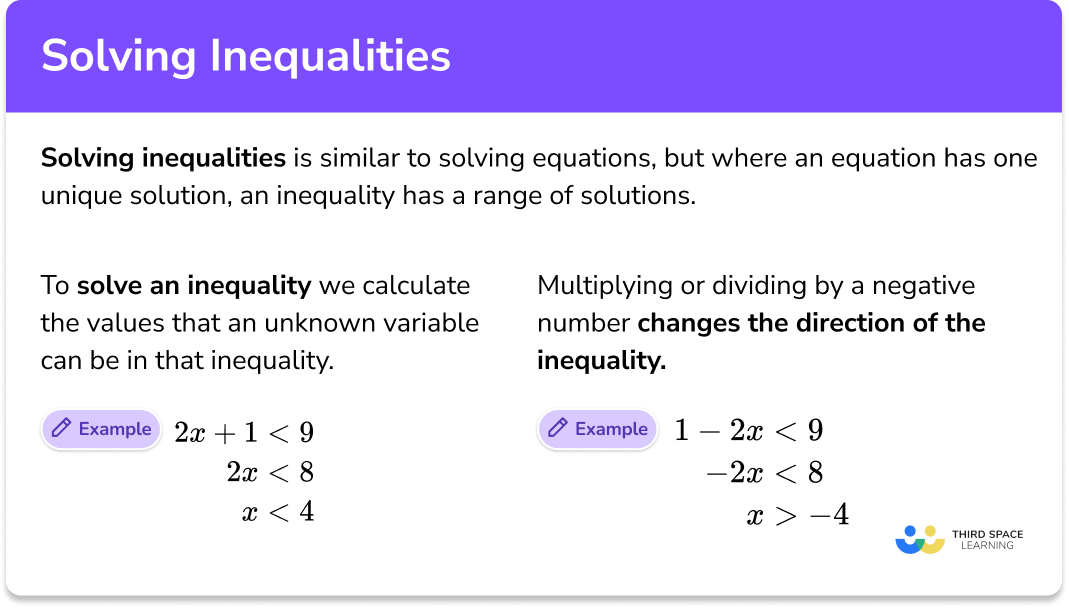
When dealing with inequalities, remember that:
📝 Note: The solution set of an inequality often includes a range of values, not just a single value, unlike equations.
In summary, by following these steps, you're not just solving problems mathematically; you're learning to think logically, translate natural language into mathematical terms, and understand the implications of your answers in real-world scenarios. Mathematics is not merely about numbers; it's about solving life's various puzzles through structured thought processes.
Why do we use inequalities instead of equations for some word problems?

+
Inequalities are used when we have conditions that specify ranges rather than exact values. They allow for multiple solutions which can reflect real-world scenarios where flexibility in outcomes is acceptable.
Can inequalities have multiple solutions?

+
Yes, inequalities can have an infinite number of solutions or a finite range, depending on the problem’s conditions. For example, ( x \geq 10 ) includes all numbers from 10 and upwards.
How do you represent inequalities with graph?
+
Inequalities can be graphed on a number line where a closed circle at the endpoint indicates “or equal to,” while an open circle means “less than” or “greater than” only.



