5 Tips for Writing Equations in Standard Form

Writing equations in standard form is a fundamental skill in algebra, allowing you to express relationships between variables clearly and consistently. Standard form for linear equations, which typically looks like Ax + By = C, has specific guidelines that ensure uniformity and facilitate easier manipulation and solving of equations. Here are some practical tips to master writing equations in standard form, enhancing your understanding of algebra and mathematical principles.
Understanding Standard Form

Before diving into the tips, let’s clarify what we mean by standard form for a linear equation:
- The coefficient of x should be positive, and if not, multiply or divide by a negative number to make it so.
- All terms must be moved to one side of the equation, with zero on the other side.
- Coefficients are usually written as integers with no common factor other than one (in simplest form).
- Variables are ordered with x first, then y, followed by the constant term on the right-hand side.
Tip #1: Identify Variable Terms and Constants

Start by clearly identifying which terms are variables (x, y) and which are constants. Here’s how to do that:
- List all variable terms on one side of the equation and constants on the other.
- Ensure all variable terms are combined and expressed in their simplest form.
- Make sure the equation has only one constant term on the right side.
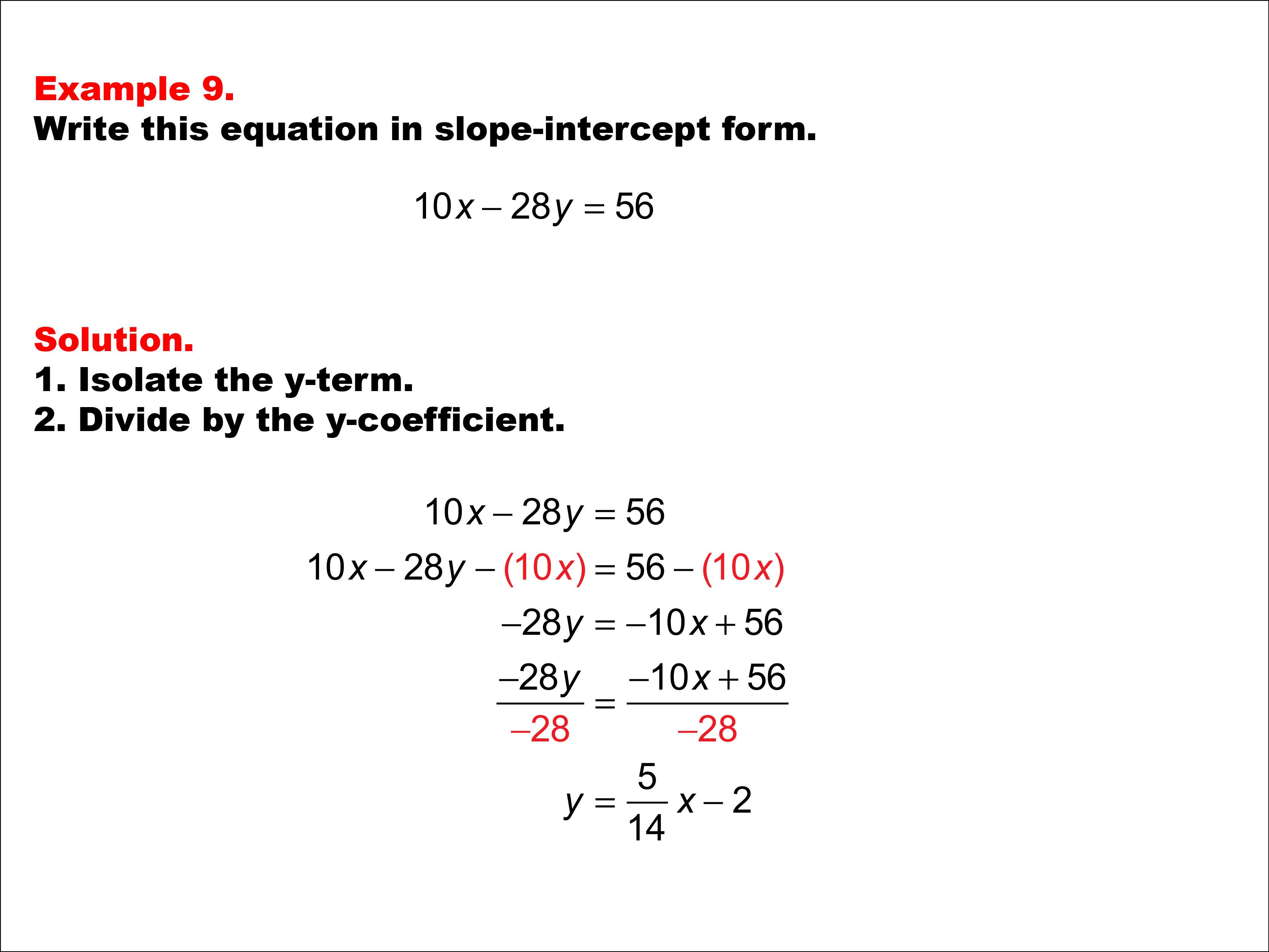
Tip #2: Rearrange the Equation
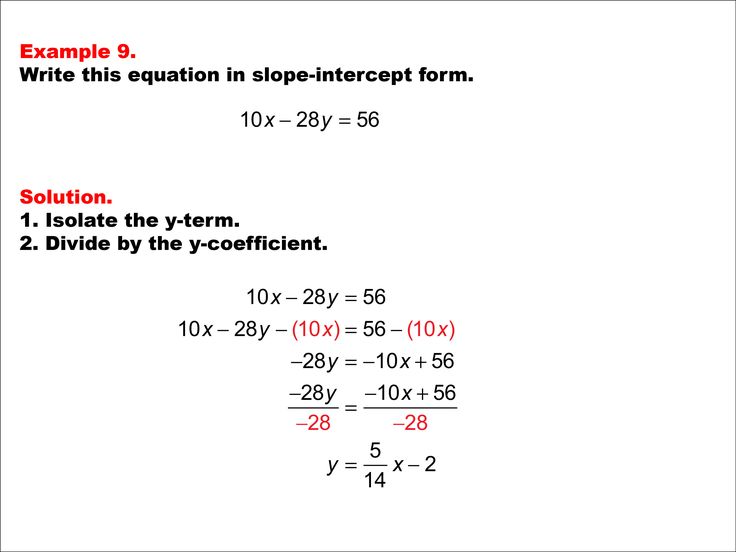
Rearranging terms is crucial for converting any equation into standard form:
- Move all variable terms to one side by subtracting or adding terms as necessary.
- Move the constant term to the opposite side of the equation, ensuring it remains positive.
- If the coefficient of x is negative, multiply every term by -1 to make it positive.
Tip #3: Simplify and Factor Out Integers

Equations in standard form look neat and are easier to solve when simplified:
- Reduce common factors from all terms to ensure no common divisor exists for the coefficients.
- Factor out any integer greater than 1 if possible, for clarity and ease of reading.
Tip #4: Order of Variables
The standard form dictates the order of variables:
- The x term should come first, followed by the y term, and then the constant term.
- If any term is missing, it is assumed to be zero or one as appropriate.
Tip #5: Double-Check Your Work

Verification is essential to ensure accuracy:
- Ensure the coefficient of x is positive.
- Check that all terms are combined on one side with the constant on the other.
- Confirm that the variables are in order and that there are no common factors in the coefficients.
To illustrate these tips, consider the following example:
| Step | Description |
|---|---|
| 1 | Original Equation: -2x + y = 6 |
| 2 | Rearrange to move x and y terms to the left: -2x + y - 6 = 0 |
| 3 | Make the coefficient of x positive by multiplying by -1: 2x - y + 6 = 0 |
| 4 | Ensure the constant is on the right-hand side: 2x - y = -6 |
🚨 Note: While standard form is the goal, some special cases might require deviations, such as when solving specific types of equations or systems of equations.
In conclusion, mastering the art of writing equations in standard form involves understanding the fundamental principles, rearranging terms, simplifying, ordering variables, and verifying your work. These techniques not only streamline your algebraic processes but also enhance your comprehension of mathematical relationships and facilitate easier problem-solving and graphing in future mathematical explorations.
Why is standard form important in algebra?
+
Standard form ensures uniformity in equation presentation, making it easier to solve systems of equations, graph, and understand the relationship between variables.
Can equations always be written in standard form?

+
Most linear equations can be written in standard form, but some non-linear or special forms might not conform strictly to standard form conventions.
What if I have more than two variables in my equation?
+
Extend the same principles by ordering variables from x, y, z, and so forth, ensuring the coefficient of the first variable is positive.



