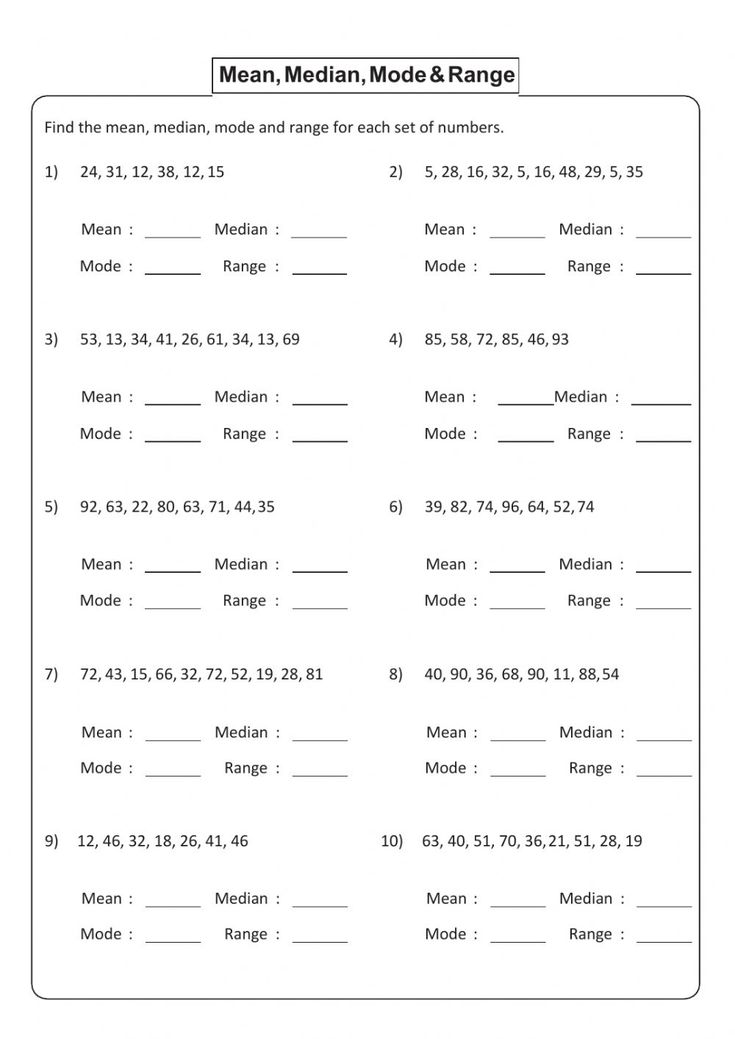
Color the Numbers: Mean, Median, Mode, Range Worksheet

Delving into the world of statistics and data analysis can initially appear complex, but it becomes manageable once we grasp some fundamental concepts such as mean, median, mode, and range. These terms describe how data can be summarized, compared, and analyzed, providing valuable insights into any dataset. This blog post aims to demystify these concepts, offering an educational journey through real-life examples, visual aids, and practical worksheets to make learning statistics an engaging and interactive experience.
Understanding Mean

The mean, commonly known as the average, is one of the most basic yet crucial statistical measures. It is calculated by summing all the numbers in a dataset and then dividing by the count of those numbers.
📊 Note: The mean is very sensitive to extreme values, which can skew the result significantly.
How to Calculate Mean

- Add up all the values in the dataset.
- Count how many values there are.
- Divide the total by the count.
| Day | Temperature (°C) |
|---|---|
| Monday | 12 |
| Tuesday | 15 |
| Wednesday | 18 |
| Thursday | 20 |
| Friday | 17 |
| Mean Temperature | 16.4 |

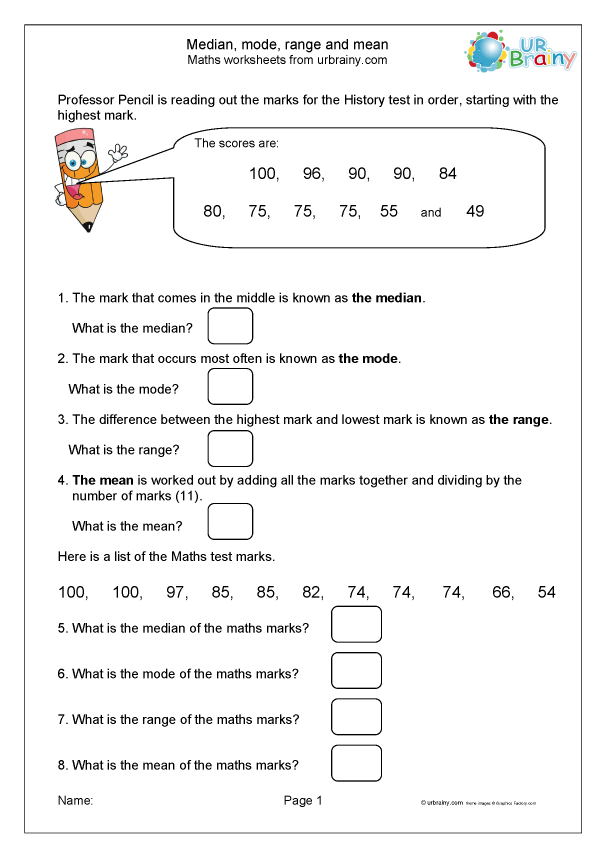
Unraveling Median

While the mean represents the typical value, the median highlights the middle value when data points are arranged in ascending or descending order. This measure is less sensitive to outliers and provides a different perspective on the central tendency.
How to Find the Median
- List the numbers in order from smallest to largest.
- If there is an odd number of observations, the median is the middle number.
- If there is an even number, the median is the average of the two middle numbers.
| Data Point | Value |
|---|---|
| Lowest | 10 |
| Second Lowest | 12 |
| Middle (Median) | 15 |
| Second Highest | 18 |
| Highest | 20 |
Exploring Mode

The mode of a dataset is the value that appears most frequently. In some cases, there might be more than one mode (multimodal) or no mode at all.
Finding the Mode

- List all unique values.
- Count the frequency of each value.
- The value with the highest frequency is the mode.
| Color | Frequency |
|---|---|
| Red | 3 |
| Blue | 2 |
| Green | 1 |
| Yellow | 3 |
| Mode(s) | Red, Yellow |
Calculating Range

Range provides a measure of dispersion or spread, indicating the difference between the highest and lowest values in the dataset.
To Determine the Range

- Identify the largest value in your dataset.
- Identify the smallest value in your dataset.
- Subtract the smallest value from the largest to get the range.
| Data Value | Amount |
|---|---|
| Smallest Value | 3 |
| Other Values | 5, 6, 7, 8, 9, 10, 11 |
| Largest Value | 15 |
| Range | 12 |
In wrapping up our exploration, we've seen how mean, median, mode, and range serve as tools to understand, describe, and analyze data. These statistical measures each give us a unique lens through which to view our data, allowing for better decision-making and deeper insights. By using these concepts effectively, we're better equipped to manage variability, make predictions, and critically assess the data that surrounds us every day.
What if I have two modes in my dataset?

+
If there are multiple values with the same highest frequency, your dataset is multimodal. Both of these values are considered the mode of the data set.
Why does the mean not always represent the typical value?

+
The mean can be significantly influenced by outliers or extreme values, making it not always representative of the central tendency if there are such anomalies.
Can the median be used instead of the mean when the data has outliers?
+
Yes, since the median is less affected by extreme values, it’s often a better measure of central tendency when dealing with skewed data or outliers.