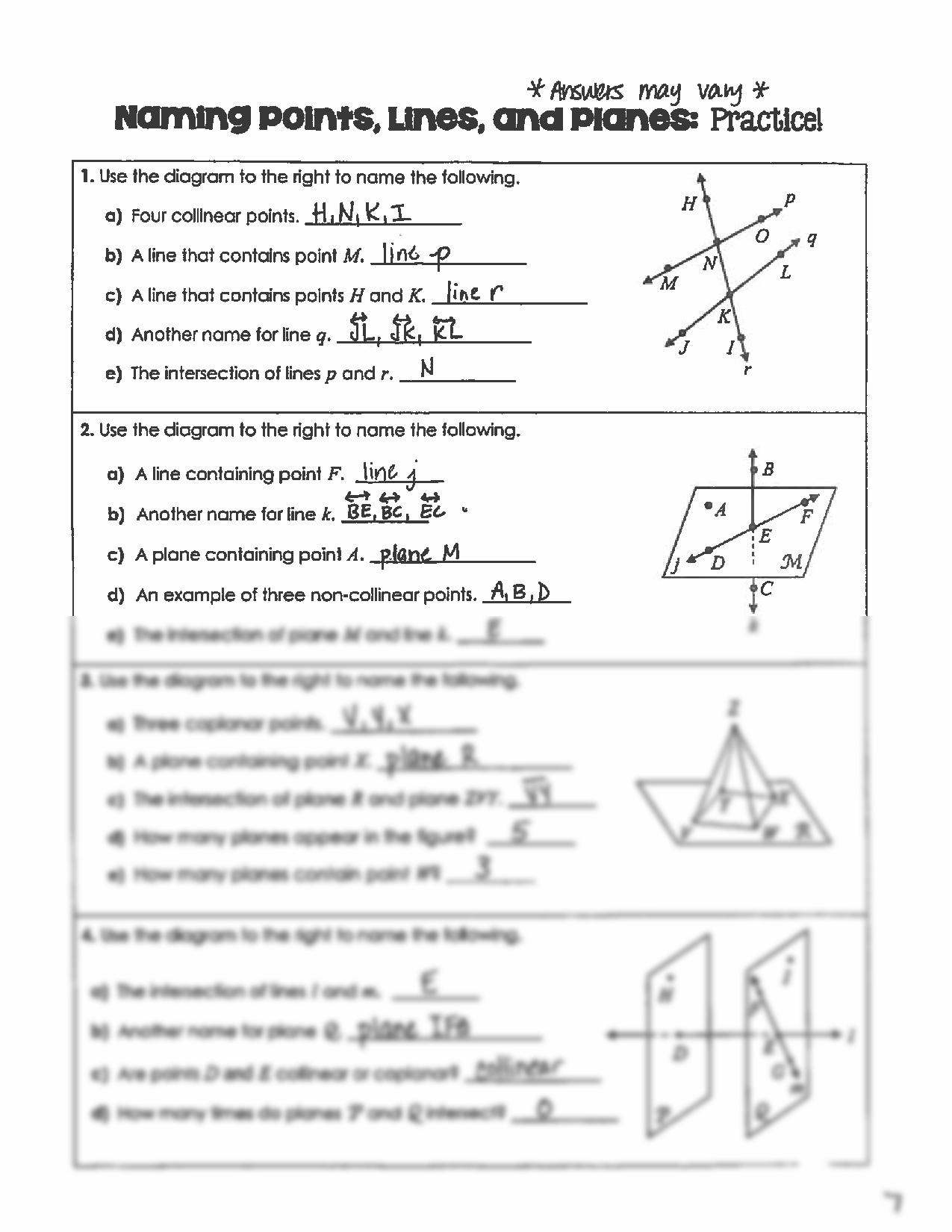
5 Ways to Master Angles in Parallel Lines

In the fascinating world of geometry, the understanding of angles in parallel lines is not just essential; it's a cornerstone for a myriad of applications in mathematics and beyond. This blog post will guide you through five proven techniques to enhance your grasp on these fundamental geometric concepts. Whether you're a student aiming for proficiency in high school geometry or someone interested in design, architecture, or any field where precision matters, these strategies are designed to provide you with a solid foundation.
Understanding the Basics

Before diving into the mastery techniques, let’s quickly cover the basic terms and properties of angles between parallel lines:
- Parallel Lines: Two lines that never intersect, irrespective of their length.
- Transversal: A line that intersects two or more parallel lines at distinct points.
- Corresponding Angles: Angles on the same side of the transversal and at the same position relative to the lines.
- Alternate Interior Angles: Angles inside the parallel lines but on opposite sides of the transversal.
- Alternate Exterior Angles: Angles outside the parallel lines but on opposite sides of the transversal.
- Co-Interior or Same-Side Interior Angles: Angles inside the parallel lines and on the same side of the transversal.
1. Visual Aids and Interactive Tools
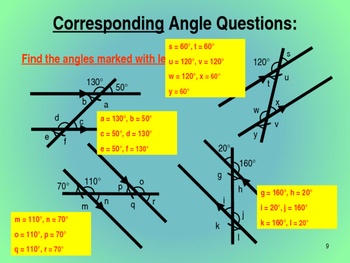
Geometry can be abstract, but visual aids like diagrams, angles rulers, and digital tools can make it tangible:
- Use Diagrams: Drawing diagrams helps in visualizing the relationships between angles. Label your diagrams carefully to keep track of which angles are corresponding, alternate, etc.
- Interactive Geometers: Utilize free online tools like GeoGebra to experiment with angles. You can see how changing one angle affects others, providing a dynamic learning experience.

📝 Note: Always ensure the diagram is drawn to scale to avoid confusion during calculations.
2. Formula Memorization and Practice

Remembering and understanding the following formulas can greatly aid in mastering angles:
| Angle Type | Relationship | Formula |
|---|---|---|
| Corresponding Angles | Equal | ∠1 = ∠5, ∠2 = ∠6, etc. |
| Alternate Interior Angles | Equal | ∠3 = ∠5, ∠4 = ∠6 |
| Alternate Exterior Angles | Equal | ∠1 = ∠7, ∠2 = ∠8 |
| Co-Interior Angles | Supplementary | ∠3 + ∠5 = 180°, ∠4 + ∠6 = 180° |

- Memorize the formulas for quick recognition.
- Practice using these formulas with a variety of angles problems to build familiarity and speed.
3. Proofs and Logical Reasoning

Mastery of angles requires not just knowing the relationships but also understanding why they hold true:
- Understand proofs: Learn to construct proofs for parallel lines cut by a transversal. Understanding the steps in geometric proof helps solidify your knowledge.
- Logical Reasoning: Practice deducing the measures of unknown angles from given information.
4. Real-World Applications

Geometry’s applicability in real-world scenarios can help reinforce your understanding:
- Architecture: Look at how architects use angles when designing buildings. Understanding how to construct parallel lines helps in creating blueprints.
- Engineering: Angles play a critical role in engineering design, especially in mechanics and civil engineering, where understanding forces and beams is essential.
- Art and Design: Artists and designers use angles to create balance, perspective, and visual appeal.

🌉 Note: Bridges often use parallel lines and angles to distribute weight evenly, which is an excellent real-world example of angles in parallel lines.
5. Continuous Learning and Practice

Geometry, like any other discipline, requires constant engagement:
- Quizzes and Tests: Regularly test your knowledge with quizzes or by attempting past exam questions.
- Geometry Community: Engage with online communities or forums to discuss and solve problems together.
By keeping a steady pace of learning and practicing, you ensure that your understanding of angles in parallel lines remains robust and applicable in various scenarios.
Through these five steps, you've laid the groundwork to master angles in parallel lines. This knowledge opens up not just academic avenues but also practical applications in numerous fields. Understanding these basic geometric principles not only boosts your confidence in solving problems but also enhances your ability to reason and apply logical thinking. Remember, practice is the key to proficiency. Keep experimenting, keep learning, and you'll find yourself effortlessly navigating through the complex world of angles and lines.
What are corresponding angles?

+
Corresponding angles are pairs of angles that occupy the same relative position at each intersection where a transversal line cuts across two parallel lines. They are congruent.
How do you identify alternate interior angles?

+
Alternate interior angles are those formed on the inside of two parallel lines when intersected by a transversal, but they lie on opposite sides of the transversal. They are also congruent.
Can angles be used in real-life applications?

+
Absolutely! Angles are integral to numerous fields including architecture, engineering, art, and even navigation. Understanding angles helps in creating balanced designs, optimizing structures, and ensuring accuracy in various measurements.
Why is memorizing formulas important?

+
Memorizing formulas allows for quick problem-solving and understanding of the underlying geometric principles. It enables you to perform calculations efficiently and focus more on the conceptual application rather than the arithmetic.