5 Tips to Solve Variables on Both Sides Equations
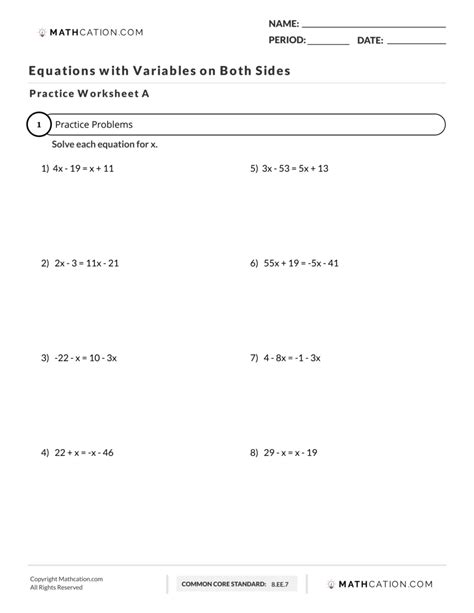
What are Equations with Variables on Both Sides?
Equations with variables on both sides are a type of linear equation where the variable (usually x) appears on both the left and right sides of the equation. These equations can be challenging to solve, but with the right strategies, you can simplify and solve them with ease.
Tips to Solve Equations with Variables on Both Sides

Here are five tips to help you solve equations with variables on both sides:
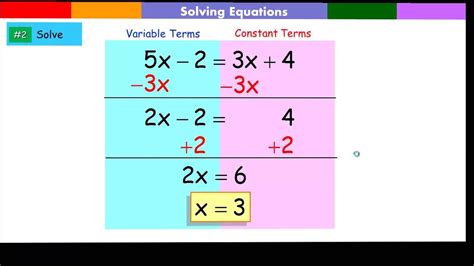
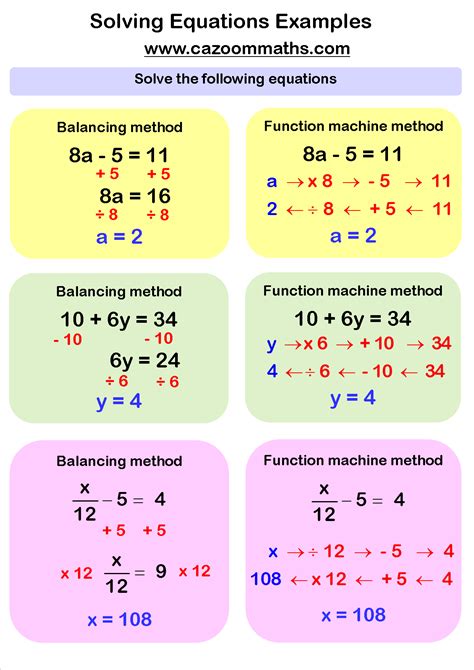
1. Simplify the Equation

Before attempting to solve the equation, simplify it by combining like terms on both sides. This will make it easier to work with the equation.
Example:
2x + 3 = x + 5
Combine like terms:
x + 3 = 5
2. Move the Variable to One Side

To solve for the variable, try to move it to one side of the equation by adding, subtracting, multiplying, or dividing both sides of the equation.
Example:
x + 3 = 5
Subtract 3 from both sides:
x = 2
3. Eliminate the Coefficient

If the variable has a coefficient (a number in front of it), eliminate it by dividing both sides of the equation by the coefficient.
Example:
2x = 6
Divide both sides by 2:
x = 3
4. Use Inverse Operations
Inverse operations are operations that “undo” each other, such as addition and subtraction, multiplication and division. Use inverse operations to isolate the variable.
Example:
x + 2 = 7
Subtract 2 from both sides (inverse operation of addition):
x = 5
5. Check Your Solution

Finally, check your solution by plugging it back into the original equation. This ensures that your solution is correct.
Example:
x + 2 = 7
x = 5
Plug x = 5 back into the original equation:
5 + 2 = 7
7 = 7
True!
👍 Note: Make sure to follow the order of operations (PEMDAS) when solving equations with variables on both sides.
Common Mistakes to Avoid
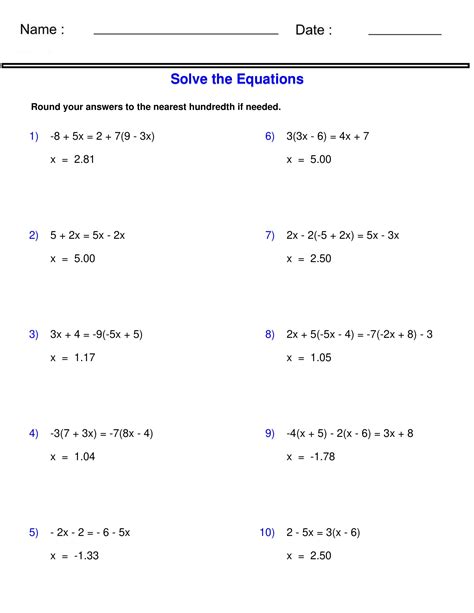
When solving equations with variables on both sides, be careful not to make the following common mistakes:
- Adding or subtracting the same value to both sides of the equation without canceling it out
- Forgetting to distribute the coefficient to all terms on one side of the equation
- Not checking the solution by plugging it back into the original equation
Practice Exercises
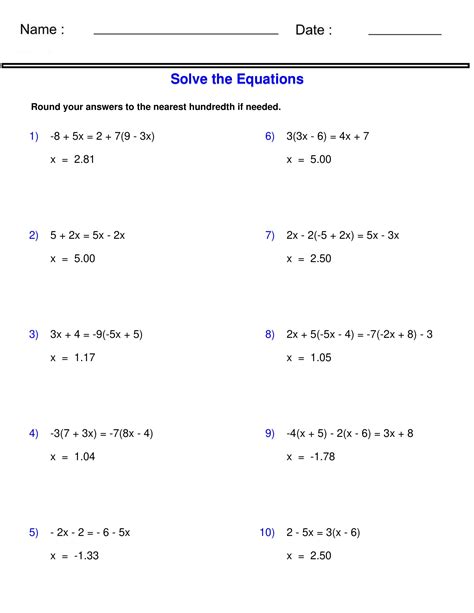
Try solving the following equations with variables on both sides:
- 2x + 5 = x + 11
- x - 3 = 2x + 1
- 4x = 2x + 12
Check your solutions by plugging them back into the original equations.
Table of Solutions

| Equation | Solution |
|---|---|
| 2x + 5 = x + 11 | x = 6 |
| x - 3 = 2x + 1 | x = -2 |
| 4x = 2x + 12 | x = 6 |

What is the purpose of simplifying an equation with variables on both sides?

+
Simplifying an equation with variables on both sides makes it easier to work with the equation and solve for the variable.
How do I eliminate the coefficient in an equation with variables on both sides?
+
To eliminate the coefficient, divide both sides of the equation by the coefficient.
Why is it important to check my solution by plugging it back into the original equation?
+
Checking your solution by plugging it back into the original equation ensures that your solution is correct and accurate.
By following these five tips and avoiding common mistakes, you’ll become a pro at solving equations with variables on both sides!
Related Terms:
- equations on both sides pdf
- equations with two variables worksheet
- solving equations with variables worksheet
- variables on both sides pdf
- algebraic equations worksheet pdf