Master Variables on Both Sides with Our Worksheet
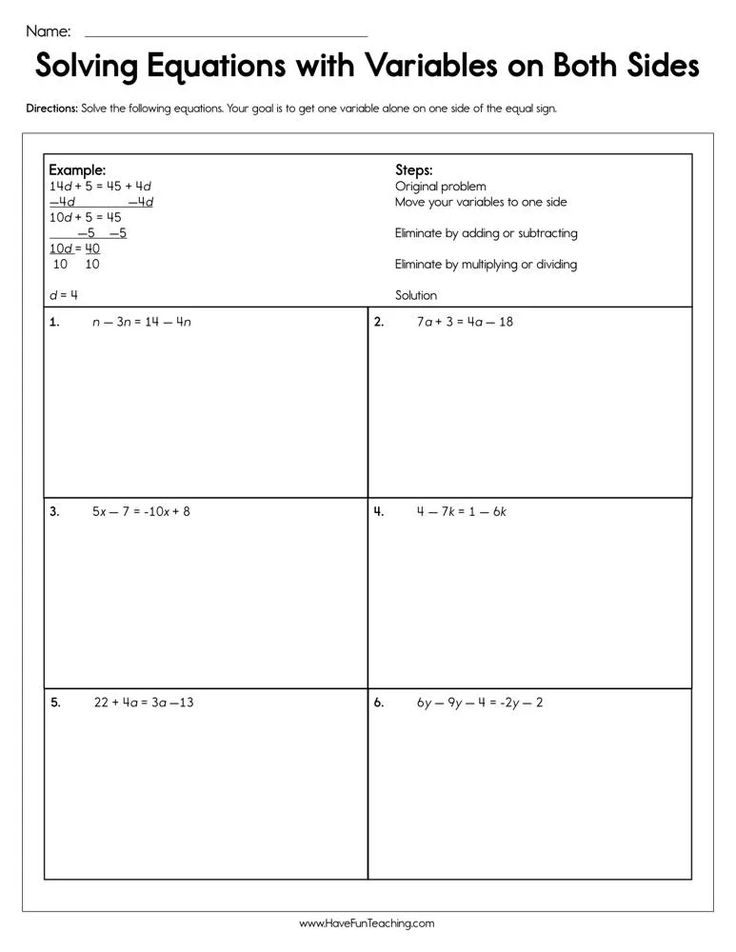
In the vast landscape of algebra, one of the key skills students must master is solving equations with variables on both sides. This skill is not just a mathematical challenge; it's a foundational step that enhances problem-solving abilities in numerous areas. Whether you're gearing up for an exam or just trying to understand the mechanics behind these equations, mastering variables on both sides is crucial. This blog post will delve into the intricacies of solving equations where variables are present on both sides, explore the importance of this skill, and provide a structured approach through our specialized worksheet.
The Importance of Solving Equations with Variables on Both Sides

Algebra, often seen as the gateway to higher mathematics, relies heavily on understanding how to manipulate equations. Here’s why solving equations with variables on both sides is vital:
- Higher-Level Mathematics: Mastery of these equations is necessary for subjects like calculus where you’ll encounter more complex forms of these equations.
- Problem Solving: This skill teaches logical thinking and step-by-step problem-solving, which is applicable in science, engineering, and various other fields.
- Real-Life Applications: Many real-world problems can be formulated as equations with variables on both sides, from financial planning to physical laws.
The Basics of Equations with Variables on Both Sides

An equation of the form ax + b = cx + d where a and c are coefficients of the variable x, and b and d are constants, exemplifies an equation with variables on both sides. Here are the foundational steps to solve such equations:
- Isolate the Variables: Add or subtract to get all variables on one side.
- Combine Like Terms: Simplify by combining constants and variables.
- Solve for the Variable: Use inverse operations to isolate x.
- Check Your Solution: Substitute your solution back into the original equation to ensure accuracy.
⚠️ Note: Always double-check your work when solving equations, as one small mistake can lead to an incorrect solution.
Introducing the Variables on Both Sides Worksheet

Our worksheet is designed to help students practice and master this concept. Here’s what it includes:
- A step-by-step guide for solving equations with variables on both sides.
- Worked examples to illustrate the method.
- Practice problems with increasing difficulty to build confidence.
- Answer key for immediate feedback.
Step-by-Step Guide Through the Worksheet

Let’s walk through the process with some examples from our worksheet:
Example 1: Simple Equation

Solve the equation 2x + 6 = x + 10:
- Subtract x from both sides to isolate the variable: 2x - x + 6 = 10, which simplifies to x + 6 = 10.
- Subtract 6 from both sides: x = 4.
- Check: 2(4) + 6 = 4 + 10, which is 14 = 14.
Example 2: Equation with Decimals

Solve 0.2x + 4 = 0.6x - 2:
- Subtract 0.6x from both sides: 0.2x - 0.6x + 4 = -2, which simplifies to -0.4x + 4 = -2.
- Subtract 4 from both sides: -0.4x = -6.
- Divide by -0.4: x = 15.
- Check: 0.2(15) + 4 = 0.6(15) - 2, which simplifies to 7 = 7.
| Step | Operation | Result |
|---|---|---|
| 1 | Subtract 0.6x | -0.4x + 4 = -2 |
| 2 | Subtract 4 | -0.4x = -6 |
| 3 | Divide by -0.4 | x = 15 |
| 4 | Check | 7 = 7 |

Common Mistakes and How to Avoid Them

Even with a good grasp of the process, some common pitfalls can trip students up:
- Neglecting to Combine Like Terms: Ensure you combine terms properly after moving variables or constants across the equal sign.
- Dividing by Zero: When solving, make sure you don’t inadvertently divide both sides by zero, which is undefined.
- Misidentifying Like Terms: Carefully identify which terms are like before combining.
- Skipping the Check Step: Always verify your solution.
🔍 Note: Mistakes in math are part of the learning process; they often highlight areas that need more attention or a different approach.
Why Practice is Essential

Practice is the key to mastering equations with variables on both sides because:
- It reinforces the steps and logic behind solving these equations.
- Repetition increases speed and accuracy, crucial for timed assessments.
- Exposure to varied problems helps students adapt to different equation structures.
Final Thoughts

By now, you’ve seen that solving equations where variables appear on both sides is not just about the mechanics of moving terms around. It’s about cultivating a deep understanding of the relationships between numbers and symbols, honing your critical thinking, and preparing for more advanced mathematical explorations. Whether for academic success or to enhance everyday problem-solving skills, mastering these equations is invaluable. Our worksheet is a stepping stone in this journey, offering a comprehensive approach to practice, learn, and excel in algebra. Remember, the more you practice, the more intuitive these solutions become, transforming complex equations into straightforward puzzles to solve.
Why are equations with variables on both sides important?

+
These equations are crucial for developing problem-solving skills, understanding higher-level mathematics, and preparing for practical applications in fields like science, finance, and engineering.
How can I practice solving equations with variables on both sides?

+
Use our worksheet, engage in textbook exercises, and consider online platforms or tutors for additional practice. Regular, varied practice is key.
What’s the most common mistake when solving these equations?

+
One of the most common errors is not combining like terms correctly, or forgetting to check the solution after solving.



