Master Integers Easily: Free Worksheet for Clear Understanding

Understanding integers is essential for various mathematical operations, from simple arithmetic to more complex problems like number theory. For many learners, grappling with the concept of negative numbers can be a challenge. This post will offer you a step-by-step guide on mastering integers, particularly through the use of a free worksheet designed to enhance your understanding and ensure clarity in this fundamental area of math.
Introduction to Integers


Let's begin with the basics:
- Integers include all whole numbers, both positive and negative, along with zero.
- The set of integers is often represented as ℤ.
- They can be thought of as points on a number line, extending indefinitely in both directions.
Why Learning Integers is Important
- Foundation for Advanced Math: Integers lay the groundwork for algebra, calculus, and other advanced mathematical fields.
- Real-world Applications: Integers are used in finance, accounting, temperature readings, and many other areas where both positive and negative values are needed.
- Developing Logical Skills: Understanding the operations with integers helps in building logical reasoning abilities.
Exploring the Integer Number Line


To visualize integers:
- Draw a horizontal line, with zero at the center.
- Positive numbers are marked to the right of zero, while negative numbers are placed to the left.
- Each increment represents one unit on this number line.
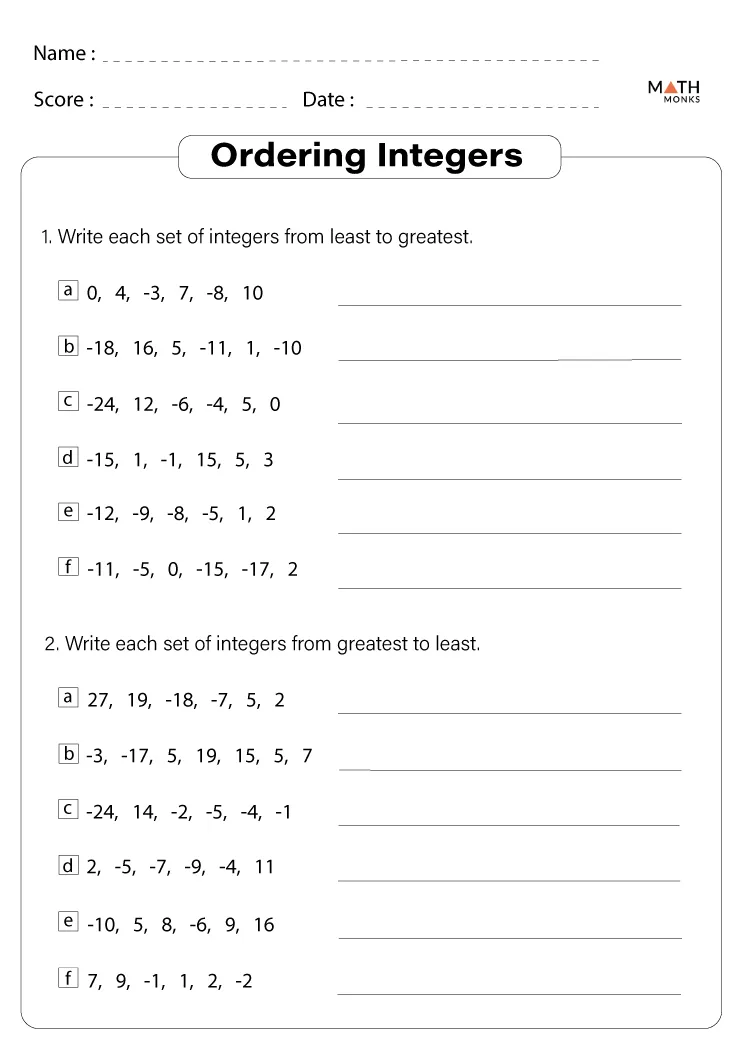
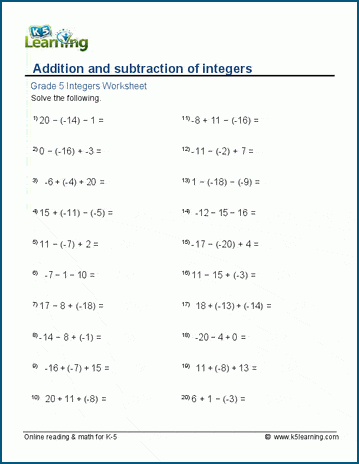
Free Worksheet on Integers

Below is a sample of our free worksheet designed to help you practice and understand integers better:
| Question Type | Example |
|---|---|
| Basic Addition & Subtraction | Solve: -5 + 3 = |
| Comparing Integers | Which is smaller: -7 or -3? |
| Ordering Integers | Arrange in ascending order: 4, -2, 0, -5 |
| Multiplication & Division | Calculate: (-2) * 6 = |
| Real-life Application Problems | Jane’s account has a balance of -$20. She deposits $50. What is her new balance? |

Explanations and Tips for Each Section

Basic Addition & Subtraction

🌟 Note: When adding a positive and a negative integer, subtract the smaller absolute value from the larger and keep the sign of the integer with the greater absolute value.
- To add integers with different signs, think about moving along the number line. Adding a negative number is like subtracting.
- For subtraction, change the sign of the integer being subtracted and then add them together.
Comparing Integers
- Compare their absolute values if integers have different signs. The negative integer with the larger absolute value is smaller.
- If both are negative, the one with the smaller absolute value is greater.
Ordering Integers

- Sort positive integers from smallest to largest.
- Sort negative integers from largest to smallest, as they are the opposite of positive numbers.
- Zero is considered neutral and always lies in the middle.
Multiplication & Division

🔍 Note: When multiplying or dividing two integers, the result is positive if both numbers have the same sign, and negative if the signs are different.
- Remember, the product or quotient of two negatives is positive.
- The sign of the result is determined by the signs of the integers involved.
Real-life Application Problems

- Consider integers as representing both debts and credits, temperatures, gains and losses, etc.
- Translating word problems into mathematical equations using integers helps in visualizing the problem.
Incorporating this free worksheet into your study routine can significantly improve your mastery over integers. With practice and understanding of the underlying principles, you'll find yourself navigating these numbers with ease. Whether you're a student looking to strengthen your mathematical foundation or an adult brushing up on math skills, the ability to understand and work with integers is invaluable.
Recalling key points, we've explored what integers are, why they're important, how to visualize them on a number line, and have provided a structured approach to learning through our free worksheet. Each section focuses on essential aspects of integer operations, offering both practical examples and theoretical underpinnings. In essence, this post has aimed to demystify integers, making them less abstract and more applicable to real-world scenarios.
What is the difference between an integer and a whole number?

+
A whole number includes all natural numbers (1, 2, 3, …) along with zero (0), but excludes negative numbers. Integers, on the other hand, encompass both whole numbers and negative numbers.
How can I quickly determine if an integer operation will result in a positive or negative number?

+
For addition, if the sum of two integers has the same sign as both numbers, the result is positive. If the signs differ, the sign of the integer with the larger absolute value determines the result’s sign. For multiplication and division, if the signs are the same, the result is positive; if they differ, the result is negative.
Is zero considered a positive or negative integer?

+
Zero is neither positive nor negative; it’s the neutral point in the number line, dividing positive from negative integers.