Master Two-Step Equations: Fun 7th Grade Worksheet

Understanding the Basics of Two-Step Equations

Mathematics, in its beauty and complexity, constantly challenges us to find solutions to equations that not only test our basic algebraic skills but also our patience and creativity in problem-solving. For 7th graders, mastering two-step equations is a fundamental step towards delving deeper into algebra. Let's break down this concept, so you can excel in solving these equations with ease.
What are Two-Step Equations?

Before diving into the steps, let's define what we mean by two-step equations. A two-step equation involves two operations to isolate the variable (usually x). These operations typically include addition/subtraction and multiplication/division. The goal is to manipulate the equation so that the variable stands alone on one side of the equation.
Key Steps to Solve Two-Step Equations

The process of solving two-step equations follows a straightforward procedure. Here are the steps you need to master:
- Undo Addition/Subtraction: If there's an operation that involves addition or subtraction on the side with the variable, we undo it first. This moves the constant term to the other side of the equation.
- Undo Multiplication/Division: Next, we deal with operations involving multiplication or division. This isolates the variable.
Step-by-Step Example:

Let's take an example equation: 2x + 3 = 11. Here's how you solve it:
- First, we undo the addition of 3 by subtracting 3 from both sides of the equation:
2x + 3 - 3 = 11 - 3
This simplifies to 2x = 8.
- Next, we need to isolate x by undoing the multiplication by 2. We divide both sides by 2:
(2x) / 2 = 8 / 2
This gives us x = 4.
By following these steps systematically, you can solve any two-step equation accurately.
Fun and Engaging Worksheets
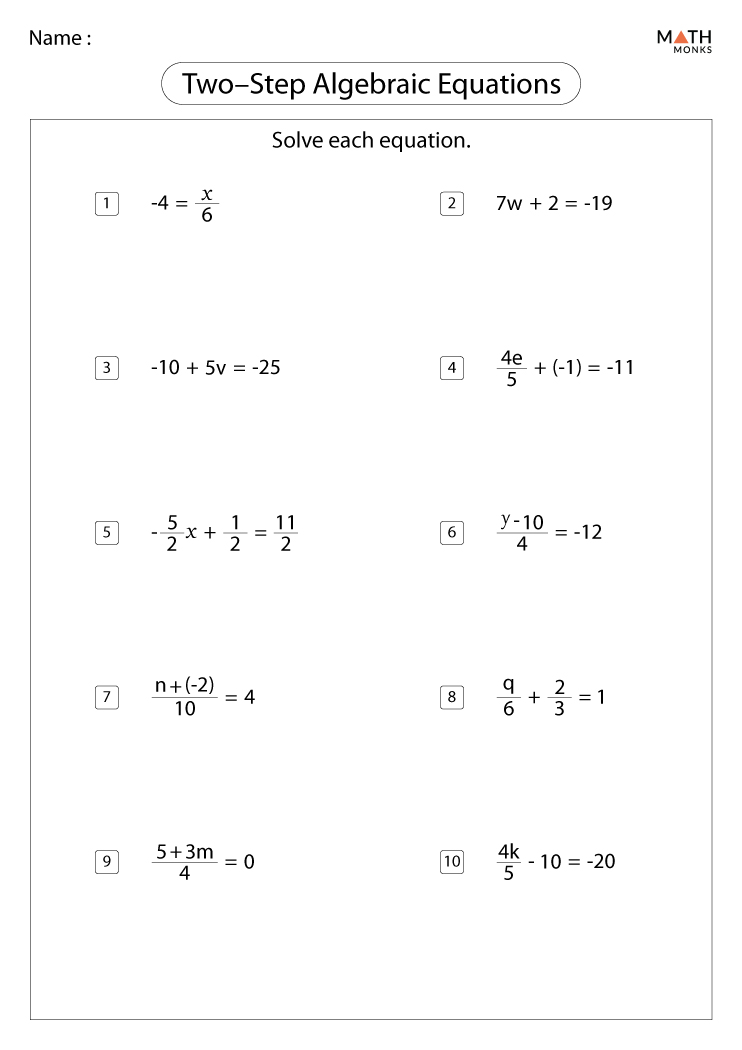
Practicing with worksheets can make mastering two-step equations more engaging. Here's a structured worksheet idea:
| Problem | Answer |
|---|---|
| 4x - 2 = 10 | x = 3 |
| -3x + 7 = 13 | x = -2 |
| 5x / 3 + 6 = 16 | x = 6 |
| 12 - x/4 = 3 | x = 36 |
This worksheet can be expanded or adapted to include more complex or variable types of problems, incorporating elements like negative coefficients or fractions to challenge students further.
Making Math Fun with Word Problems

To engage students further, word problems can be an excellent tool. Here are some examples:
- Maria had 14 shells more than twice the number of shells Juan had. She has 18 shells. How many shells does Juan have? (Answer: Juan has 7 shells)
- There are three times as many apples as oranges in a fruit basket. If the total number of fruits is 16, how many of each type of fruit are in the basket? (Answer: There are 4 oranges and 12 apples)
📝 Note: Always ensure you understand the problem before writing out the equation. This helps in translating real-life scenarios into mathematical expressions correctly.
Checking Your Work

After solving an equation, it's a good habit to check your work. Plug the solution back into the original equation:
- Using the equation 2x + 3 = 11, if x = 4:
Left side = 2(4) + 3 = 8 + 3 = 11.
Right side = 11
Both sides are equal, so our solution is correct.
Tips for Tackling More Complex Two-Step Equations

As equations become more complex, here are some strategies to keep in mind:
- Factorization: Sometimes equations might require factoring before solving.
- Negative Coefficients: Pay attention to signs. Dividing by a negative number changes the direction of the inequality.
- Parentheses: If an equation includes parentheses, simplify what's inside first.
Remember, practice is key. The more you solve, the more intuitive these steps become.
So, as you've journeyed through the land of two-step equations, remember that each problem solved is a step closer to algebraic mastery. Your understanding of variables, operations, and the order of operations grows with each worksheet completed. Keep practicing with fun problems and real-life scenarios, check your work diligently, and you'll find that two-step equations become not just manageable, but enjoyable.
Why do we perform operations on both sides of an equation?

+
Performing operations on both sides of an equation maintains the equality between the two sides, which is fundamental to solving for the unknown variable.
How can I make learning two-step equations fun?
+
Incorporate real-life word problems, group work, games like “equation bingo”, or competitive challenges to engage students in learning.
What if I get stuck on a particularly difficult equation?

+
Don’t give up. Try breaking down the problem into smaller parts, look for similar solved examples, or ask for help from teachers, classmates, or online resources.