5 Essential Tips for Solving Trig Equations Easily

Are you grappling with trigonometric equations? Trigonometry, with its diverse array of identities and functions, can appear daunting. However, with the right tips and tricks, solving these equations can become much simpler. Here's an in-depth guide to master these equations with ease:
1. Familiarize Yourself with Trig Identities
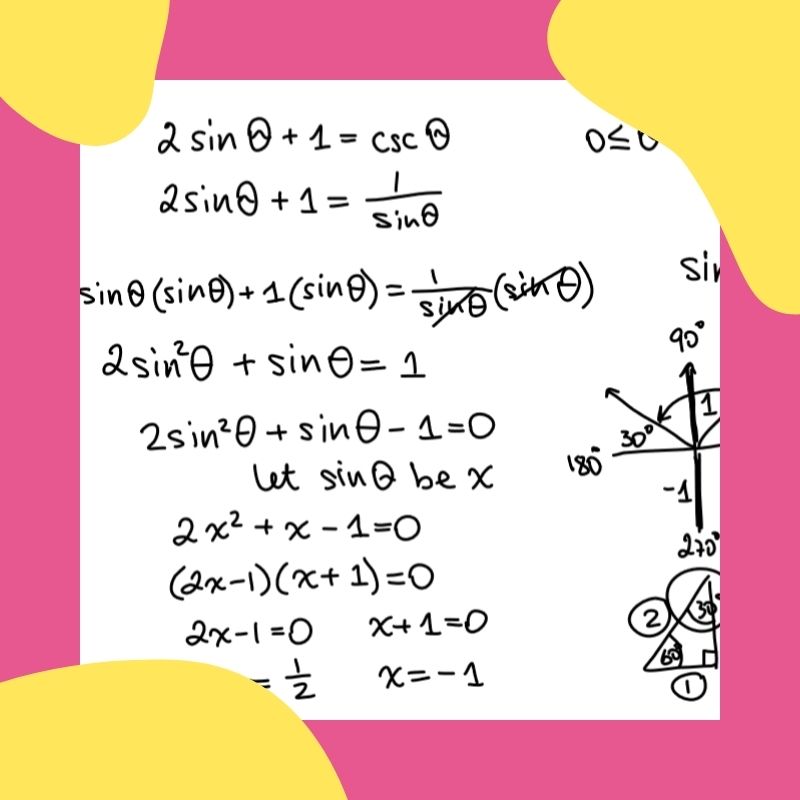

Trigonometric identities are essential tools for solving trigonometric equations. Here are some fundamental identities you should be comfortable with:
- Pythagorean Identities:
(\sin^2\theta + \cos^2\theta = 1) - Sum and Difference Identities:
(\sin(a \pm b) = \sin a \cos b \pm \cos a \sin b) - Double-Angle Identities:
(\sin 2\theta = 2 \sin \theta \cos \theta) - Half-Angle Identities:
(\sin \frac{\theta}{2} = \pm \sqrt{\frac{1 - \cos \theta}{2}})
Mastering these identities will allow you to simplify complex trigonometric equations into more manageable forms. Regular practice and memorization can significantly enhance your ability to manipulate these equations effectively.
💡 Note: Spend time regularly practicing these identities. A good exercise is to derive these identities from the basic definitions of sine and cosine.
2. Graph Trigonometric Functions


Understanding the graphical representation of trigonometric functions can provide intuitive solutions:
- Sine, cosine, and tangent have predictable wave-like patterns. Identifying where these functions cross specific values on the unit circle can help solve equations.
- Graphs allow you to visualize where the equations intersect the x-axis, which directly translates to the solutions for (y = 0).
- Use graphing tools or sketch by hand to see how changing parameters affects the function’s behavior.
Graphing not only helps in understanding trigonometric functions visually but also in solving them more efficiently. It's beneficial for both conceptual understanding and practical problem-solving.
3. Solve Using Unit Circle
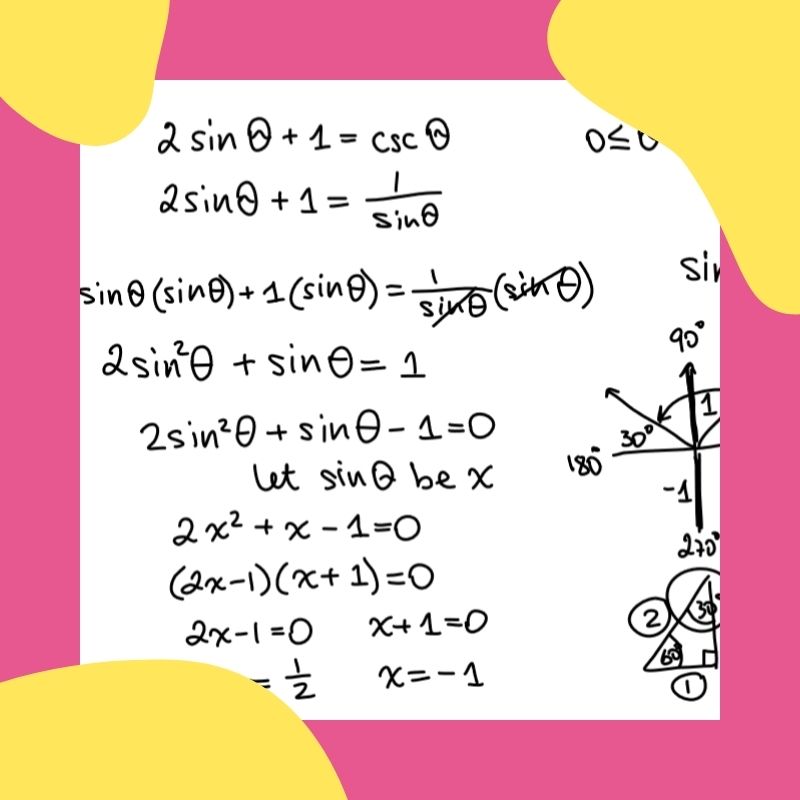

The unit circle is an invaluable tool for solving trigonometric equations:
- Understand key points on the circle where sine and cosine are known values (like 0°, 30°, 45°, 60°, and 90°).
- For any given trigonometric value, determine which angles could produce that value within one period. Then extend these solutions to all periods.
- Always consider both positive and negative values as trigonometric functions can repeat in different quadrants.
By mastering the unit circle, you'll find that solving equations for sin, cos, or tan becomes much more intuitive and quicker.
🔍 Note: Using reference angles will help you identify solutions in different quadrants, which can be crucial for finding all possible solutions.
4. Use Trigonometric Substitution

Trigonometric substitution involves replacing parts of your equation with trigonometric functions:
- Substitute expressions like (a^2 - x^2) with (\cos \theta) or (x^2 - a^2) with (-\sin \theta), or (\sqrt{a^2 + x^2}) with (\sec \theta).
- This technique can simplify square roots and other complex algebraic expressions, reducing them to more manageable trigonometric forms.
| Original Expression | Substitution |
|---|---|
| a^2 - x^2 | \cos \theta |
| x^2 - a^2 | -\sin \theta |
| \sqrt{a^2 + x^2} | \sec \theta |

Substitution not only simplifies equations but also sometimes allows us to use known integration or algebraic techniques.
5. Utilize Factoring and Simplification

Sometimes, trigonometric equations can be simplified by factoring or using algebraic techniques:
- Factor out common trigonometric terms, similar to how you would with polynomials.
- Use the zero-product property to solve factored equations by setting each factor to zero.
This approach can reveal solutions that might not be immediately apparent, making complex equations more straightforward.
Wrapping Up

Mastering trigonometric equations involves understanding and applying various strategies. From recognizing and using trigonometric identities, graphing, leveraging the unit circle, employing substitution, to simplifying through factoring, each method provides a unique pathway to solutions. With practice, these techniques will become second nature, allowing you to tackle even the most complex trig equations with confidence.
How do trigonometric identities help solve equations?

+
Trigonometric identities provide shortcuts to simplify expressions and transform equations into forms where solving becomes easier.
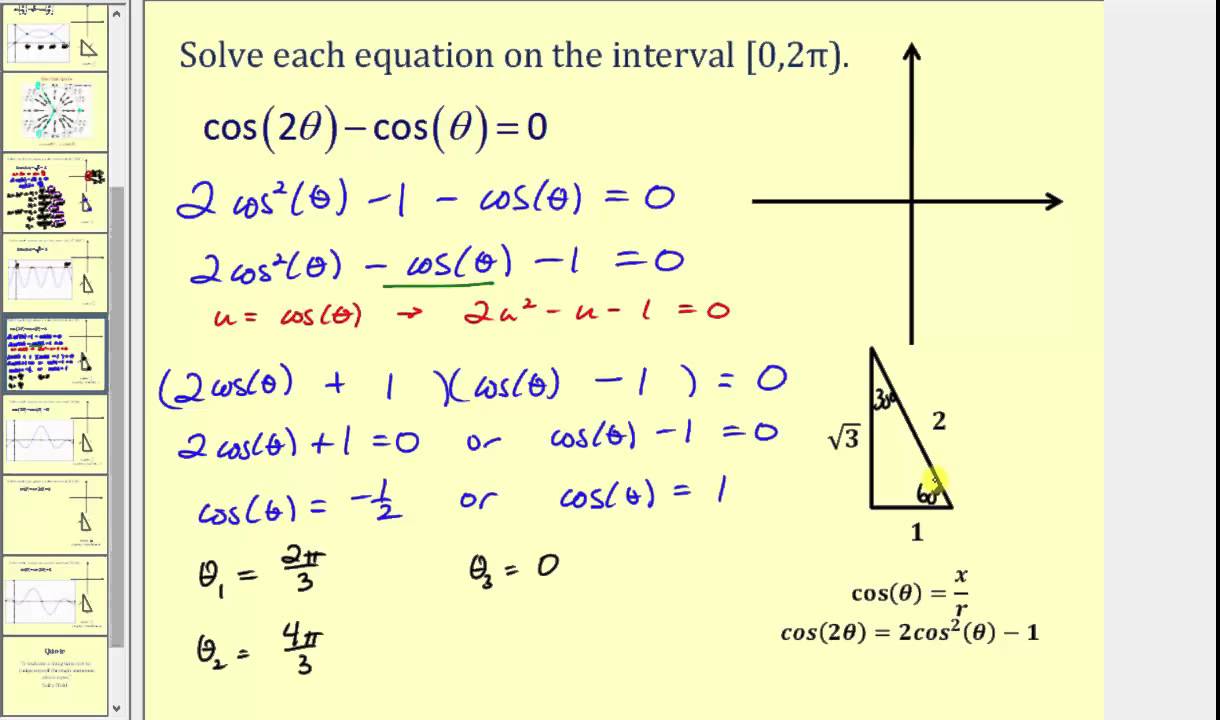
Can you solve trigonometric equations without graphing?
+
Yes, you can solve them algebraically, but graphing often helps visualize the solutions, making the process more intuitive.
Why is the unit circle important in trigonometry?

+
The unit circle provides a standardized way to understand and solve trigonometric equations by offering a visual reference for all angles and their corresponding trigonometric values.