Triangle Congruence Worksheet: Master with Answer Key

In the world of geometry, understanding triangle congruence is a fundamental skill that lays the groundwork for advanced mathematical reasoning and real-world applications. Whether you're a student diving into geometry for the first time or a seasoned educator looking to refresh your teaching materials, this comprehensive guide on triangle congruence worksheets with an answer key will be an invaluable resource.
Why Study Triangle Congruence?

Triangle congruence is essential because it allows us to determine when two triangles are identical, even if they are positioned differently in space. This knowledge is crucial for:
- Understanding properties and theorems related to shapes and angles.
- Architectural designs where exact measurements must be duplicated.
- Engineering, where parts need to be interchangeable.
- Problem-solving in both theoretical mathematics and practical applications.
💡 Note: Congruence is not about similarity; it’s about identicality in all respects.
Triangle Congruence Postulates

There are several postulates or criteria that help us determine whether two triangles are congruent:
Side-Side-Side (SSS)
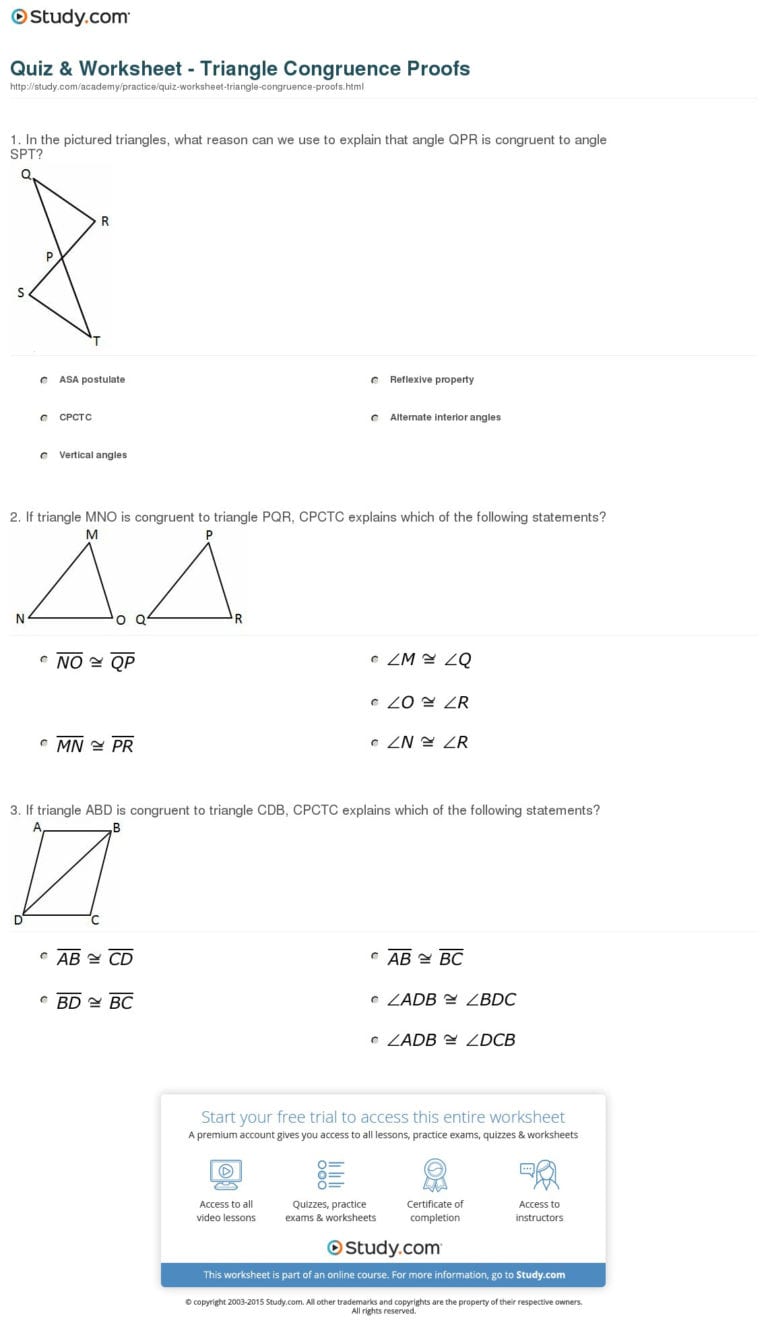
If the three sides of one triangle are equal to the three sides of another triangle, then the two triangles are congruent.
Side-Angle-Side (SAS)

Two triangles are congruent if two sides and the included angle of one triangle are equal to two sides and the included angle of the other triangle.
Angle-Side-Angle (ASA)

This postulate states that two triangles are congruent if two angles and the included side of one triangle are equal to two angles and the included side of the other triangle.
Angle-Angle-Side (AAS)

If two angles and a non-included side of one triangle are equal to two angles and the corresponding non-included side of another triangle, the triangles are congruent.
Hypotenuse-Leg (HL) - Only for Right Triangles

For right triangles, if the hypotenuse and one leg of one triangle are equal to the hypotenuse and one leg of another triangle, then the triangles are congruent.
Practical Example: Using Congruence Postulates

Here’s a practical example to illustrate how to use these postulates:
| Triangle 1 | Triangle 2 | Postulate Applied | Conclusion |
|---|---|---|---|
| A: 6, B: 8, C: 10 | D: 6, E: 8, F: 10 | SSS | Congruent |
| ∠PQR=75°, QR=5, ∠PRQ=35° | ∠LMN=75°, MN=5, ∠NML=35° | ASA | Congruent |
🔍 Note: Always ensure you check all corresponding sides and angles before concluding congruence.
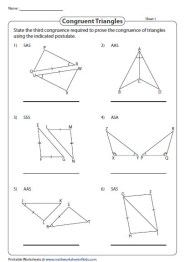
Creating an Effective Triangle Congruence Worksheet

When crafting a worksheet to teach or test triangle congruence:
- Include Various Types of Problems: Cover all congruence postulates with examples and exercises.
- Offer Visual Aids: Use diagrams or sketches to help students visualize triangles.
- Gradual Complexity: Start with simple comparisons and progressively increase difficulty.
- Answer Key: Provide an answer key for self-assessment, ensuring students can check their work without assistance.
Sample Triangle Congruence Worksheet

Below is a sample question from a worksheet:
| Problem | Diagram | Postulate | Congruence? |
|---|---|---|---|
| Are triangles XYZ and JKL congruent? Given XY=JK, YZ=KL, ∠XYZ=∠JKL | [Include diagram here] | SAS | Yes |
📊 Note: Ensure the worksheet covers all the postulates to give students a well-rounded understanding.
Utilizing the Answer Key
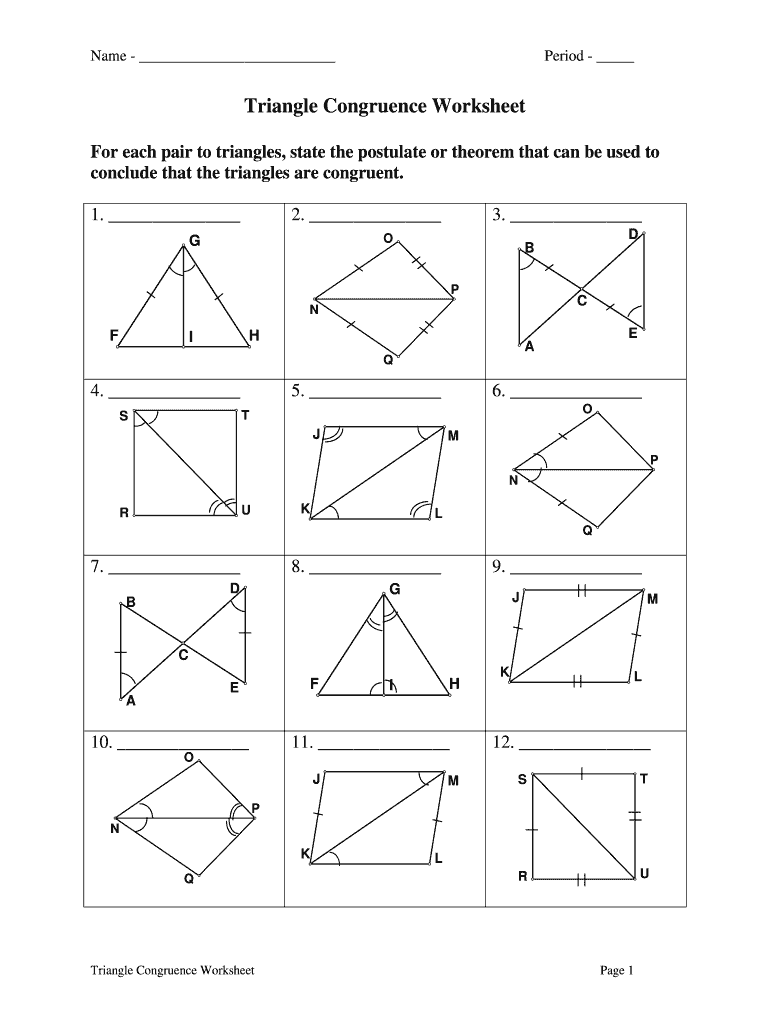
The answer key serves multiple purposes:
- It helps students to self-evaluate their understanding.
- It provides teachers with a tool to assess learning outcomes.
- It’s essential for homework or quizzes, offering immediate feedback.
The journey through triangle congruence is not just about mastering a topic but also about appreciating the beauty and logic in geometry. By understanding how triangles can be congruent, students develop spatial awareness and problem-solving skills that transcend mere academic requirements. This guide serves as a roadmap to understanding and mastering triangle congruence, making it easier for both students and educators to navigate through this fascinating aspect of mathematics.
What are the five main triangle congruence postulates?

+
The five main triangle congruence postulates are Side-Side-Side (SSS), Side-Angle-Side (SAS), Angle-Side-Angle (ASA), Angle-Angle-Side (AAS), and for right triangles only, Hypotenuse-Leg (HL).
Why can’t you use Angle-Angle-Angle (AAA) to prove congruence?

+
While AAA can prove similarity, it cannot prove congruence because two triangles can have all angles equal yet still have different side lengths, making them similar but not congruent.
How can I identify which postulate to use?

+
Identify which parts (sides or angles) of the triangles are given or known. Then, match these with the postulates:
- SSS if three sides are known.
- SAS if two sides and the included angle are known.
- ASA or AAS if two angles and one side are known.
- HL for right triangles with the hypotenuse and one leg.



