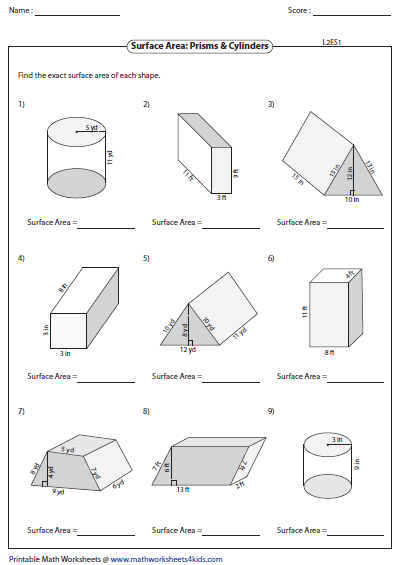
Mastering Surface Area of Prisms: Rectangular and Triangular Worksheets

🔧 Note: This post uses html formatted contents for a better reading experience.
Understanding Surface Area: A Mathematical Overview

The concept of surface area is fundamental in the study of geometry. It's the total area that covers the surface of a three-dimensional object. When it comes to prisms, which are polyhedra with two parallel and congruent polygonal bases, understanding surface area involves knowing how to calculate the area of each face of the prism, whether it's a rectangular prism or a triangular prism.
Geometry isn't just an abstract study; it has practical applications ranging from architecture to engineering. Calculating surface area aids in determining material quantities for construction, estimating costs, and even in everyday tasks like painting or wrapping gifts. Let's dive into the specifics of prisms and how to master their surface area calculations.
The Anatomy of Rectangular Prisms

A rectangular prism, also known as a cuboid or a rectangular box, has six rectangular faces where opposite sides are equal. The two bases are congruent rectangles, and the sides are also rectangles.
- Bases: Two rectangles with the same area.
- Sides: Four rectangles, two of which are congruent pairs.
To find the total surface area (SA) of a rectangular prism, you sum the areas of all six faces:
SA = 2lw + 2lh + 2wh
Where:
- l is the length of the prism.
- w is the width of the prism.
- h is the height of the prism.
This formula ensures that all faces are accounted for in the calculation. Let's look at an example to clarify this process:
Practical Example: Calculating the Surface Area of a Rectangular Prism

Suppose we have a rectangular prism with dimensions as follows:
| Dimension | Value |
|---|---|
| Length (l) | 5 meters |
| Width (w) | 4 meters |
| Height (h) | 3 meters |

Calculating the surface area:
SA = (2 * 5 * 4) + (2 * 5 * 3) + (2 * 4 * 3)
SA = 40 + 30 + 24
SA = 94 m²
🔧 Note: Pay close attention to units when calculating surface area; all dimensions should use the same units.
The Anatomy of Triangular Prisms

A triangular prism has five faces: two triangular bases and three rectangular lateral faces. Here’s how to approach its surface area:
- Bases: Two congruent triangles.
- Sides: Three rectangles, with the length of each side equal to the corresponding side of the base triangle.
The formula for the surface area of a triangular prism can be written as:
SA = b + ph + B
Where:
- b is the area of one of the triangular bases.
- p is the perimeter of one of the triangular bases.
- h is the height (length) of the prism.
- B is the area of the triangular base.
Here's a visual representation of a triangular prism:
Let’s illustrate this with an example:
Practical Example: Calculating the Surface Area of a Triangular Prism

Consider a triangular prism with:
- Base side lengths: 3 meters, 4 meters, and 5 meters (right angled triangle)
- Height of the prism (h): 6 meters
First, calculate the area of the triangular base:
B = (1/2) * b * h = (1/2) * 3 * 4 = 6 m²
Then, the perimeter of the base:
p = 3 + 4 + 5 = 12 meters
Finally, apply the formula for surface area:
SA = 6 + (12 * 6) + 6 = 6 + 72 + 6 = 84 m²
Worksheets as a Learning Tool

Worksheets provide a structured approach to practicing and understanding surface area calculations. Here are some benefits of using worksheets:
- Practice: They allow students to work through numerous problems, reinforcing their understanding.
- Visualization: Diagrams on worksheets can help in visualizing the problem, making abstract concepts more concrete.
- Interactive Learning: Worksheets can be designed to include interactive elements, like color coding or cutouts, to engage students further.
Tips for Teaching Surface Area with Worksheets

- Start Simple: Begin with basic rectangular prisms before moving to more complex shapes like triangular prisms.
- Use Real-World Examples: Connect the math to real-life applications, such as calculating the area needed for a paint job.
- Encourage Sketching: Have students sketch the prisms they're working with to better understand the shapes.
- Break Down the Steps: Clearly delineate each step of the surface area calculation process.
🔧 Note: Worksheets should be supplemented with explanations, visual aids, and practice problems for a well-rounded learning experience.
Mastering the calculation of surface area for prisms not only involves understanding the geometric shapes but also knowing how to apply mathematical formulas to solve problems. With a blend of theory and practical application through worksheets, students can develop a solid foundation in this aspect of geometry. By grasping how each face contributes to the overall surface area, they can confidently tackle both rectangular and triangular prisms, understanding their applications in various real-world scenarios. This skill also promotes logical thinking, problem-solving, and a deeper appreciation for the spatial relationships in our three-dimensional world.
What’s the difference between a prism and a pyramid?

+
A prism has two identical bases that are polygons, and its sides are parallelograms. A pyramid, on the other hand, has a single polygonal base and triangular faces that meet at a common point, called the apex.
How do I calculate the surface area if the prism isn’t regular?
+
If the prism has non-congruent sides, you will need to calculate each lateral face’s area individually before summing them up with the area of the bases.
Why is understanding surface area important?

+
Calculating surface area is crucial for tasks like determining material quantities for packaging, construction, painting, and in scientific fields where the surface area can affect chemical reactions or heat transfer.