Angle Pair Worksheet Answer Key Revealed

Exploring geometry often involves understanding the intricate relationships between angles, particularly when they come in pairs. This comprehensive guide aims to unravel the mysteries behind angle pair relationships, provide detailed answers to common angle pair worksheets, and enhance your geometric reasoning skills.
Understanding Angle Pairs

Geometry teaches us about various types of angle pairs, each defined by their position relative to intersecting lines. Here’s a quick overview:
- Adjacent Angles - Angles sharing a common vertex and a common side.
- Vertical Angles - Pairs of opposite angles formed by two intersecting lines.
- Complementary Angles - Angles that add up to 90 degrees.
- Supplementary Angles - Angles that add up to 180 degrees.
- Linear Pairs - Adjacent and supplementary angles.

Decoding the Worksheet

Let’s delve into common questions found on angle pair worksheets:
Problem 1: Identifying Angle Types
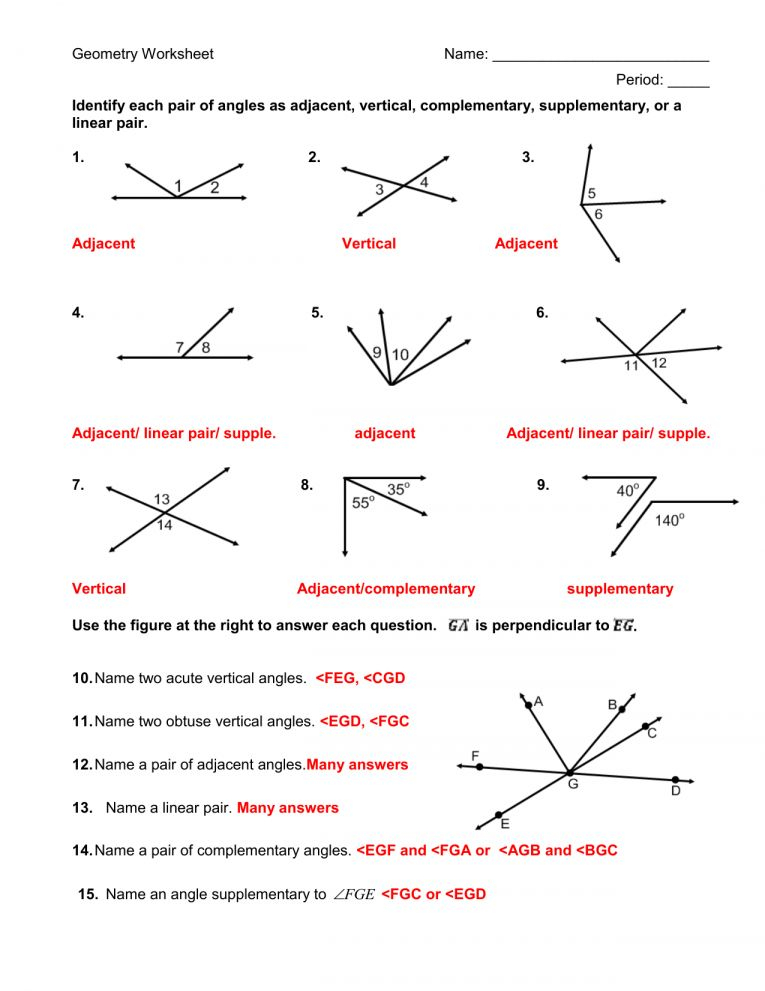
Given angles are:
- Angle A = 50°
- Angle B = 130°
Identify whether these are complementary, supplementary, adjacent, or none:
| Angle Pair | Relationship |
|---|---|
| Angle A + Angle B | Supplementary |
| Angle A + Angle B | Not Adjacent |
| Angle A + Angle B | Not Complementary |

📝 Note: Adjacent angles must share a side, which is not the case here.
Problem 2: Solving for Unknown Angles

Given that Angle A is complementary to Angle B, and Angle A measures 25°:
Find Angle B:
Since Angle A + Angle B = 90°, Angle B = 90° - 25° = 65°.
Problem 3: Linear Pairs

Two lines intersect creating angles:
- Angle X = 105°
- Angle Y = unknown
Since X and Y form a linear pair:
Angle Y = 180° - 105° = 75°.
Strategies for Mastering Angle Pairs

To excel in solving angle pair problems:
- Memorize Definitions - Know the definitions of each angle pair type.
- Visualize Relationships - Draw diagrams to help visualize how angles relate.
- Check for Adjacency - Determine if angles are adjacent before deciding on their relationship.
- Practice Algebraic Problems - Angles often involve algebraic expressions to find unknown values.
As you grow more familiar with these strategies, you’ll find that solving angle pair problems becomes second nature.
In summary, understanding angle pairs is foundational to mastering geometry. This blog post has provided an in-depth look at how to identify and solve common angle pair problems, from supplementary and complementary angles to linear pairs and adjacent angles. With these tools in hand, you are well-equipped to tackle any angle pair worksheet or geometric challenge you encounter.
What makes angle pairs important in geometry?

+
Understanding angle pairs helps in solving many geometric problems, from calculating the measures of angles to proving geometric theorems. They provide insight into how lines and angles interact in a plane.
How do I identify if angles are adjacent?

+
Adjacent angles share a common side and a common vertex but do not overlap. They are next to each other.
Can an angle pair be both complementary and supplementary?

+
No, because complementary angles add up to 90° while supplementary angles add up to 180°. An angle pair can only be one or the other, not both.
What’s the difference between linear pairs and vertical angles?

+
Linear pairs are adjacent angles that add up to 180°. Vertical angles are opposite angles formed by intersecting lines, which are always equal.
Why do I need to understand angle pairs for real-life applications?

+
Angle pairs are used in architecture, engineering, surveying, and even art to determine angles for structural integrity, designs, and alignments. Understanding these relationships helps in practical applications and problem-solving.