5 Key Stoichiometry Worksheet Answers Unlocked

In the intricate world of chemistry, stoichiometry forms the backbone of understanding how reactants transform into products in chemical reactions. For students and enthusiasts keen on grasping this fundamental concept, worksheets act as a litmus test for their comprehension. Here, we delve into five crucial stoichiometry worksheet questions, providing detailed solutions that unravel the mystery behind these reactions.

Understanding the Basics of Stoichiometry

Before diving into the specifics, let's clarify what stoichiometry entails. Stoichiometry is the calculation of reactants and products in chemical reactions using balanced equations. The key here is the mole ratio, which acts as a conversion factor to determine the quantities involved in reactions.

• The mole ratio is derived from the coefficients in a balanced chemical equation.
• Using these ratios, you can compute how much of one substance is needed or produced given the amount of another substance.

Question 1: The Mole-to-Mole Ratio

Here's a sample stoichiometry problem:

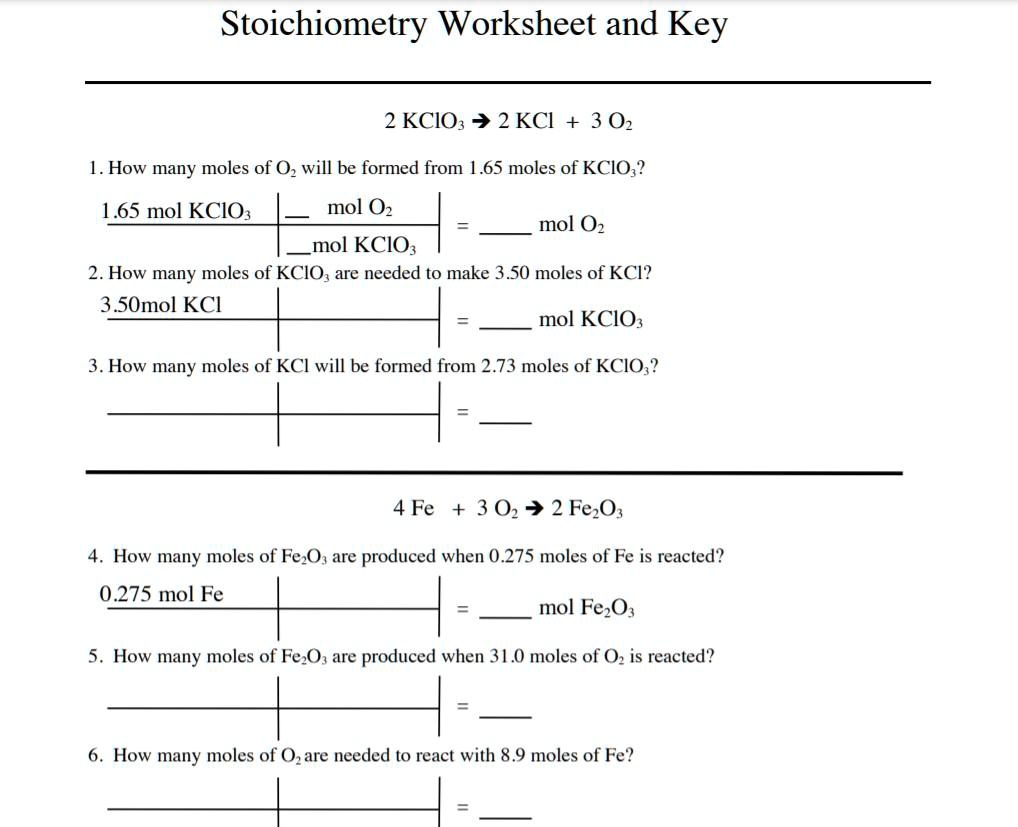
Given the reaction: 
2H2 + O2 → 2H2O

How many moles of water can be produced if 5 moles of hydrogen react with an excess of oxygen?

To solve this:

1. Identify the mole ratio from the balanced equation:

2 moles of H₂ : 2 moles of H₂O

2. Set up the calculation based on the given amount:

5 moles of H₂ × (2 moles of H₂O / 2 moles of H₂) = 5 moles of H₂O

3. Answer: 5 moles of water can be produced.

Question 2: Mass-to-Mass Calculations

Consider this scenario:

If 15 grams of oxygen reacts with hydrogen, how many grams of water can be formed?

Follow these steps:

1. Convert the mass of O₂ to moles using the molar mass (32 g/mol):

15 g ÷ 32 g/mol = 0.46875 moles of O₂

2. Find the mole ratio from the balanced equation:

1 mole of O₂ : 2 moles of H₂O

3. Calculate moles of H₂O:

0.46875 moles of O₂ × (2 moles of H₂O / 1 mole of O₂) = 0.9375 moles of H₂O

4. Convert moles of H₂O back to grams using the molar mass (18 g/mol):

0.9375 moles × 18 g/mol = 16.875 grams of H₂O

5. Answer: 16.88 grams of water can be formed.

Question 3: Limiting Reactants

Let's consider a reaction where excess isn't specified:

For the reaction: N2 + 3H2 → 2NH3

If 2 moles of nitrogen and 4 moles of hydrogen react, how much ammonia can be produced?

Here's how to tackle this:

1. Calculate the moles of NH₃ produced if all N₂ were to react:

2 moles N₂ × (2 moles NH₃ / 1 mole N₂) = 4 moles NH₃

2. Calculate the moles of NH₃ produced if all H₂ were to react:

4 moles H₂ × (2 moles NH₃ / 3 moles H₂) = 2.67 moles NH₃

3. Hydrogen is the limiting reactant, as it produces less NH₃.
4. Answer: 2.67 moles of ammonia can be produced.

⚠️ Note: In real-world scenarios, reactant availability might not always match theoretical values, leading to a limiting reactant scenario.

Question 4: Theoretical vs. Actual Yield

Given the reaction:

C3H8 + 5O2 → 3CO2 + 4H2O

If 100 grams of propane are burned in excess oxygen, how much CO2 is theoretically produced? If the actual yield is 120 grams, what is the percent yield?

Follow these steps:

1. Calculate the theoretical moles of C₃H₈:

100 g ÷ 44 g/mol = 2.27 moles of C₃H₈

2. Find the mole ratio:

1 mole C₃H₈ : 3 moles CO₂

3. Calculate theoretical moles of CO₂:

2.27 moles × 3 = 6.81 moles CO₂

4. Convert moles to grams:

6.81 moles × 44 g/mol = 300.04 grams CO₂

5. Calculate the percent yield:

(120 g / 300.04 g) × 100% = 40%

6. Answer: The percent yield is 40%.

Question 5: Complex Stoichiometry with Limiting Reactants

Consider this scenario:

Given the balanced equation:

3A + 5B → 2C + 4D

If you have 10 moles of A and 8 moles of B, how many moles of D can be produced?

Here’s how to solve this:

1. Find moles of D if all A is used:

10 moles A × (4 moles D / 3 moles A) = 13.33 moles D

2. Find moles of D if all B is used:

8 moles B × (4 moles D / 5 moles B) = 6.4 moles D

3. B is the limiting reactant here.
4. Answer: 6.4 moles of D can be produced.

In summary, stoichiometry enables chemists to predict outcomes of chemical reactions with precision. It's not just about balancing equations but understanding the relationships between substances at a molecular level. This guide has walked you through the key calculations involved in stoichiometry, from mole ratios to limiting reactants, providing insights into how we can quantify chemistry reactions. Whether for academic pursuits or practical applications, mastering stoichiometry is invaluable in navigating the world of chemical reactions.

The intricate dance of molecules in a reaction is governed by stoichiometric principles, allowing us to anticipate the products from given reactants, manage resources efficiently, and understand the chemistry behind everyday phenomena.

Why do we need to balance chemical equations before stoichiometry?

+

A balanced chemical equation provides the correct ratios of reactants to products, ensuring the conservation of mass and the ability to calculate accurate yields and requirements.

Can actual yield ever exceed theoretical yield?

+

Typically, no. Actual yield can approach but not exceed theoretical yield due to inefficiencies or side reactions, but with very high efficiency or additional reactions forming the desired product, it can theoretically match or slightly exceed expectations.

How does stoichiometry apply outside of a laboratory?

+

Stoichiometry is crucial in industrial processes, pharmaceuticals, environmental chemistry, and even cooking, where understanding reactant ratios ensures optimal results.

What role does a catalyst play in stoichiometry?

+

A catalyst speeds up a chemical reaction but does not change the stoichiometry of the reaction itself. It affects only the kinetics, not the thermodynamics or the final product amounts.