Master Proportions Easily: 7th Grade Worksheet Guide

Understanding proportions is an essential skill that not only serves students well in mathematics but also in everyday life. From cooking and scaling recipes to interpreting data in various scientific fields, proportions are everywhere. This guide aims to provide 7th graders with a comprehensive walkthrough on mastering proportions through an easy-to-understand worksheet guide. By following these steps, students will enhance their math skills and ensure they are well-prepared for more advanced math concepts in the future.
Understanding Proportions

Before diving into practical examples, let’s clarify what proportions are:
- Definition: A proportion is an equation which states that two ratios are equivalent. For example, 2:3 = 4:6 is a proportion.
- Purpose: Proportions are used to compare quantities and find missing values when two ratios are known.
Here’s a visual representation of proportions:
| First Ratio | Equals | Second Ratio |
|---|---|---|
| 2 | 3 | |
| 4 | 6 |

Key Concepts for 7th Grade
At the 7th grade level, students are expected to understand:
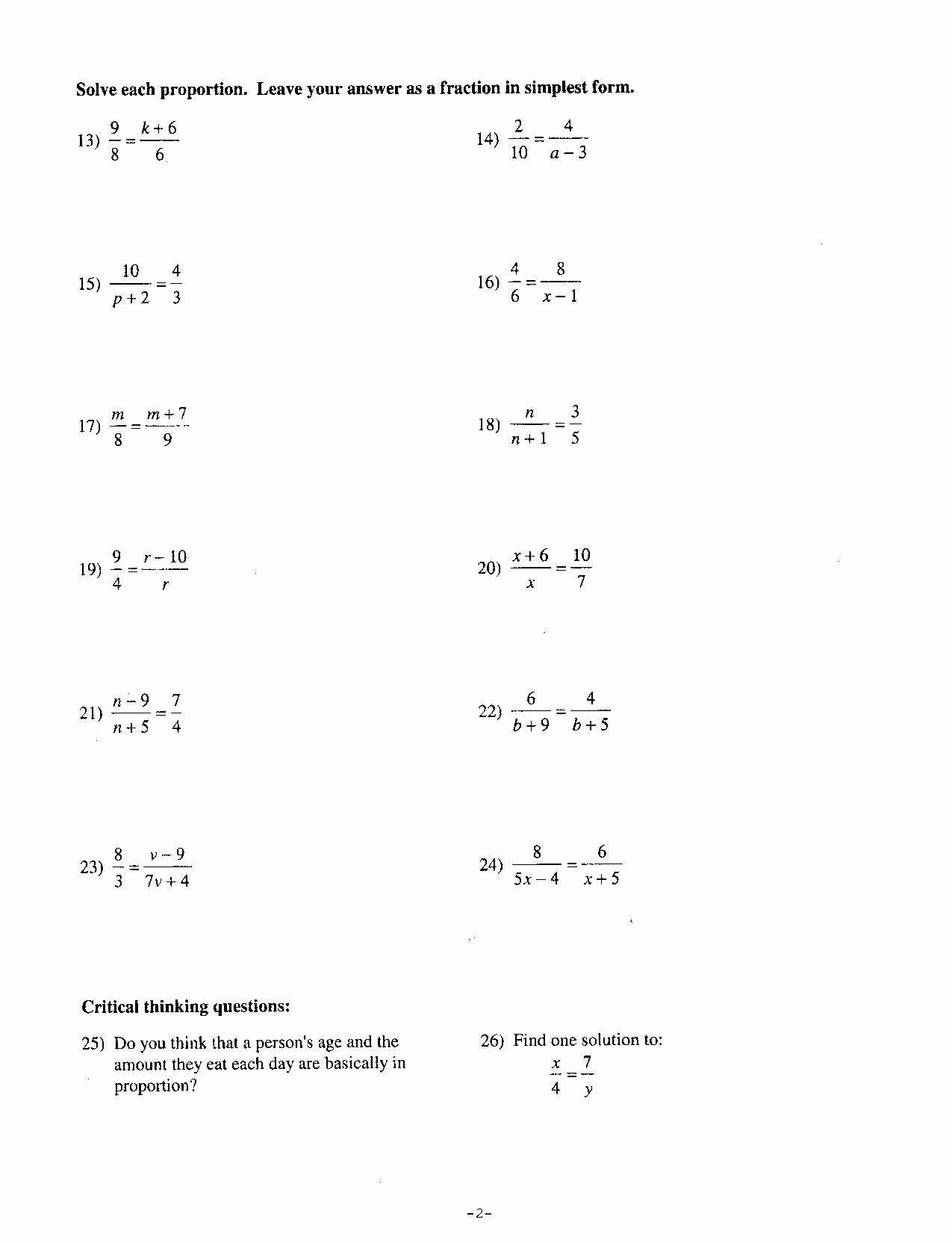
- How to cross-multiply to solve for an unknown in a proportion.
- The difference between direct and inverse proportions.
- Using proportions to solve real-world problems.
Cross Multiplication

To solve a proportion, you can use the cross-multiplication method, where:
a/b = c/d becomes ad = bc
Here’s how to do it:
- Multiply the numerator of the first ratio by the denominator of the second.
- Multiply the numerator of the second ratio by the denominator of the first.
- Set these two products equal to each other to solve for the unknown variable.
💡 Note: This method ensures the proportionality of both sides of the equation.
Direct and Inverse Proportions

Let's distinguish between these two types:
- Direct Proportions: When two quantities increase or decrease together in such a way that their ratio remains constant. For example, if you double the amount of apples in a recipe, you also double the sugar.
- Inverse Proportions: When an increase in one quantity results in a decrease in another, keeping the product constant. An example is speed and time in a fixed distance scenario; if speed increases, time decreases.
Practical Application: 7th Grade Worksheet

Here is a set of exercises to practice proportions:
Worksheet Example

| Problem | Solution |
|---|---|
| 3/x = 6⁄12 | x = 6 |
| y/10 = 4⁄5 | y = 8 |
| 9 : 3 = 3 : z | z = 1 |
🌟 Note: Always check your answers by ensuring that the ratios are equal.
Real-Life Examples

Let’s apply proportions to real-life scenarios:
- Cooking: If a recipe calls for 2 eggs for 10 people, how many eggs do you need for 25 people?
- Travel: If you can travel 60 miles in 2 hours, how far can you travel in 5 hours at the same speed?
- Economics: If the exchange rate is 1 = IDR 14,500, how many Rupiah will you get for 50?
Understanding how to apply proportions to real-life situations not only reinforces mathematical concepts but also enhances problem-solving skills outside the classroom.
Mastering proportions at this stage sets a solid foundation for advanced algebra, geometry, and even higher-order thinking skills necessary for science and engineering. By understanding how to manage ratios and solve for unknowns, students develop critical thinking abilities that are beneficial in various academic and real-world settings. Keep practicing with proportions to sharpen your mathematical prowess, and soon, you'll find these problems easier and more intuitive.
What is a proportion?

+
A proportion is an equation stating that two ratios or fractions are equal.
How do you solve a proportion?

+
To solve a proportion, you can use the cross-multiplication method where you set the product of the numerators and denominators equal to each other and solve for the unknown variable.
What’s the difference between direct and inverse proportions?

+
In a direct proportion, if one quantity increases, the other increases too, keeping the ratio constant. In an inverse proportion, as one quantity increases, the other decreases, keeping the product constant.



