Master Multi-Step Inequalities with Our Worksheet Guide

In mathematics, inequalities are statements that compare two values or expressions, indicating which one is greater or lesser than the other. Multi-step inequalities, in particular, require a series of operations to solve, making them a crucial part of algebra education. This guide will walk you through mastering these inequalities with a comprehensive worksheet approach.
Understanding Multi-Step Inequalities

Before diving into the worksheet, it's vital to grasp what multi-step inequalities entail. Unlike simple inequalities, multi-step inequalities involve several algebraic steps to isolate the variable. These steps might include:
- Distributing variables across parentheses.
- Combining like terms.
- Performing operations to isolate the variable.
- Dealing with fractions or decimals.
📝 Note: Always remember the rules of inequality: multiplying or dividing by a negative number reverses the inequality sign.
Step-by-Step Guide to Solving Multi-Step Inequalities

Here is a step-by-step guide to solving multi-step inequalities:
Step 1: Distribute and Simplify
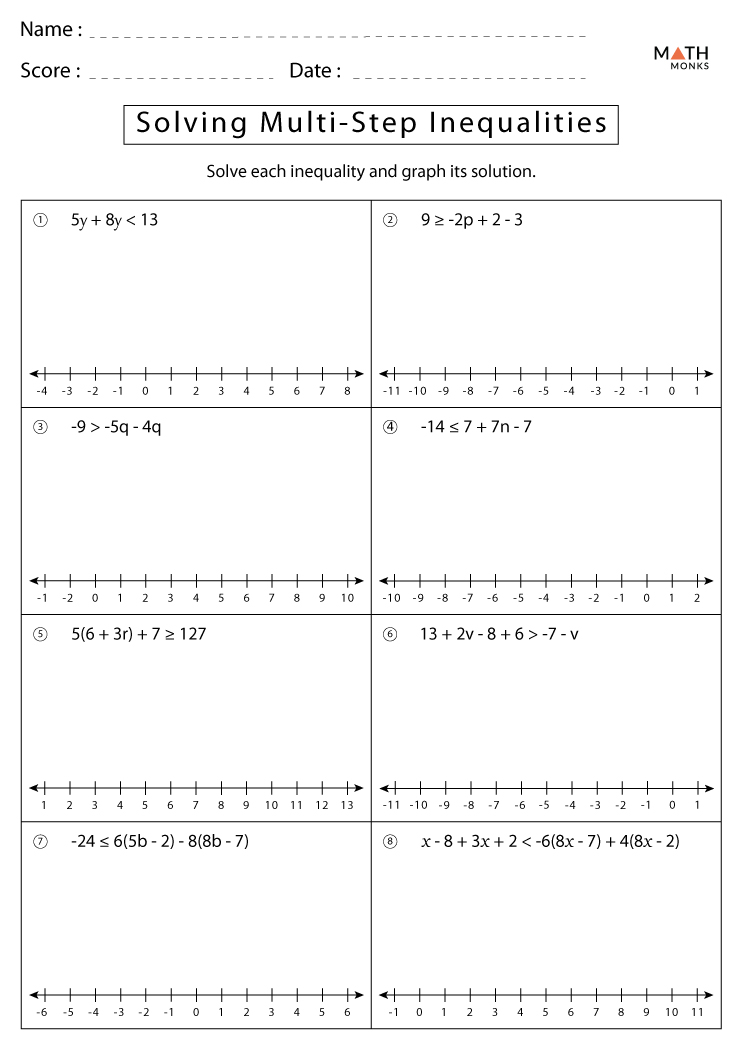
If the inequality contains terms inside parentheses, distribute the variable or constant outside to every term within the parentheses.
Step 2: Combine Like Terms

Combine any like terms on both sides of the inequality to simplify your expression. This reduces clutter and helps to focus on isolating the variable.
Step 3: Move Variables to One Side

Get all the variable terms on one side of the inequality and constant terms on the other. This step often involves adding or subtracting variables or constants to either side of the equation.
Step 4: Isolate the Variable

Use multiplication or division to isolate the variable. Remember, if you multiply or divide by a negative number, the direction of the inequality sign changes.
Step 5: Check Your Solution

Plug your solution back into the original inequality to ensure it satisfies all conditions. This step is crucial for verifying the correctness of your solution.
📝 Note: While solving, maintain the same inequality sign unless you multiply or divide by a negative number.
Using Worksheets to Practice

Worksheets are an excellent tool for honing your skills in solving multi-step inequalities. Here's how to utilize them effectively:
- Start with Basic Problems: Begin with simpler inequalities to solidify your understanding of each step.
- Progress to Complex Problems: Gradually increase the complexity as you become more comfortable.
- Check Your Work: Use answer keys or solutions provided with the worksheet to check your accuracy.
- Time Yourself: To simulate test conditions, time yourself to see how long it takes to solve each problem.
- Identify Mistakes: Review your mistakes to understand where you commonly stumble.
| Type of Inequality | Difficulty Level |
|---|---|
| Simple Inequalities (e.g., x > 5) | Beginner |
| Two-Step Inequalities (e.g., 3x + 2 < 8) | Intermediate |
| Multi-Step Inequalities with Distributing (e.g., 2(x - 3) > 5) | Advanced |
| Multi-Step Inequalities with Fractions (e.g., (x/2) + 4 > 10) | Advanced |

📝 Note: Practicing regularly on worksheets helps in identifying common error patterns, which is essential for improvement.
Mastering the Technique

Mastering multi-step inequalities involves consistent practice, understanding the core concepts, and adapting to different types of problems:
- Understand the Steps: Knowing the steps by heart reduces the cognitive load during problem-solving.
- Focus on Signs: Pay attention to when you need to flip the inequality sign.
- Use Graphing: Sometimes, graphing inequalities can help visualize the solution set, making it easier to check.
- Review Mistakes: Analyzing your errors in detail can prevent future mistakes.
- Apply Real-World Scenarios: Understanding how inequalities can represent real-world situations can deepen your understanding.
In summary, mastering multi-step inequalities through worksheets provides a structured approach to enhance your algebraic skills. The consistent practice helps in internalizing the steps, reducing errors, and increasing speed and accuracy in solving inequalities. This guide has offered insights into the process, from understanding the theory to practical application, ensuring you are well-equipped to tackle any multi-step inequality you encounter.
What are multi-step inequalities?

+
Multi-step inequalities are algebraic expressions that require several operations to solve, typically involving distribution, combining like terms, and isolating the variable to find the solution set.
Why do we sometimes change the inequality sign?

+
When you multiply or divide both sides of an inequality by a negative number, the inequality sign must be flipped to maintain the truth of the statement.
Can I use the distributive property in solving inequalities?

+
Yes, just like in solving equations, you can use the distributive property to eliminate parentheses and simplify expressions before isolating the variable.
What’s the best way to practice solving multi-step inequalities?
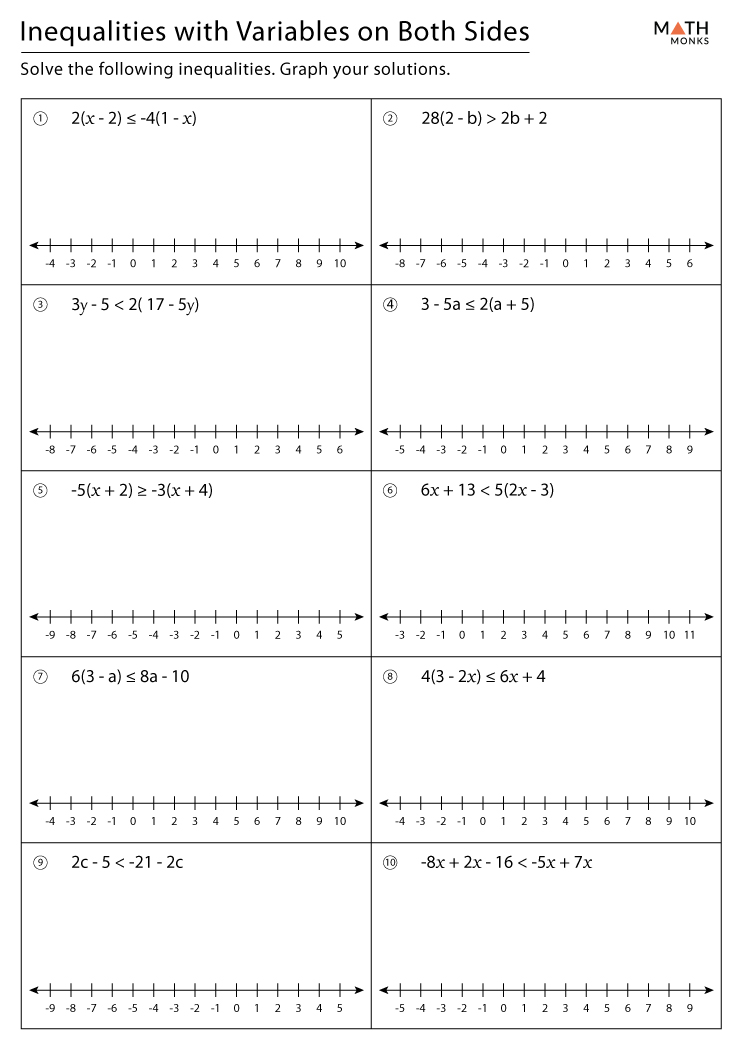
+
The best way is through consistent practice using worksheets or problem sets that vary in difficulty. Start with simple problems and gradually increase complexity.
How can real-world scenarios help in understanding inequalities?

+
Real-world scenarios provide context, making abstract mathematical concepts more tangible, and help students see the practical applications of inequalities in daily life.