5 Essential Tips for Mastering Exponent Properties

The journey through algebra often involves confronting exponents in various forms, which can be daunting at first. However, understanding exponent properties can simplify complex expressions and pave the way for a more intuitive understanding of algebra. This guide provides five essential tips for mastering exponent properties, transforming what might seem like abstract rules into practical tools for problem-solving.
Understand the Basic Definitions
Before diving into the deeper rules and applications, it’s crucial to have a firm grasp of what exponents are. An exponent refers to the power of a number, dictating how many times that number (the base) is multiplied by itself. For example:
- Definition: an = a × a × … × a (n times)
- Parts: In 34, 3 is the base and 4 is the exponent or power.

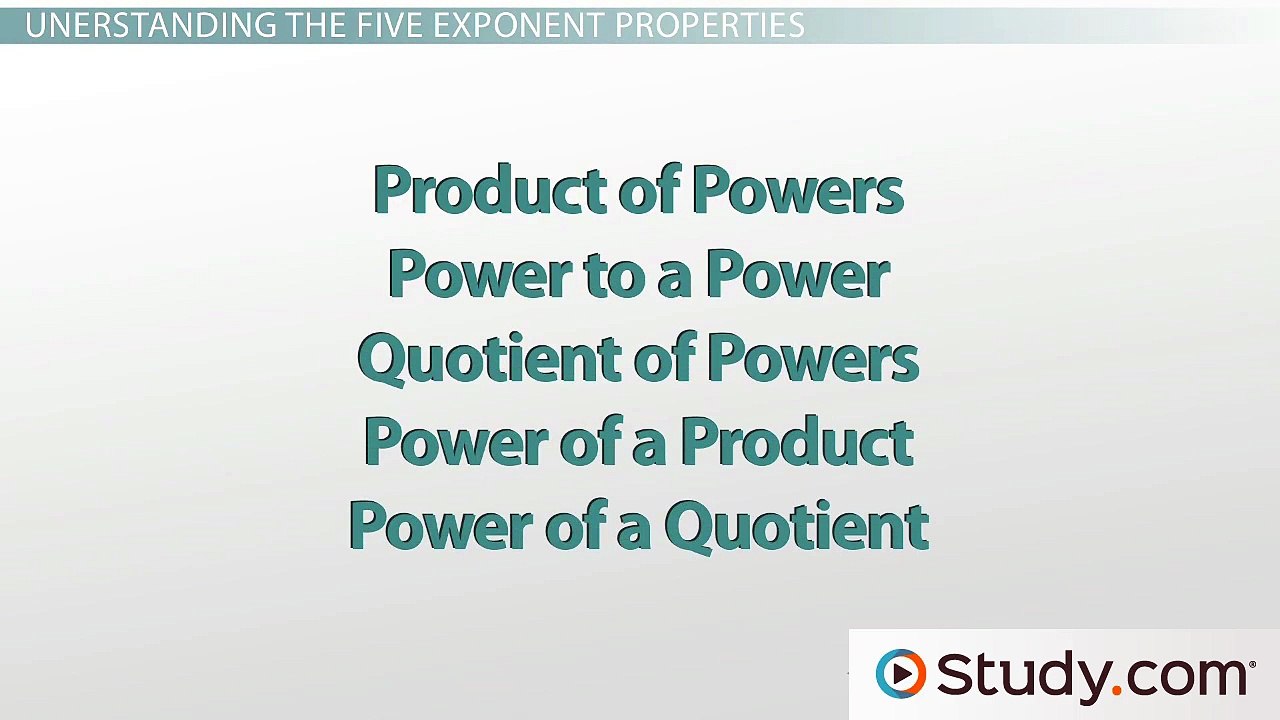
Learn the Fundamental Exponent Laws

To effectively manage exponents, it’s essential to understand these key properties:
| Exponent Property | Description | Example |
|---|---|---|
| Product of Powers | am × an = am+n | 23 × 22 = 25 |
| Quotient of Powers | am ÷ an = am-n | 56 ÷ 52 = 54 |
| Power of a Power | (am)n = am×n | (32)3 = 36 |
| Power of a Product | (ab)n = an × bn | (2 × 4)3 = 23 × 43 |
| Power of a Quotient | (a ÷ b)n = an ÷ bn | (4 ÷ 2)2 = 42 ÷ 22 |
| Negative Exponents | a-n = 1 ÷ an | 2-3 = 1 ÷ 23 |
| Zero Exponent | a0 = 1 (for a ≠ 0) | 50 = 1 |


🚨 Note: Always remember these properties form the foundation for more complex algebraic operations.
Practice Simplification

Mastering the application of exponent rules comes with practice. Here are steps to simplify expressions:
- Identify bases and exponents clearly.
- Apply the appropriate exponent rule(s) to simplify.
- Repeat if necessary, as often expressions need multiple steps.
For example, to simplify (32)4 ÷ 93:
- (32)4 = 38 (Power of a Power).
- 93 = (32)3 = 36 (Power of a Product).
- 38 ÷ 36 = 38-6 = 32 (Quotient of Powers).
💡 Note: Remember to look for opportunities to combine exponents using these rules.
Use Exponent Properties in Real-World Applications

Exponents don’t just exist in textbooks; they’re everywhere in the real world:
- Finance: Compound interest calculations often use exponents.
- Physics: Growth models, like exponential decay or population growth, rely on exponential functions.
- Computing: Data storage and information technology often deal with powers of two for memory allocation.
Understanding and applying exponent properties can help in:
- Solving real-world problems more efficiently.
- Recognizing exponential growth or decay patterns.
- Managing computational complexities.
Master the Conversion Between Exponent and Radical Form

Exponents and radicals (or roots) are two sides of the same coin. Here’s how to convert between them:
- Radical to Exponent: √a = a1⁄2, ∛a = a1⁄3, etc.
- Exponent to Radical: a1/n = n√a
This knowledge is particularly useful when dealing with:
- Simplifying expressions with fractional exponents.
- Performing operations that involve both exponents and roots.

📚 Note: This conversion is fundamental for higher-level math and physics courses.
By embracing these five tips, you'll find that the world of exponents opens up, providing you with the tools to solve algebraic expressions with both speed and understanding. The knowledge of exponent properties isn't just for tests or textbooks; it’s a fundamental part of quantitative reasoning that applies across a spectrum of real-life situations. As we explore these properties, we not only become proficient in algebra but also enhance our capability to understand exponential growth, decay, and even the compound interest in finance. This is your roadmap to mastering exponent properties, ensuring that what was once a challenging area becomes an avenue for swift and insightful problem-solving.
What are the common mistakes when dealing with exponents?

+
Common mistakes include confusing the base and the exponent, not recognizing negative or zero exponents, incorrectly applying the rules to different bases, and misusing parentheses when raising expressions to a power.
How can understanding exponents help in other areas of mathematics?

+
Exponent properties are foundational for calculus, where you’ll deal with logarithms, derivatives, and integrals. They also help in understanding polynomial functions, exponential growth, and geometric series in statistics and probability.
Can exponents ever result in negative values?

+
If the base is negative and the exponent is even, then the result is positive. However, if the exponent is odd, the result will be negative. For example, (-2)3 = -8, but (-2)2 = 4.
What’s the significance of zero exponents?

+
Any non-zero number raised to the power of zero equals one. This concept is crucial for understanding how to manipulate expressions when terms are dividing or canceling each other out in equations.
What are some real-life applications where understanding exponents is useful?

+
Exponents are used in finance for compound interest, in physics for modeling exponential growth or decay, in chemistry for reaction rates, in computer science for data storage and algorithms, and in statistics for exponential distributions.