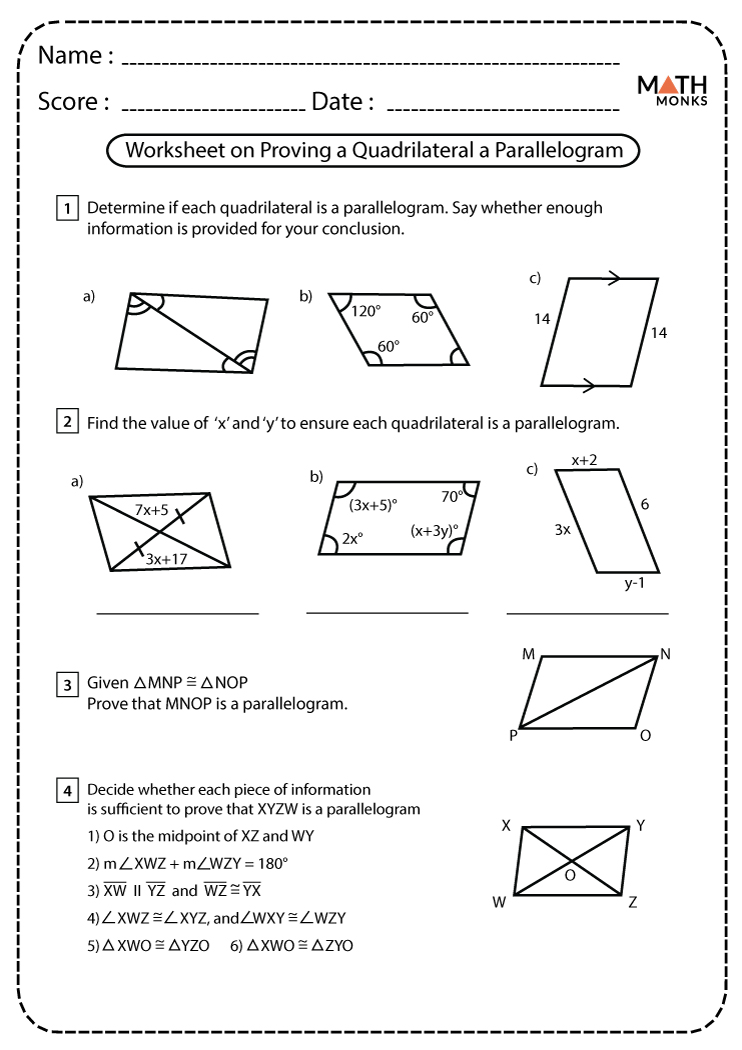
Master Linear Equations with Our Practice Worksheet
Linear equations are not just a cornerstone of algebra but are also vital for understanding more complex mathematical principles. Whether you're a student grappling with algebra, a teacher preparing lesson plans, or a curious mind exploring the language of mathematics, mastering linear equations can unlock numerous mathematical concepts. Let's delve deep into the world of linear equations, how to practice, and why they are important through a carefully designed worksheet.
Understanding Linear Equations


A linear equation is an equation for a straight line, expressed in the form of:
[ y = mx + b ]- m: represents the slope of the line.
- b: represents the y-intercept, the point where the line crosses the y-axis.
📚 Note: Linear equations can be manipulated into other forms like point-slope form or intercept form, which are useful in different scenarios.
Why Practice Linear Equations?

Practicing linear equations offers several benefits:
- Improves problem-solving skills by teaching logical steps to solve equations.
- Enhances understanding of how variables and constants interact.
- Provides a foundation for more advanced mathematical topics like calculus.
- Develops analytical thinking and fosters mathematical confidence.
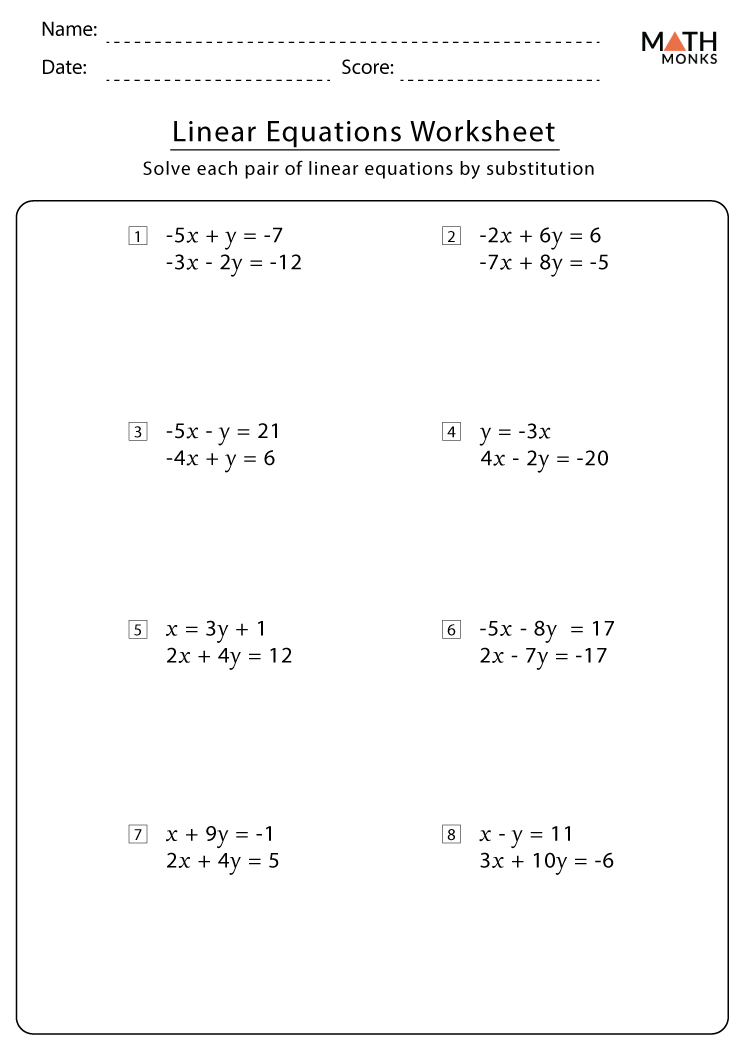
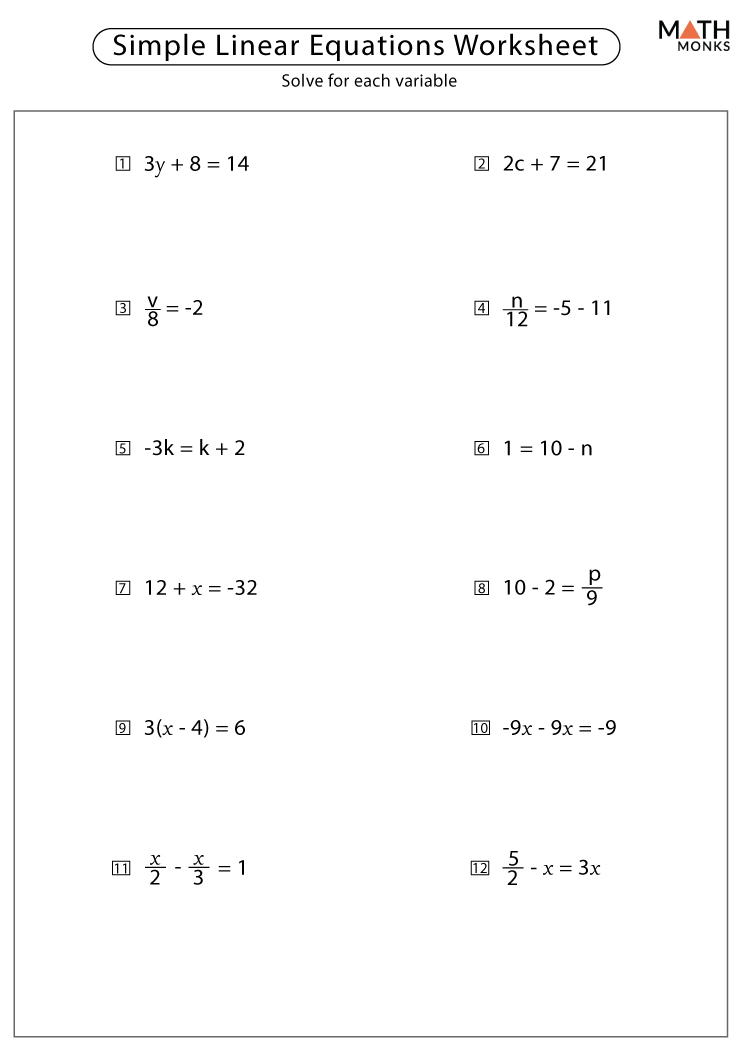
How to Use Our Linear Equations Worksheet

Getting Started
Download the worksheet and ensure you have a ruler, pen, or pencil. Here’s how to make the most of your practice session:
- Read the instructions: Understand what each question asks.
- Solve step by step: Don’t rush. Write each step clearly for better clarity.
- Check your answers: Using an answer key will provide immediate feedback, which is crucial for learning.
Worksheet Structure
The worksheet is designed with a variety of question types:
| Type of Problem | Description |
|---|---|
| Standard form equations | Solve for x or y in equations like ax + by = c |
| Word problems | Translate real-world scenarios into linear equations and solve. |
| Graphing lines | Identify or plot lines given their equation or intercept. |
| Parallel and perpendicular lines | Explore relationships between line slopes. |
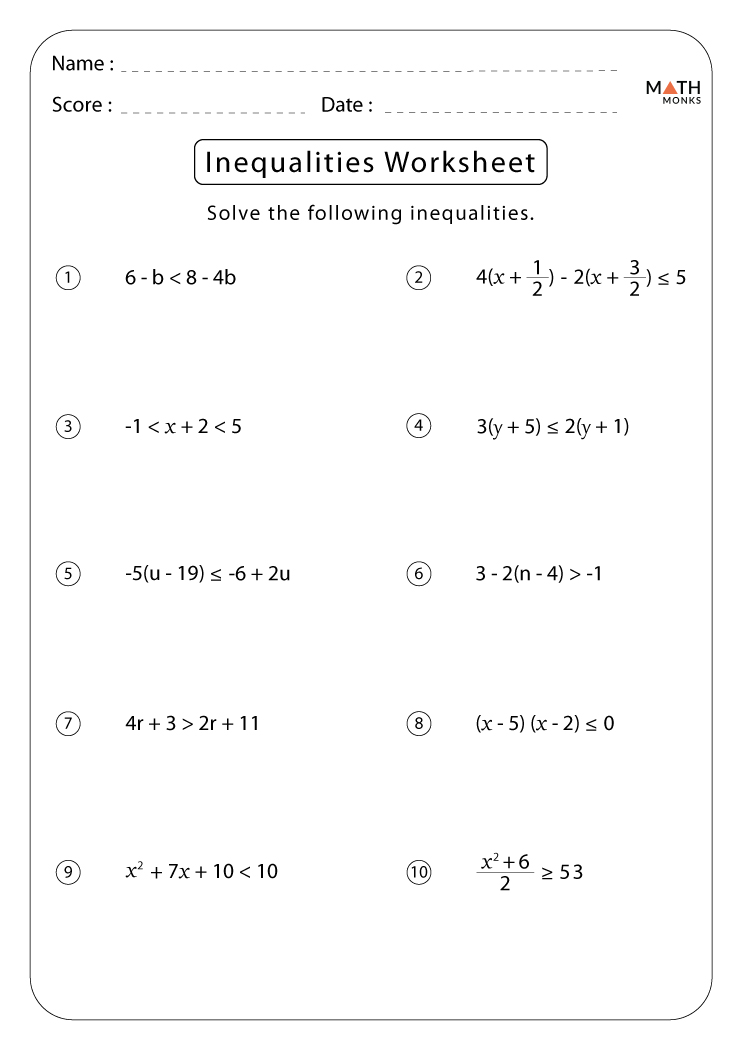
Tips for Effective Practice

- Focus on understanding: Each practice session should enhance your understanding of concepts, not just problem-solving speed.
- Use tools: Utilize graphing calculators or software to visualize equations, making it easier to grasp abstract concepts.
- Seek feedback: Discussing solutions with peers or a teacher can provide new insights.
- Repeat and review: Revisit past questions to reinforce your learning.
🧠 Note: Learning from mistakes is key. Analyze each incorrect answer to understand where you went wrong.
Final Thoughts

Embarking on the journey to master linear equations through our practice worksheet can significantly bolster your mathematical aptitude. The benefits are far-reaching; it’s not just about algebra but understanding how numbers, variables, and functions interact in the broader context of mathematics. With diligent practice, you’ll not only solve equations but begin to see the patterns and connections in mathematics that were previously hidden.
What is the difference between linear and non-linear equations?

+
Linear equations graph as straight lines, while non-linear equations result in curves or other shapes that aren’t straight lines. The highest power of any variable in a linear equation is one.
Can linear equations have more than one solution?

+
Linear equations typically have one unique solution for each variable, or they might have no solution at all (when the lines are parallel) or infinite solutions (when the lines are identical).
How can I solve a system of linear equations?

+
Systems of linear equations can be solved using methods like substitution, elimination, or graphing. Each method has its advantages depending on the specific equations involved.