5 Proven Methods for Solving Equations with Ease

In the world of mathematics, solving equations can sometimes feel like navigating through a dense fog. However, with the right techniques, what once seemed like an abstract puzzle becomes a series of logical steps. Here, we explore five proven methods to tackle equations, making this academic exercise a more straightforward and less daunting task.
Substitution Method

The Substitution Method is a direct approach for solving systems of equations. Here’s how it works:
- Identify one equation where isolating a variable is straightforward.
- Solve for that variable in terms of the others.
- Substitute the solved expression back into another equation.
- Repeat as necessary until all variables are solved for.
Here’s an example:
Suppose you have:
x + y = 5 (1) 2x + 3y = 12 (2)
From equation (1), we can isolate x:
x = 5 - y
Now, substitute this into equation (2):
2(5 - y) + 3y = 12 10 - 2y + 3y = 12 y = 2
Using y = 2 back into equation (1):
x + 2 = 5 x = 3
💡 Note: The Substitution Method works best when one of the equations has a variable with a coefficient of 1.
Graphing Method
The Graphing Method can visually show the solution of linear equations. Follow these steps:
- Graph each equation on the coordinate plane.
- Identify the point where the lines intersect.
- This intersection point represents the solution to the system of equations.
Here’s a simple example:
Consider:
y = 2x + 1 y = -x + 4
Plot both lines on a graph:

The intersection point at (1, 3) means x = 1 and y = 3.
🔎 Note: Graphing can be less precise than algebraic methods, but it's excellent for visualizing how equations relate to each other.
Linear Combination (Addition or Elimination Method)

This method involves combining equations in such a way that one variable cancels out:
- Ensure one variable has the same or opposite coefficients.
- Add or subtract the equations to eliminate one variable.
- Solve for the remaining variable.
- Substitute back to find the other variable.
Example:
2x + 3y = 12 3x - 3y = 6
Adding these:
(2x + 3y) + (3x - 3y) = 12 + 6 5x = 18 x = 18⁄5
Substitute x = 18⁄5 into either equation to find y.
Matrix Method
The Matrix Method is ideal for systems with many variables. Here’s the process:
- Write the system of equations in matrix form (A*X = B).
- Calculate the inverse of matrix A.
- Multiply both sides by the inverse to isolate X (X = A-1*B).
Here’s an example using the previous system:
[2 3 | 12] [3 -3| 6]
Matrix A =
[2 3] [3 -3]
To solve, multiply by the inverse:
X = A-1*B
X = [0.6 -0.6 | 2.4]
[-0.2 0.4 | 0.8]
X = [3⁄5 | 4⁄5]
🔧 Note: Learning matrix operations takes practice but can save time when solving complex systems.
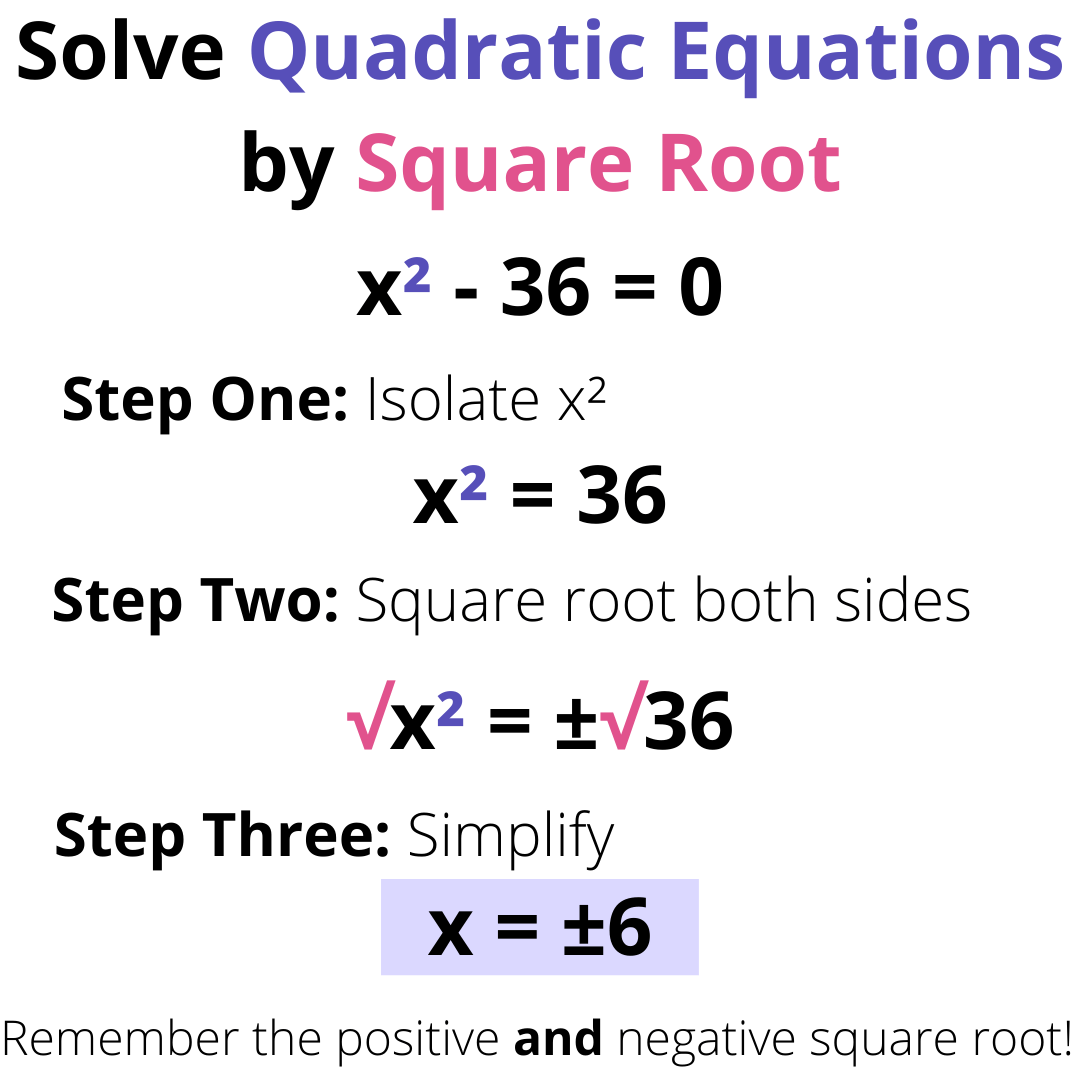
Quadratic Formula Method

For quadratic equations, the Quadratic Formula provides a straightforward approach:
The formula is:
x = (-b ± √(b² - 4ac)) / (2a)
Where a, b, and c come from the standard form of a quadratic equation, ax² + bx + c = 0.
Let’s use:
2x² + 5x - 3 = 0
Using the formula:
x = (-5 ± √(25 - 42(-3))) / (2*2) x = (-5 ± √49) / 4 x = (-5 ± 7) / 4
Thus:
x = 1⁄2 or x = -3
Understanding these methods equips you with a variety of tools for solving equations, each with its own advantages. The Substitution and Linear Combination Methods are perfect for basic systems, while the Matrix Method offers a more systematic approach for complex systems. The Graphing Method visually demonstrates solutions, and the Quadratic Formula provides a reliable solution for polynomial equations of degree two. These techniques not only facilitate solving equations but also enhance your overall mathematical thinking and problem-solving skills.
When should I use the substitution method over other methods?

+
Use the Substitution Method when one equation has a variable with a coefficient of 1 or when there’s an obvious way to isolate a variable easily.
Can I use the graphing method for non-linear equations?

+
Yes, graphing can be used for non-linear equations, but it might require graphing tools that can handle complex curves or surfaces. Software like Desmos or GeoGebra can be helpful.
Is the Matrix Method better for all systems of equations?

+
The Matrix Method is particularly efficient for systems with many variables due to its algorithmic approach, but for simple systems, other methods might be quicker and more straightforward.
What makes the Quadratic Formula so popular in solving quadratics?

+
Its popularity stems from its ability to provide exact solutions for any quadratic equation directly, without the need for factoring or completing the square.
Are there any pitfalls to avoid when using the Linear Combination Method?

+
Avoid mistakes in arithmetic when adding or subtracting equations, and ensure you accurately transfer coefficients. Also, be cautious with sign errors when combining equations.



