5-Step Guide to Mastering Multi-Step Equations

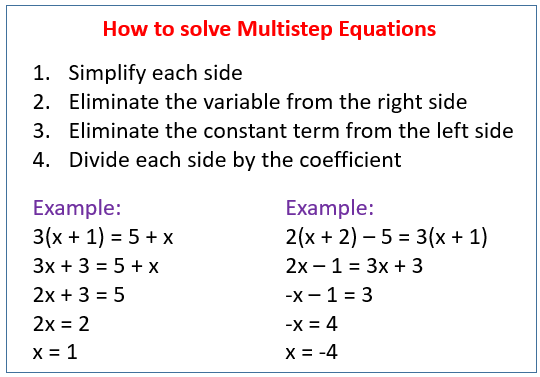
Embarking on a journey to master multi-step equations is a significant milestone for students in mathematics. Multi-step equations are not just about finding a solution but understanding a structured approach to problem-solving. They can be daunting, but with the right techniques, they transform into engaging puzzles waiting to be solved. Here's how you can excel in solving multi-step equations with a methodical, five-step approach.
Step 1: Understanding the Equation

Before diving into solving, it’s imperative to dissect the equation to comprehend what you’re dealing with. Multi-step equations contain variables, constants, and various operations. Here’s what to look for:
- Variables: Identify the unknown quantities, typically represented by letters like x, y, or z.
- Constants: Notice the numbers in the equation; these are your known values.
- Operations: Spot addition, subtraction, multiplication, division, exponents, or roots.
- Combined Terms: Look for terms grouped with parentheses or implied grouping.
📝 Note: Understanding the structure is key. Break the equation into manageable parts to reduce complexity.
Step 2: Clearing Parentheses and Grouping

Next, you’ll need to simplify your equation by dealing with any parentheses or grouped terms. Use the distributive property to distribute multiplication or division across parentheses. Here’s a simplified example:
2(x + 3) - 5 = 7
Distribute the 2 across the parentheses:
2*x + 2*3 - 5 = 7
Simplify:
2x + 6 - 5 = 7
Combine like terms:
2x + 1 = 7
🔧 Note: Always start by clearing any grouping symbols to reduce the equation’s complexity.
Step 3: Isolate the Variable

Your aim is to have the variable on one side of the equation and constants on the other. Here’s how:
- If there are constants on the variable’s side, move them by performing inverse operations.
- Ensure to perform the same operation on both sides of the equation to maintain balance.
Continuing from the example above:
2x + 1 = 7
Subtract 1 from both sides:
2x = 6
Step 4: Solving for the Variable

With the variable isolated, proceed to find its value:
- Divide both sides by the coefficient (the number before the variable).
From the example:
2x = 6
Divide by 2:
x = 3
Step 5: Verification and Refinement

Solving multi-step equations isn’t complete without verifying the solution. Here’s how to ensure accuracy:
- Substitute the found value back into the original equation.
- Check if both sides are equal; if not, revisit your steps for potential errors.
Using our example:
2(3) + 3 - 5 = 7
Which simplifies to:
6 + 3 - 5 = 7
Finally:
4 = 4
Your solution is correct.
🔍 Note: Verifying your solution is crucial. It ensures not only the accuracy of your work but also reinforces your understanding of the process.
Throughout your journey in mastering multi-step equations, remember that practice is your strongest ally. Regularly working through these equations will sharpen your skills, increase your speed, and deepen your understanding of algebra. To aid in your learning, consider exploring real-life applications of algebra or tackling complex equations from various fields, which can make this journey not just educational but also highly engaging.
How do I know if I have correctly distributed terms within parentheses?

+
Check that each term inside the parentheses has been multiplied or divided by the factor outside the parentheses. Sum or subtract these results appropriately.
Why do I need to perform the same operation on both sides of the equation?

+
To maintain the equality, each operation performed must be replicated on both sides to ensure the equation stays balanced.
Can I perform operations in any order when solving?
+
Generally, you should follow the order of operations (PEMDAS) when simplifying an equation but adapt to isolate the variable first when solving for a solution.