Solving by Elimination: Easy Math Worksheet Tips

When it comes to excelling in math, whether you're a student tackling high school algebra or an adult brushing up on basic arithmetic, understanding how to solve problems efficiently is key. One of the most versatile strategies you can master is solving by elimination. This method, while straightforward, can significantly streamline your problem-solving approach, making it an essential tool in your mathematical toolkit. Here's an in-depth look at how you can leverage this technique on math worksheets to boost your performance.
The Basics of Solving by Elimination

Elimination in mathematics involves removing variables or possibilities from an equation or a set of equations to isolate and solve for the unknown. This technique is particularly useful in systems of equations, where you aim to eliminate one variable to simplify the problem.
- Identify the Variables: The first step is to know what you are trying to eliminate. Typically, in math worksheets, you'll be looking at systems with two or three variables.
- Manipulate Equations: Make the coefficients of one of the variables in both equations match. This might involve multiplying or dividing both sides of an equation by a number.
- Eliminate a Variable: Add or subtract the manipulated equations to eliminate one variable. Now, you can solve for the remaining variable.
- Substitute and Solve: Once you've found one variable, substitute it back into one of the original equations to find the other variable(s).
📝 Note: When solving systems of equations, make sure to check if your solutions satisfy all original equations.
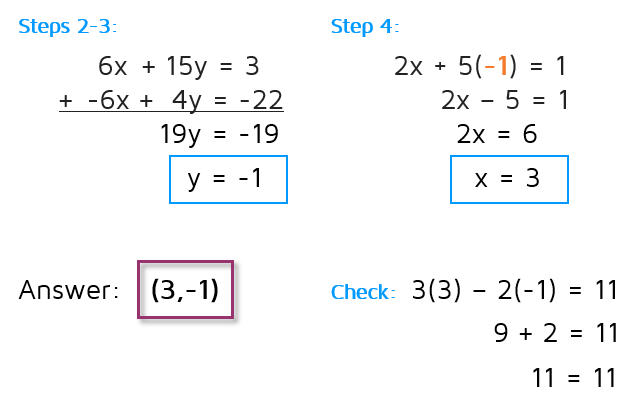
Steps for Solving Equations by Elimination

Let’s dive deeper into the step-by-step process:
- Align Like Terms: Ensure that like terms in both equations are on the same side.
- Make Coefficients Equal: If one variable's coefficients are not opposite or equal, multiply one or both equations to make them so.
- Add or Subtract to Eliminate: Perform addition or subtraction to cancel out one variable.
- Find One Variable: Now, solve for one of the variables left.
- Substitute and Solve Further: Substitute the known value into either of the original or simplified equations to find the other variable(s).
- Verify Solutions: Always check your work by substituting the solutions back into all original equations to ensure accuracy.
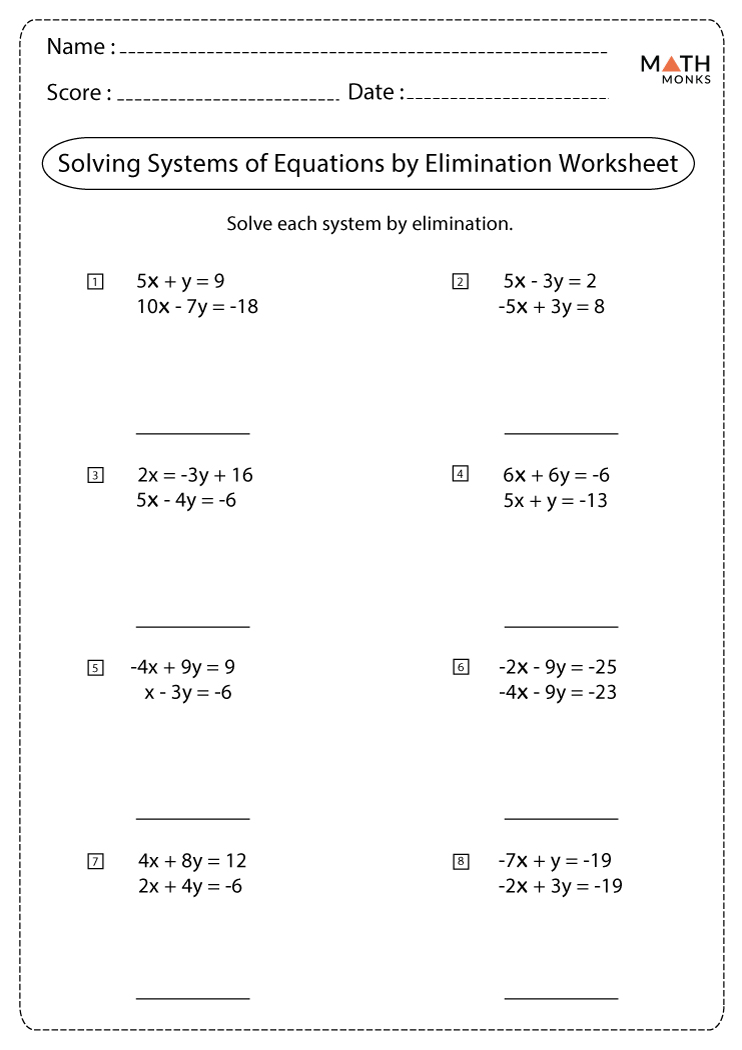
Practical Tips for Worksheets

Applying these steps effectively to math worksheets involves some additional considerations:
- Choose the Easiest Variable to Eliminate: Look for variables where the coefficients are already equal or very close, to minimize manipulation.
- Be Careful with Signs: Remember that adding a negative is the same as subtracting, which can be particularly handy when dealing with elimination.
- Use Visual Aids: Sometimes, underlining or highlighting the terms you're focusing on can help maintain concentration and reduce errors.
- Practice Simplification: The simpler you can make each equation, the easier the elimination process will be.
Here's an example of how this might look in a table format:
| Equation | Step 1 | Step 2 | Step 3 | Solution |
|---|---|---|---|---|
| 2x + 3y = 7 | Multiply by 2 | 4x + 6y = 14 | Subtract from next equation | 4x + 6y - (3x + 6y = 15) = x = -1 |
| 3x + 6y = 15 | No change | 3x + 6y = 15 | y = 3 |

🔎 Note: Remember that when multiplying an equation by a constant, all terms must be multiplied by that constant.
Common Pitfalls and How to Avoid Them
Even experienced math solvers can fall into traps when using elimination. Here are common pitfalls:
- Losing Track of Signs: Always ensure you are correctly adding or subtracting equations. A small sign error can lead to incorrect solutions.
- Misaligning Equations: Make sure when aligning terms that you're adding or subtracting like terms.
- Not Checking Solutions: Verify your work by plugging solutions back into the original equations to avoid false solutions due to errors in elimination or substitution.
With practice, these common mistakes become less frequent, but always keep them in mind to ensure accuracy.
To summarize, solving by elimination is an incredibly useful method that simplifies complex mathematical problems by systematically removing variables. Here are the key points:
- It involves aligning, manipulating, and eliminating variables from equations.
- The technique is particularly effective for systems of linear equations.
- It requires careful attention to signs and equation manipulation.
- Always verify your solutions to ensure they fit the original equations.
By mastering this approach, you're not only solving math problems more efficiently but also honing your problem-solving skills in general. Whether you're preparing for an exam or solving real-world problems, elimination can make the process more manageable.
What if my variables can’t be easily eliminated?

+
If the variables aren’t easily eliminated, you might need to adjust your strategy. Consider using substitution instead, or multiply one equation by a number that will make elimination possible. Remember, sometimes you’ll need to multiply both equations to get the coefficients to match.
Can elimination be used in other fields besides algebra?

+
Yes, the principle of elimination can be applied in various contexts. For example, in programming to remove redundant code, in scientific simulations to simplify models, or even in daily life when planning to exclude options that don’t meet specific criteria.
Is there any software that can help with the elimination method?
+
Yes, tools like MATLAB, GeoGebra, and some graphing calculators have features to solve systems of equations using the elimination method. They can perform the tedious calculations, helping you focus on the strategy and interpretation of results.



