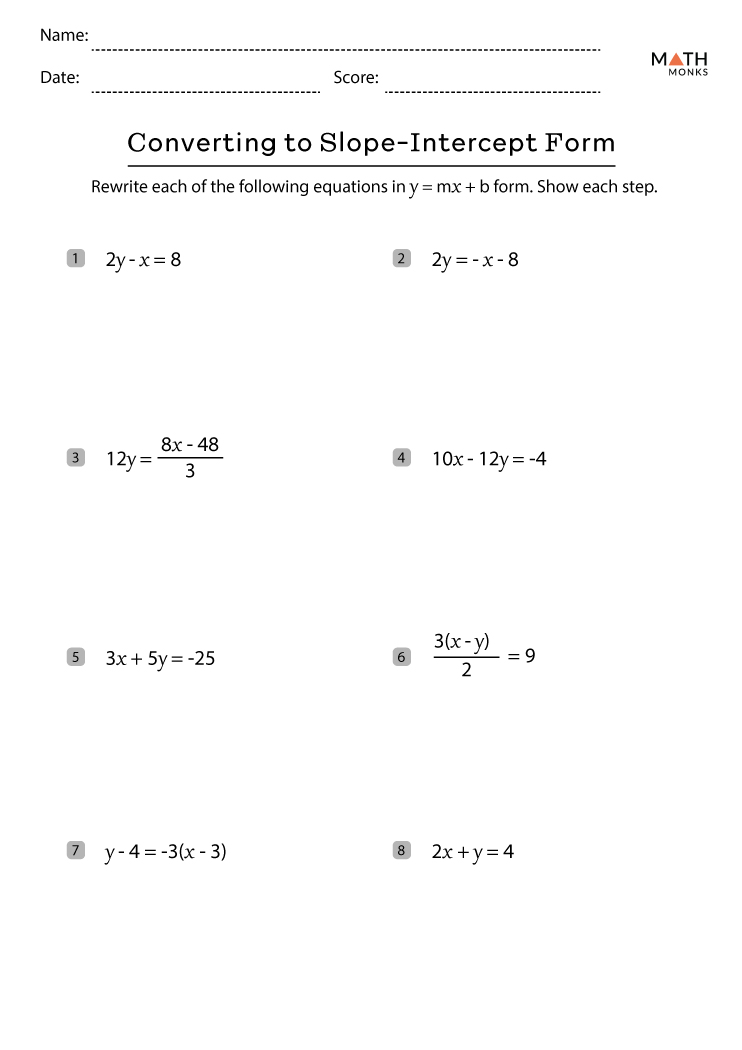
Slope Intercept Form: 5 Worksheets with Answer Keys

Exploring the Slope Intercept Form: Enhancing Understanding with Worksheets

The slope intercept form is a fundamental concept in algebra that simplifies the equation of a straight line into a readable and easily understandable format. Represented as y = mx + b, this equation helps students visualize and analyze the properties of lines. Here, we provide 5 worksheets complete with answer keys to solidify understanding of this concept.
Why Use the Slope Intercept Form?

Understanding the slope-intercept form is crucial for several reasons:
- Linear Equations: It simplifies solving linear equations by directly identifying the slope and y-intercept.
- Graphing: It offers an intuitive way to graph linear functions with minimal computation.
- Real-Life Applications: Helps in understanding trends in real-life scenarios like finance, physics, and engineering.
Worksheet 1: Basic Understanding

This worksheet introduces students to the basic concept:
| Question | Answer Key |
|---|---|
| Identify m and b in y = 3x + 4 | m = 3, b = 4 |
| Graph y = -2x + 5 | (Sloping downward from y-intercept 5) |

🔍 Note: This worksheet covers the basics to ensure students understand the terms slope and y-intercept.
Worksheet 2: Finding Slope and Y-Intercept

Students practice identifying the slope and y-intercept from various equations:
- Find the slope and y-intercept for y = -4x - 1.
- Convert 2x - y = 7 into slope-intercept form.
Worksheet 3: Writing Equations from Points

This worksheet focuses on writing equations given points:
- Write the equation of the line passing through (3, 4) with a slope of -2.
- Find the equation of the line with points (1, 5) and (4, 1).
📏 Note: Here, students combine algebra and geometry to derive equations from given points.
Worksheet 4: Graphing Lines

Students graph lines using the slope-intercept form:
- Graph y = -3/2x + 2.
- Sketch y = x - 5.
Worksheet 5: Complex Problems

This final worksheet challenges students with more complex problems:
- Given points (0,5) and (2,1), find the slope, y-intercept, and equation.
- Write an equation for a line parallel to y = -x + 6 that passes through (5, 0).
Wrapping Up the Journey Through Slope Intercept

These five worksheets are designed to provide a comprehensive understanding of the slope-intercept form of a line. They start with basic identification and progress to more complex problem-solving, ensuring that students not only grasp the concept but also apply it in varied contexts. Here's what we've covered:
- How to read and interpret the components of the slope-intercept form.
- Skills to identify the slope and y-intercept from any given linear equation.
- Constructing equations from graphical information or given data points.
- Graphing lines using both algebraic and graphical methods.
- Practical application through word problems and real-life scenarios.
In mastering these skills, students enhance their algebraic knowledge, graphical interpretation, and problem-solving capabilities, making them well-prepared for higher-level math courses and everyday mathematical challenges.
How do I determine the slope when given two points?
+
To find the slope between two points (x1, y1) and (x2, y2), use the formula: slope = (y2 - y1) / (x2 - x1).
Can the slope-intercept form be used for any line?

+
Yes, any straight line can be expressed in the slope-intercept form, though some might require conversion from other forms like point-slope or standard form.
What does the y-intercept tell us about the line?

+
The y-intercept indicates where the line crosses the y-axis. It’s the value of y when x is zero in the equation y = mx + b.
Why is the slope important in real life?
+Slope represents the rate of change or trend. In real life, this could be the steepness of a hill, the rate at which temperature is changing, or how fast a population is growing.