Simplify Radicals with Ease
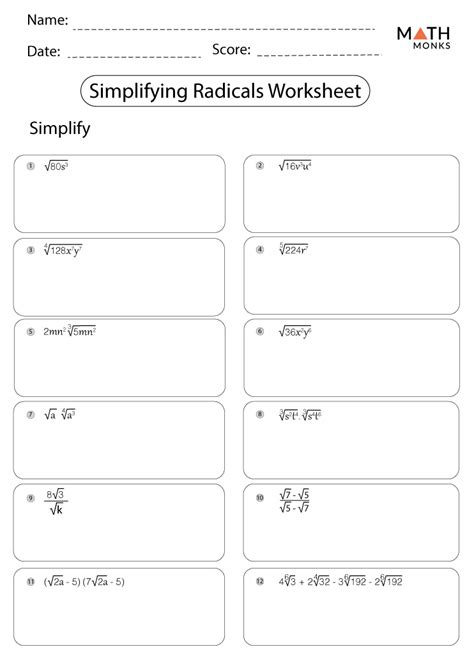
Simplifying Radicals: A Comprehensive Guide
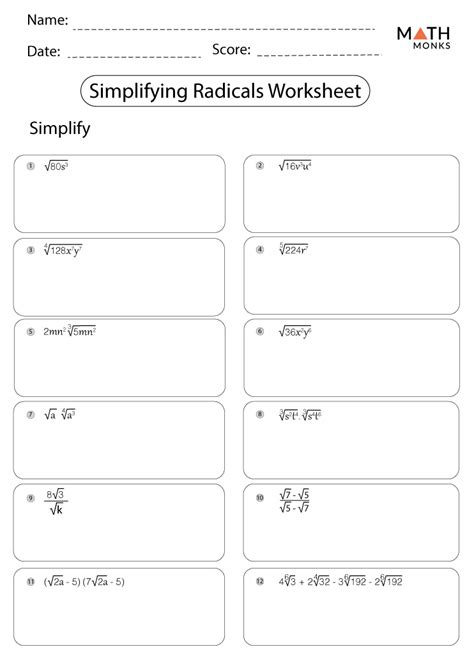
Radicals are an essential part of mathematics, and simplifying them is a crucial skill that every student should master. In this article, we will provide a step-by-step guide on how to simplify radicals with ease.
What are Radicals?
Radicals are mathematical expressions that contain a root symbol (√) followed by a number or expression. The root symbol indicates that we are looking for a number that, when multiplied by itself, gives us the original value. For example, √16 = 4 because 4 × 4 = 16.
Types of Radicals
There are two types of radicals: square roots and cube roots. Square roots are the most common type of radical and are represented by the symbol √. Cube roots are represented by the symbol ∛.
Simplifying Radicals
Simplifying radicals involves factoring out perfect squares or perfect cubes from the radicand (the number or expression inside the radical). Here are the steps to simplify radicals:
- Factor out perfect squares: Look for perfect squares that can be factored out of the radicand. For example, √16 = √(4 × 4) = 4√1 = 4.
- Factor out perfect cubes: Look for perfect cubes that can be factored out of the radicand. For example, ∛27 = ∛(3 × 3 × 3) = 3∛1 = 3.
- Simplify the radicand: If there are any remaining numbers or expressions inside the radical, simplify them.
Examples
Here are some examples of simplifying radicals:
- √24 = √(4 × 6) = 2√6
- √48 = √(16 × 3) = 4√3
- ∛64 = ∛(4 × 4 × 4) = 4∛1 = 4
- ∛125 = ∛(5 × 5 × 5) = 5∛1 = 5
Tips and Tricks
Here are some tips and tricks to help you simplify radicals:
- Look for perfect squares and perfect cubes: Always look for perfect squares and perfect cubes that can be factored out of the radicand.
- Use the distributive property: Use the distributive property to factor out numbers or expressions from the radicand.
- Simplify the radicand: Always simplify the radicand by factoring out numbers or expressions.
📝 Note: When simplifying radicals, always look for perfect squares and perfect cubes that can be factored out of the radicand.
Common Mistakes
Here are some common mistakes to avoid when simplifying radicals:
- Not factoring out perfect squares or perfect cubes: Make sure to factor out perfect squares or perfect cubes from the radicand.
- Not simplifying the radicand: Always simplify the radicand by factoring out numbers or expressions.
🚫 Note: Be careful not to make these common mistakes when simplifying radicals.
Conclusion
Simplifying radicals is a crucial skill that every student should master. By following the steps outlined in this article, you can simplify radicals with ease. Remember to look for perfect squares and perfect cubes, use the distributive property, and simplify the radicand.
What is the difference between a square root and a cube root?

+
A square root is a radical that represents a number that, when multiplied by itself, gives us the original value. A cube root is a radical that represents a number that, when multiplied by itself twice, gives us the original value.
How do I simplify a radical with a coefficient?

+
To simplify a radical with a coefficient, factor out the perfect squares or perfect cubes from the radicand, and then multiply the coefficient by the simplified radical.
Can I simplify a radical with a variable?
+
Yes, you can simplify a radical with a variable by factoring out perfect squares or perfect cubes from the radicand, and then simplifying the remaining expression.
Related Terms:
- Simplifying radicals worksheet pdf
- Simplifying radicals worksheet answer key
- Simplifying Radicals Worksheet Kuta



