7 Tricks to Simplify Polynomials Easily

Polynomials are an essential part of algebra, often presenting complexities that can be overwhelming for both students and professionals. However, by mastering a few strategic tricks, simplifying these mathematical expressions can become much more manageable. This blog post will delve into 7 Tricks to Simplify Polynomials Easily, providing practical steps and examples to streamline your approach to polynomial simplification.

1. Use the Distributive Property

The distributive property states that a(b + c) = ab + ac. This rule allows us to distribute factors to each term inside a parenthesis:

• Expand the expression: (2x + 3)(5x - 1)
  • Apply the distributive property:
    • 2x(5x) + 2x(-1) + 3(5x) + 3(-1)
  • Simplify to: 10x² - 2x + 15x - 3
  • Combine like terms to get: 10x² + 13x - 3

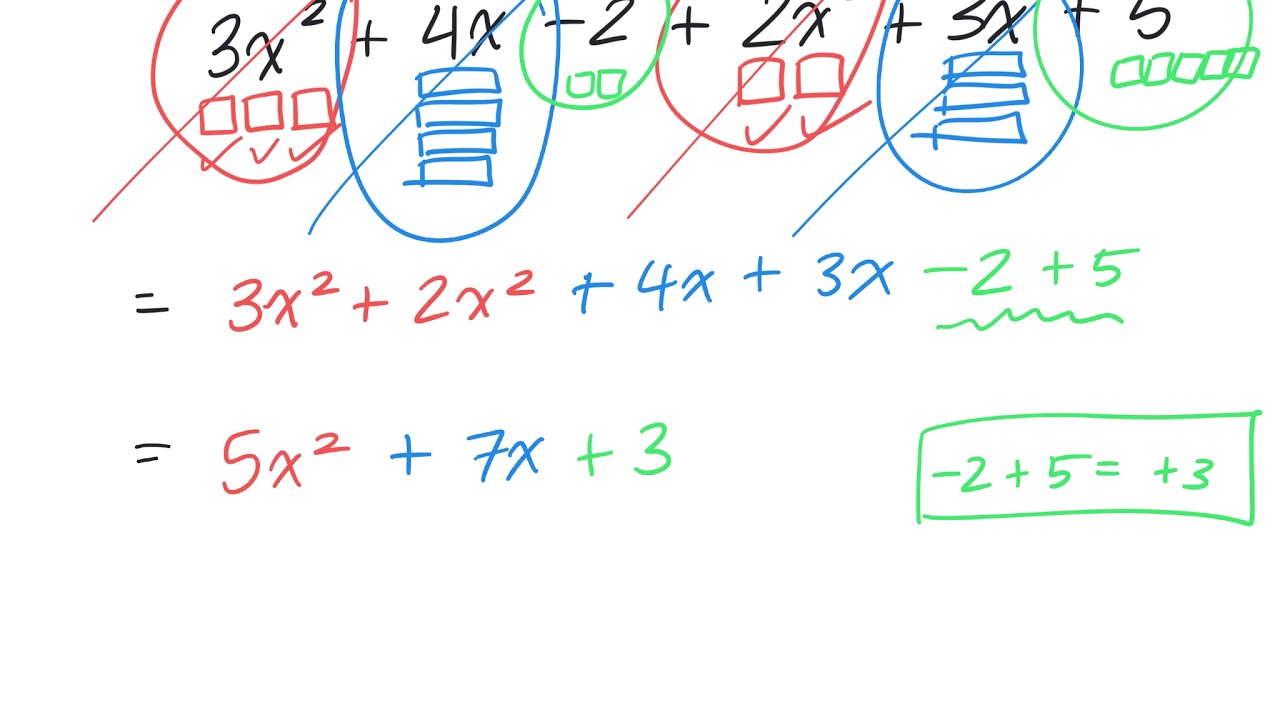
2. Combine Like Terms

Like terms are those with the same variable part and degree. Here’s how to combine them:

• Identify and sum up the like terms in an expression:
  • 3x² + 4x² - 2x + 5x - 6
  • Combine the x² terms: (3 + 4)x² = 7x²
  • Combine the x terms: (-2 + 5)x = 3x
  • Resulting in: 7x² + 3x - 6

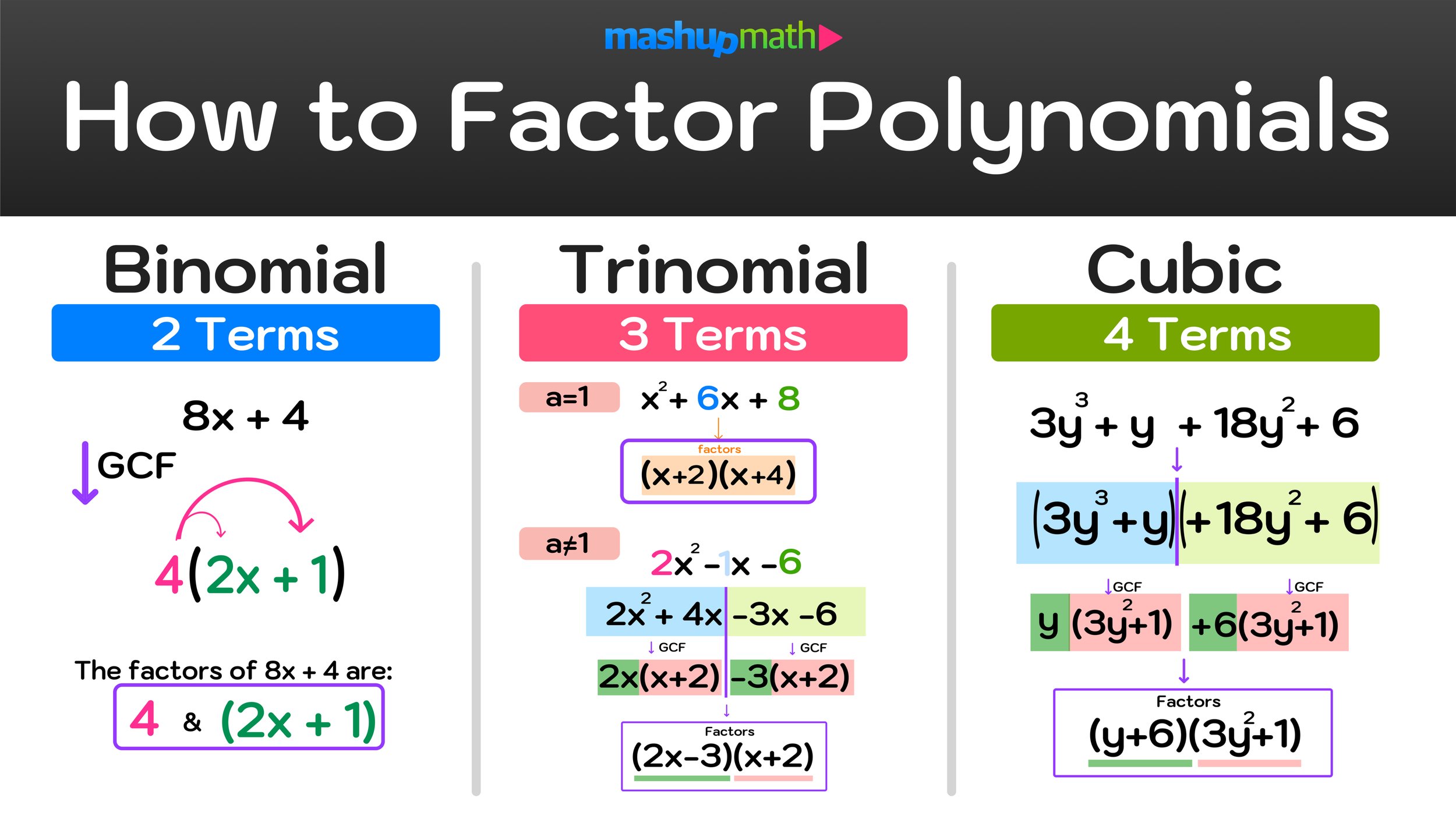
3. Factor Out Common Terms

Factoring out common terms can significantly simplify polynomial expressions:

• For example: 6x³ - 9x²
  • Factor out the common term ‘3x²’:
    • 3x²(2x - 3)

💡 Note: Factoring out common terms is particularly useful when dealing with polynomial equations to find roots.

4. Use Synthetic Division for Polynomial Division

Synthetic division provides a straightforward method to divide polynomials by a linear binomial:

• To divide 2x³ - 5x² + 4 by (x - 2):
  • Write down the coefficients of the polynomial and the root: 2, -5, 0, 4, and root 2.
  • Perform synthetic division:
    • Bring down 2
    • Multiply 2 by the root (2 * 2) to get 4, then add to the next coefficient (-5 + 4 = -1)
    • Multiply -1 by 2 to get -2, then add to the next coefficient (0 - 2 = -2)
    • Multiply -2 by 2 to get -4, then add to the last coefficient (4 - 4 = 0)
  • The quotient is 2x² - x - 2, and the remainder is 0.

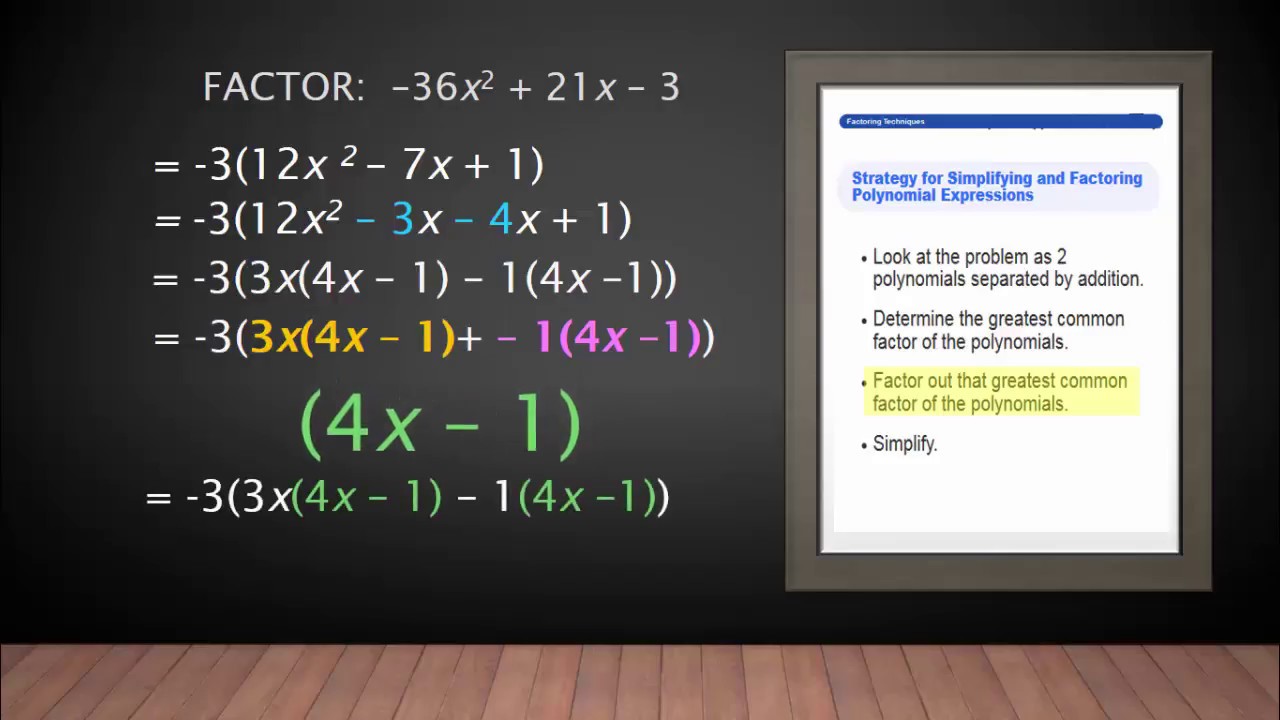
5. Simplify Using the Difference of Squares

The difference of squares formula allows you to simplify expressions in the form of a² - b²:

• Given 9x² - 1:
  • Recognize it as (3x)² - 1²
  • Apply the formula: (3x + 1)(3x - 1)

💡 Note: This trick is particularly useful when simplifying expressions where you can recognize perfect squares.

6. Group Terms for Factoring

Grouping terms can aid in factorization:

• Consider the polynomial x³ + 2x² - x - 2:
  • Group the terms in pairs: (x³ + 2x²) + (-x - 2)
  • Factor out common terms from each group:
    • x²(x + 2) - 1(x + 2)
  • Now, factor out the common binomial factor:
    • (x² - 1)(x + 2)
  • Further simplify by recognizing the difference of squares:
    • (x + 1)(x - 1)(x + 2)

7. Use Polynomial Long Division for More Complex Division

For polynomials that are not easily divisible by linear terms, polynomial long division can be used:

• To divide 4x⁴ - 3x³ - 30x² + 6x - 20 by 2x² + 3:
  • Follow the steps of polynomial long division:
    • Divide leading terms:
      • 4x⁴ / 2x² = 2x²
    • Multiply back by the divisor and subtract:
      • 2x²(2x² + 3) = 4x⁴ + 6x², subtract this from the dividend
    • Repeat with the new polynomial:
      • (-9x² + 6x - 20) / 2x² = -4.5
    • Continue the division until you reach a quotient and remainder of lower degree.

As we’ve journeyed through these seven tricks, you’ve learned various methods to simplify polynomials with ease. Each technique offers a unique approach to breaking down complex expressions into simpler, manageable parts. From using properties like the distributive law, combining like terms, to employing polynomial division methods, these strategies not only enhance your understanding but also improve your efficiency in algebraic problem-solving. By incorporating these techniques, you’ll be well-equipped to tackle more advanced polynomial problems and make your mathematical endeavors less daunting.

What is the difference between synthetic and long division for polynomials?

+

Synthetic division is used specifically when dividing by a linear binomial (ax + b). It’s quicker for single variable polynomials with no coefficients other than 1 in the divisor. Polynomial long division, on the other hand, is used for more complex polynomials or when the divisor has higher degrees or is not linear.

When should I use the difference of squares method?

+

The difference of squares method should be used when you have a polynomial expression that can be written as the subtraction of two squares. This is particularly useful for simplifying complex expressions or when solving quadratic equations.

Can factoring always simplify polynomial expressions?

+

While factoring is a powerful tool, not all polynomials can be factored with whole number coefficients into simpler forms. Sometimes, you might encounter irreducible polynomials that cannot be factored further over the integers or rational numbers.

Is there a systematic way to choose which trick to apply?

+

The choice of method depends on the structure of the polynomial. Here’s a basic approach:

• Combine like terms first as it simplifies the overall expression.
• Factor out common terms if there are coefficients or variables that appear in all terms.
• Look for patterns like the difference of squares or use polynomial division for complex divisions.