Simple Distributive Property Worksheet

Understanding the distributive property is fundamental in algebra and provides a deeper insight into how arithmetic operations interact. This foundational math concept allows us to distribute the multiplication across the addition or subtraction of numbers within parentheses, making complex calculations much simpler. Here’s how you can teach and practice this concept effectively using a simple worksheet that both simplifies the learning process and encourages the application of the distributive property in real-life scenarios.
Introduction to the Distributive Property

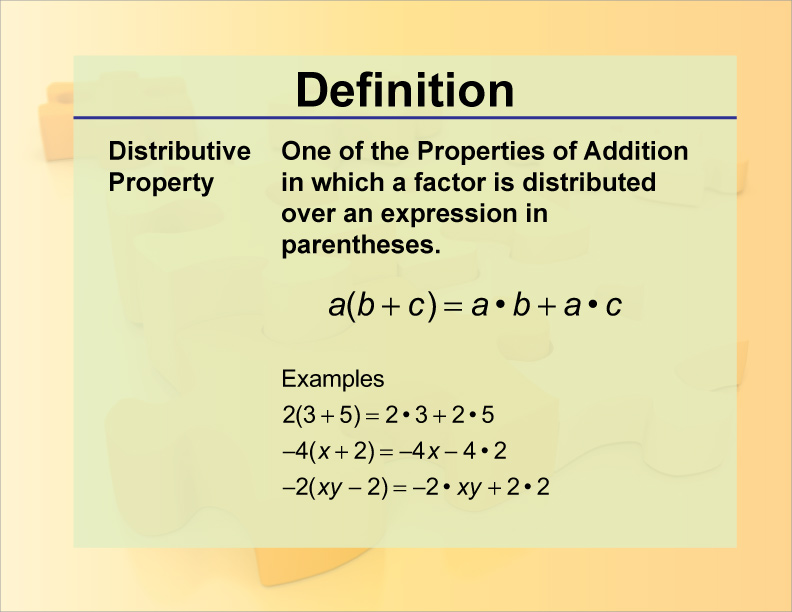
The distributive property states that for any real numbers a, b, and c:
a * (b + c) = (a * b) + (a * c) or a * (b - c) = (a * b) - (a * c).
This rule simplifies the process of multiplying a sum or difference by distributing the multiplication through the parentheses. Here’s how it looks in action:
- 4 * (2 + 3) = (4 * 2) + (4 * 3) = 8 + 12 = 20
- 5 * (7 - 3) = (5 * 7) - (5 * 3) = 35 - 15 = 20
Creating Your Distributive Property Worksheet

Let’s delve into how you can create an engaging and educational distributive property worksheet.
Step 1: Define Learning Objectives

Before you start, outline what you want students to achieve:
- Recognize and apply the distributive property.
- Simplify expressions using this property.
- Understand how it applies to both addition and subtraction.
Step 2: Begin with Examples

Start your worksheet with some straightforward examples to introduce the concept. Include a mix of simple numbers and real-life scenarios:
| Example | Worked Out |
|---|---|
| 3 * (4 + 5) | 3 * 4 + 3 * 5 = 12 + 15 = 27 |
| 6 * (10 - 2) | 6 * 10 - 6 * 2 = 60 - 12 = 48 |

Step 3: Guided Practice

Provide guided practice exercises where students fill in the blanks or solve for missing numbers:
- 3 * (5 + 2) = __ * 5 + __ * 2
- 5 * (8 - __) = 5 * 8 - __ * __
Step 4: Independent Practice

Introduce problems that encourage students to use the distributive property independently. Mix in some algebraic expressions:
- a * (b + c) =
- (x + y) * z =
- Simplify: 2(x + 5) - x + 3
Step 5: Application in Real-World Scenarios

Show how the distributive property can be applied to everyday situations:
- If a store sells cookies in packs of 3 for 5 each, how much would 6 packs cost?</li> <li>Imagine you have 10 bills of 20 and $10 each. How much total money do you have?
✨ Note: Real-world applications help students relate mathematical concepts to their daily lives, making the learning process more engaging and memorable.
Step 6: Review and Wrap Up

Include a section where students can reflect on what they’ve learned:
- How does understanding the distributive property help with problem-solving?
- Can you think of other situations where this property could be useful?
Wrapping Up
By delving into the distributive property through this simple yet effective worksheet, you’ve equipped students with a tool to simplify their mathematical journey. This property isn’t just a classroom trick; it’s a cornerstone of algebraic manipulation and critical thinking. It fosters a clearer understanding of how numbers interact, simplifies complex calculations, and is a precursor to understanding more advanced mathematical concepts like factoring and solving equations.
Engaging with real-life scenarios, guided practice, and an in-depth understanding of this rule ensures students can apply it confidently in various mathematical contexts. It’s more than a memory aid; it’s a conceptual tool that, once mastered, will support them throughout their mathematical education and beyond.
What is the purpose of the distributive property in mathematics?

+
The distributive property simplifies arithmetic operations by allowing multiplication to be distributed across addition or subtraction, making complex expressions easier to solve.
How can I teach the distributive property to young learners?

+
Using visual aids, real-world examples, and interactive activities like drawing arrays or using manipulatives can help younger students grasp this concept effectively.
Can the distributive property be used in subtraction as well as addition?

+
Yes, the distributive property applies to both addition and subtraction. For example, a * (b - c) = (a * b) - (a * c).



