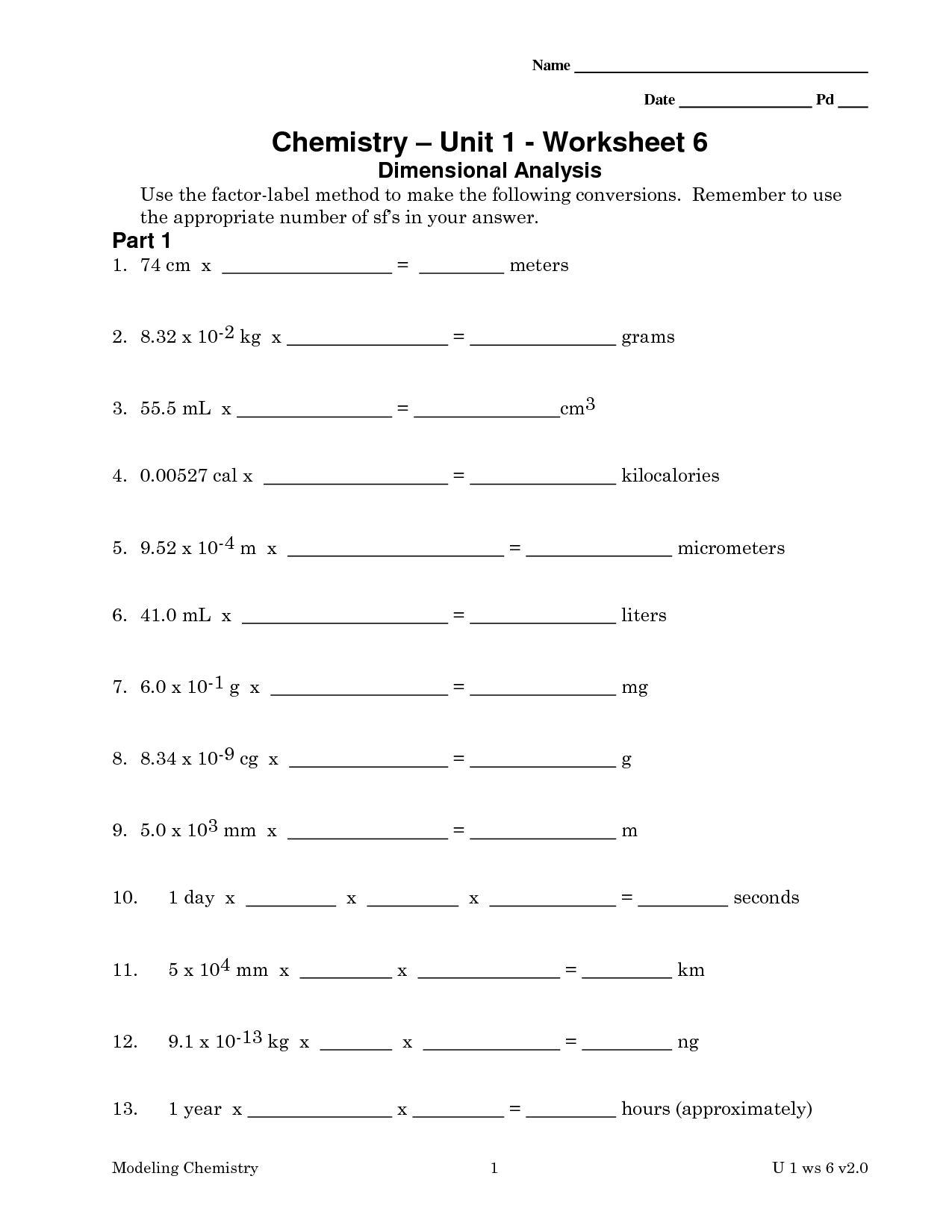
Scientific Notation Made Simple: Conversion Worksheet

If you've ever delved into scientific notation, you're likely aware it serves as a shorthand way to write very large or very small numbers. Whether it's for academic pursuits, scientific research, or even financial calculations, understanding scientific notation is key. Let's make the process of converting numbers to and from scientific notation straightforward and efficient with a handy worksheet to guide us through.
What is Scientific Notation?

Scientific notation is a mathematical expression used to represent numbers, especially those with many zeros or decimals. It’s written as a product of two factors:
- A decimal number between 1 and 10 (like 3.14 or 6.02)
- A power of 10 (like 106 or 10-3)
Here’s how it works:
- Move the decimal point to create a number between 1 and 10.
- Count the number of places you’ve moved the decimal; this count will be the exponent of 10.
- If you moved the decimal to the left, the exponent will be positive; to the right, it will be negative.
The Conversion Worksheet

Here’s a basic scientific notation conversion worksheet to help you practice:
| Standard Form | Scientific Notation |
|---|---|
| 3,200,000 | 3.2 × 106 |
| 0.000000047 | 4.7 × 10-8 |
| 5,420,000,000 | 5.42 × 109 |
| 0.00000734 | 7.34 × 10-6 |
| 780,000,000 | 7.8 × 108 |

Converting from Standard Form to Scientific Notation

To convert numbers from standard form to scientific notation:
- Determine the number’s starting point: if the number is very large, move the decimal to the left; if it’s very small, move it to the right.
- Move the decimal point so that you get a number between 1 and 10.
- Count the number of moves:
- Each move to the left adds 1 to the exponent.
- Each move to the right subtracts 1 from the exponent.
- Place the exponent on the 10.
Converting from Scientific Notation to Standard Form

The reverse process is equally straightforward:
- Look at the exponent:
- If it’s positive, move the decimal to the right.
- If it’s negative, move it to the left.
- Move the decimal the number of places indicated by the exponent.
- Fill in any necessary zeros to reach the original number.
Applications of Scientific Notation

Scientific notation isn’t just a tool for academics; it has practical applications in various fields:
- Astronomy: Distances between celestial bodies are measured in extremely large numbers, making scientific notation essential.
- Chemistry and Physics: For dealing with Avogadro’s number (6.02 × 1023) or Planck’s constant (6.626 × 10-34 Js).
- Finance: In international financial markets, large sums of currency are often referred to in terms of billions or trillions.
- Computing: Memory sizes are often expressed in scientific notation, like 16 GB (1.6 × 1010 bytes).
To make learning more interactive, here's an example exercise:
Exercise:

- Convert the following standard form numbers to scientific notation:
- 654,321
- 0.000009012
- 345,678,901
💡 Note: Always count the moves of the decimal point, even if the number ends with zeros.
In summary, scientific notation allows us to work with numbers in a more compact and manageable form. From the tiniest particles to the vast expanse of the universe, this mathematical shorthand enables precise communication across various scientific disciplines. The worksheet provided above is just a starting point to practice these conversions, which become second nature with practice. Continue to explore how numbers in different scientific contexts can be represented more easily and accurately using this notation, enhancing both your understanding and communication skills in science-related fields.
Why is scientific notation used?

+
Scientific notation is used to represent very large or very small numbers in a concise way, making calculations easier and more manageable.
What does the exponent represent in scientific notation?

+
The exponent indicates how many times the base (10) is multiplied by itself. It tells you how many places to move the decimal point to return to the original number.
Can I use scientific notation in everyday life?

+
Yes, although more commonly used in science and engineering, it can also simplify large numbers in finance, IT, and even when discussing population or economic statistics.
How do I know whether to use a positive or negative exponent?

+
Move the decimal to the left for positive exponents (numbers greater than 1) and to the right for negative exponents (numbers less than 1).
What’s the significance of the base-10 system in scientific notation?

+
Base-10 (our decimal system) is universally used because it’s the most convenient for our base-10 counting system, and it’s intuitive due to our ten fingers.