5 Ways to Master Rounding Decimals
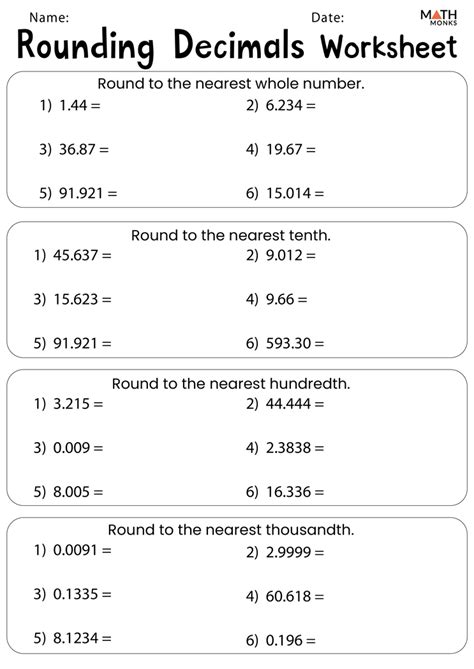
Rounding decimals is an essential skill in mathematics, finance, and science. It’s used to simplify complex calculations, reduce errors, and make data more readable. Mastering rounding decimals can be a challenge, but with the right techniques and practice, you can become proficient in no time. Here are five ways to help you master rounding decimals:
Understanding Rounding Rules

Before diving into the techniques, it’s essential to understand the basic rounding rules:
- Rounding up: If the digit to the right of the rounding place is greater than 5, round up.
- Rounding down: If the digit to the right of the rounding place is less than 5, round down.
- Rounding to the nearest: If the digit to the right of the rounding place is exactly 5, round up if the digit to the left is odd, and round down if the digit to the left is even.
Technique 1: Rounding to the Nearest Tenth

Rounding to the nearest tenth is a fundamental skill in mathematics. To round a decimal to the nearest tenth, look at the digit in the hundredths place (the second digit after the decimal point). If it’s greater than 5, round up; if it’s less than 5, round down.
Example:
- 3.457 rounded to the nearest tenth is 3.5
- 3.432 rounded to the nearest tenth is 3.4
Technique 2: Rounding to the Nearest Hundredth

Rounding to the nearest hundredth is similar to rounding to the nearest tenth. Look at the digit in the thousandths place (the third digit after the decimal point). If it’s greater than 5, round up; if it’s less than 5, round down.
Example:
- 3.4567 rounded to the nearest hundredth is 3.46
- 3.4312 rounded to the nearest hundredth is 3.43
Technique 3: Using Estimation

Estimation is a useful technique for rounding decimals. To estimate a decimal, look at the digits to the right of the rounding place and ask yourself:
- Is the digit greater than or less than 5?
- Is the digit close to 5 or far away from 5?
Example:
- 3.457 is close to 3.5, so it rounds to 3.5
- 3.432 is far away from 3.5, so it rounds to 3.4
Technique 4: Using Visual Aids

Visual aids like number lines and decimal charts can help you visualize the rounding process. A number line can help you see the relationship between decimals and whole numbers, while a decimal chart can help you compare decimals.
Example:
- Use a number line to visualize 3.457 and 3.5
- Use a decimal chart to compare 3.4567 and 3.46
Technique 5: Practicing with Real-World Examples

Practicing with real-world examples is an excellent way to master rounding decimals. Use everyday situations like measuring ingredients for a recipe or calculating change at the store.
Example:
- A recipe calls for 3.457 cups of flour. Round to the nearest tenth to get 3.5 cups.
- You receive 3.4567 in change. Round to the nearest hundredth to get 3.46.
| Decimal | Rounded to the Nearest Tenth | Rounded to the Nearest Hundredth |
|---|---|---|
| 3.457 | 3.5 | 3.46 |
| 3.432 | 3.4 | 3.43 |
| 3.4567 | 3.5 | 3.46 |
| 3.4312 | 3.4 | 3.43 |

💡 Note: Practice regularly to become proficient in rounding decimals. Start with simple examples and gradually move to more complex ones.
By mastering these five techniques, you’ll become more confident and proficient in rounding decimals. Remember to practice regularly and use real-world examples to reinforce your skills.
What is the purpose of rounding decimals?
+
Rounding decimals is used to simplify complex calculations, reduce errors, and make data more readable.
How do I round a decimal to the nearest tenth?

+
Look at the digit in the hundredths place (the second digit after the decimal point). If it’s greater than 5, round up; if it’s less than 5, round down.
Can I use visual aids to help with rounding decimals?

+
Yes, visual aids like number lines and decimal charts can help you visualize the rounding process and compare decimals.
Related Terms:
- Teacher synergy llc
- IXL Learning
- Khan Academy
- BrainPop
- Udacity
- Duolingo



