Master Rearranging Formulae: Free Printable Worksheet

Mastering the art of rearranging algebraic expressions is a vital skill for students delving into mathematics, particularly those studying physics, engineering, or any of the sciences. Not only does it allow for a deeper understanding of equations, but it also enables you to solve complex problems with greater ease. In this blog post, we'll explore the nuances of rearranging formulae, and we'll provide you with a free, printable worksheet to help you practice and refine these skills.
The Importance of Rearranging Formulae

Rearranging algebraic expressions is foundational for several reasons:
- Solving for Unknowns: It allows you to isolate variables to solve for unknowns in an equation.
- Real-World Applications: Equations in real-world problems often require variables to be expressed in different forms.
- Mathematical Fluency: It enhances your ability to manipulate expressions, a critical skill for more advanced mathematical concepts.
Before diving into the techniques, it’s helpful to understand why rearranging equations is so essential in various academic and professional fields.
Basic Techniques for Rearranging Equations

Transposition

Transposition involves moving terms from one side of the equal sign to the other. Here’s how it works:
- Choose the variable you wish to solve for.
- Move all terms not containing that variable to the opposite side of the equation by performing the inverse operation.
- Ensure you maintain the balance of the equation by changing the sign of the moved terms.
⚠️ Note: Always keep in mind the golden rule of algebra: what you do to one side of the equation must be done to the other.
Inverse Operations

Inverse operations are key to rearranging expressions:
- If a term is being added or subtracted, you would subtract or add it, respectively, to cancel it out.
- If a term is multiplied or divided by another, you multiply or divide by its reciprocal or the inverse factor.
Advanced Techniques

Square Root and Other Roots

Sometimes, you may need to isolate a variable raised to a power or under a root:
- To isolate a squared term, take the square root of both sides.
- If dealing with a cube or higher-order root, apply the appropriate inverse root to both sides.
Logarithms and Exponents

For logarithmic or exponential forms:
- Logarithms can help solve for an exponent by taking the log of both sides.
- To deal with exponents, apply logarithms to bring the exponent down.
Practice Problems

Here’s a collection of algebraic expressions for you to rearrange:
| Equation | Rearrange for |
|---|---|
| 2x + 3 = 5 | x |
| xy - 4y = 8 | x |
| a² + 3a = 24 | a |

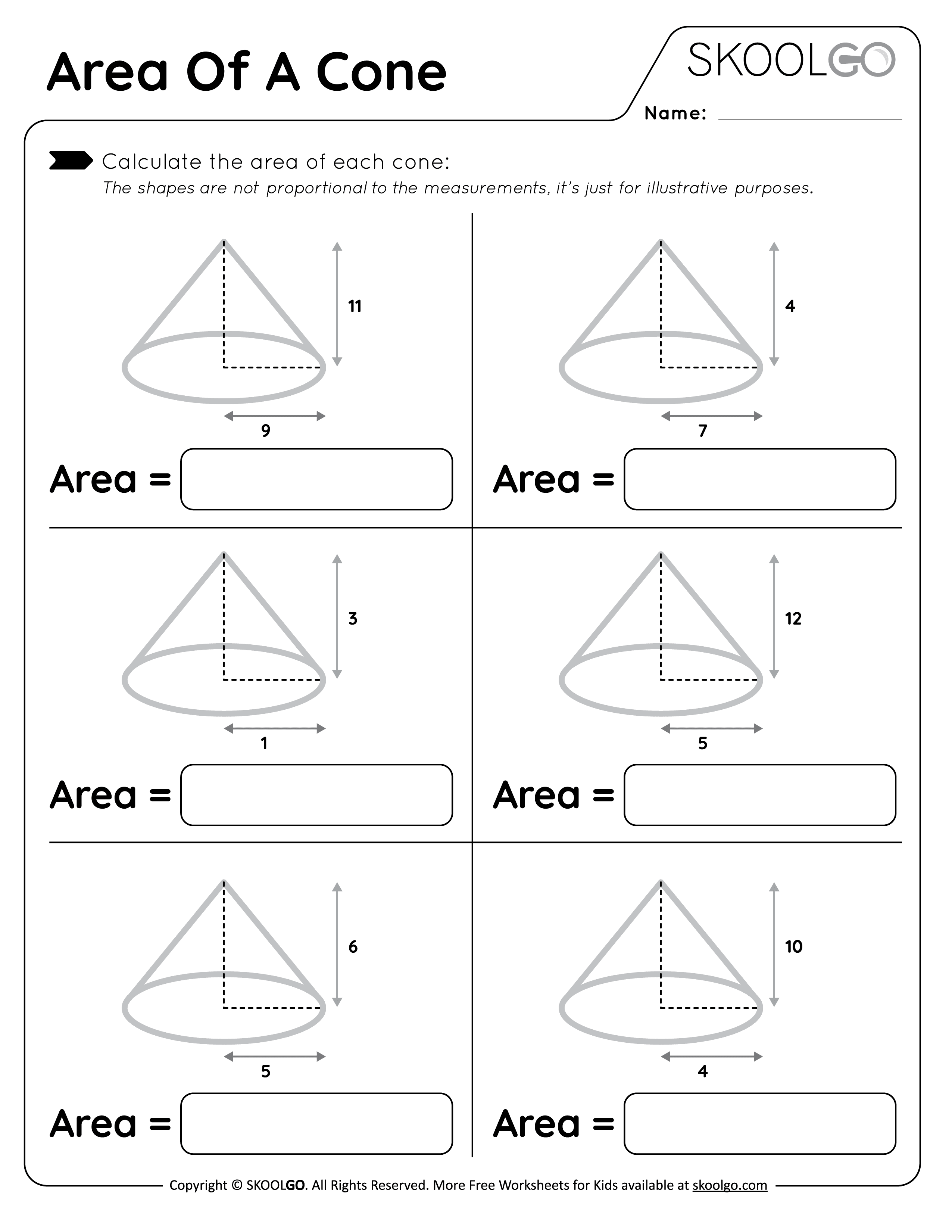
Attempt to solve these equations using the techniques described above, then verify your work using our free printable worksheet. Remember, practice is the key to mastery.
Common Pitfalls and How to Avoid Them

Here are some common mistakes students make when rearranging equations:
- Incorrect Sign: Not changing the sign when transposing terms across the equals sign.
- Overlooking Terms: Failing to account for all terms in the equation.
- Incorrect Roots: Misapplying the square root or other roots to both sides.
🔎 Note: Always double-check your work by substituting your solution back into the original equation to ensure both sides are equal.
Applications of Rearranging Equations

Rearranging equations isn’t just an academic exercise; it has practical applications in various fields:
- Physics: Solving for velocity, acceleration, or force in mechanics problems.
- Chemistry: Manipulating gas law equations to find unknown variables like temperature or pressure.
- Engineering: Structural and electrical engineering often require solving for variables in circuit analysis or beam equations.
Now, let's conclude our journey through the world of rearranging equations. We've covered basic and advanced techniques, identified common mistakes, and provided practice problems. Understanding how to manipulate algebraic expressions allows you to solve for unknowns with precision and finesse. Remember, every step you take in this learning process builds the foundation for tackling more complex mathematical challenges. With consistent practice, using tools like the printable worksheet offered here, you'll become proficient in this essential skill, paving the way for deeper insights in various scientific and mathematical domains.
Why is rearranging formulas important in science?

+
Rearranging formulas is crucial in science because it allows for the isolation of variables to understand and predict the relationships between different quantities, which is essential for experimentation and analysis.
Can you rearrange any equation?

+
Yes, most equations can be rearranged, but it’s important to ensure that the steps taken are valid mathematically. Some equations might become complex, requiring a solid understanding of mathematical operations.
What are some tips for beginners in rearranging equations?

+
Start with simple equations, understand each operation you perform, always balance the equation, and practice regularly. Visual aids like color coding operations can also be helpful.