Master Proportions in Triangles with This Worksheet

In the world of mathematics, especially geometry, understanding proportions and ratios within triangles is fundamental. Whether you're a student, a tutor, or simply someone with a love for math, this blog post will dive deep into the methods and techniques to master triangle proportions. By the end, you'll have a robust understanding of how to apply these principles effectively with the help of a targeted worksheet designed to reinforce your knowledge.
Understanding Triangles

Before we explore proportions, let’s establish a solid foundation by understanding what triangles are and their properties:
- Definition: A triangle is a polygon with three sides and three angles.
- Types of Triangles:
- Equilateral – All sides and angles are equal.
- Isosceles – Two sides and angles are equal.
- Scalene – No sides or angles are equal.
- Basic Angle Types:
- Acute – Less than 90°.
- Right – Exactly 90°.
- Obtuse – Greater than 90°.

Introducing Proportions

Proportions are mathematical statements that two ratios are equal. In the context of triangles:
- If a:b = c:d, then a x d = b x c.
- In triangles, proportions often relate to:
- Side lengths.
- Angles.
- Special lines like medians, altitudes, and angle bisectors.
Similarity of Triangles

Two triangles are similar if:
- All corresponding angles are equal.
- The ratios of corresponding sides are equal.
Worksheet: Triangles Proportions

Here’s a structured worksheet designed to help you master proportions in triangles:
| Problem Type | Example |
|---|---|
| Similar Triangle Identification | Given two triangles ABC and DEF, determine if they are similar. |
| Proportion in Side Lengths | If triangle XYZ has sides 4 cm, 6 cm, and 8 cm, find similar triangles with ratios 1:2 and 2:3. |
| Using Proportions to Find Heights | Calculate the height of a triangle if similar triangles have a height ratio of 3:5 and one triangle’s height is known. |
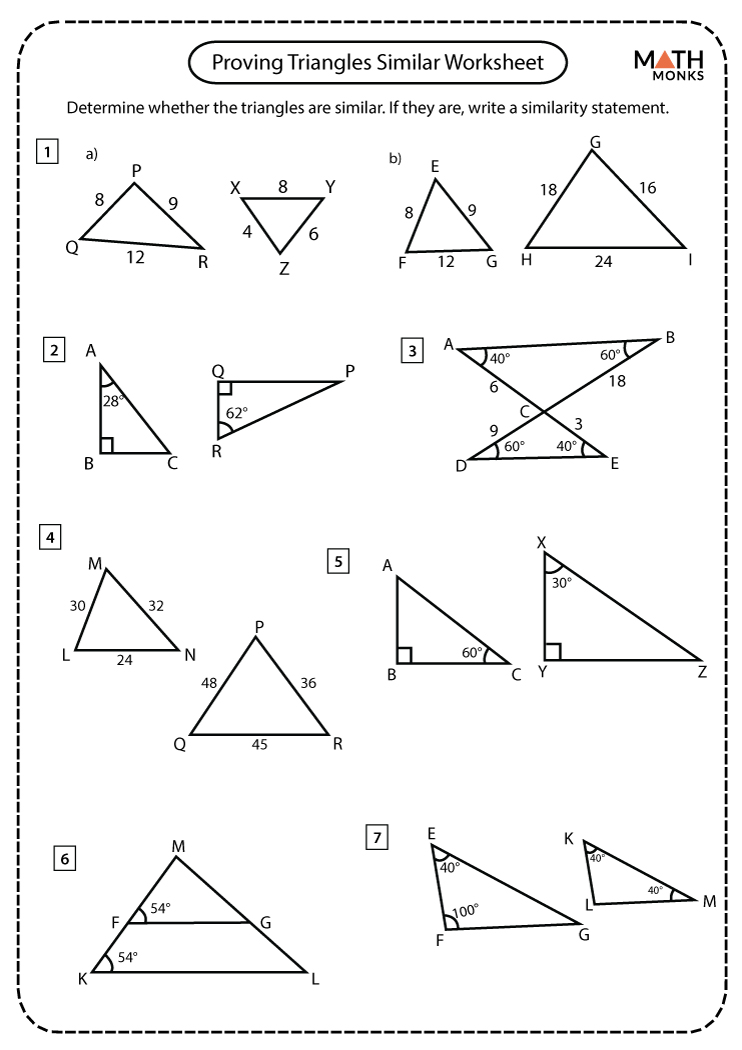
To further your practice:
- Exercise 1: Determine the side lengths of a triangle that is similar to one with sides of 3, 4, and 5 using a ratio of 2:1.
- Exercise 2: Use triangle proportions to solve real-world problems like the height of buildings or the size of shadows.
📝 Note: Practice regularly to ensure that these concepts become second nature. Using different ratios and proportions in varied problems can improve your flexibility in applying these principles.
Conclusion

Proportions within triangles are not only crucial for geometry but are also an essential part of problem-solving in physics, engineering, and art. With this worksheet, you now have a practical tool to delve into this intriguing aspect of mathematics. By exploring triangle types, understanding similarity, and actively practicing with proportions, you’ll soon find that solving these problems becomes intuitive. Remember, the key to mastering these principles is consistent practice and application in diverse contexts.
Why are proportions important in triangles?

+
Proportions are essential for identifying and working with similar triangles, which helps in solving for unknown values in geometry, engineering, and various applications in science and art.
Can I find proportions of triangles using only angles?

+
While angles are part of triangle similarity, you generally need at least one side length to set up a proportion to find the others. Proportions typically relate to side lengths or special line segments within triangles.
What’s the difference between similar and congruent triangles?

+
Similar triangles have the same shape but can differ in size (proportions are equal). Congruent triangles have both shape and size identical, meaning their corresponding sides and angles are equal in measure.