5 Essential Velocity and Acceleration Problems Solved

When diving into the world of physics, the concepts of velocity and acceleration are often among the first explored. These fundamentals help us understand motion in a quantifiable way, offering insight into how objects move through space and time. In this comprehensive guide, we will tackle five essential problems related to velocity and acceleration, providing detailed solutions that clarify these foundational principles.
Problem 1: Constant Acceleration in a Straight Line

Consider a car starting from rest, accelerating uniformly to reach a speed of 36 km/h in 10 seconds. Calculate:
- The acceleration
- The distance traveled during this acceleration
Solution:

Step 1: Convert km/h to m/s: 36 km/h = 36 / 3.6 = 10 m/s
Step 2: Calculate acceleration: Acceleration (a) = change in velocity (Δv) / time (Δt)
Here, Δv = 10 m/s (final velocity) - 0 (initial velocity) and Δt = 10s
Thus, a = (10 - 0) / 10 = 1 m/s2
Step 3: Distance traveled:
Using the equation: distance (s) = u * t + 0.5 * a * t2
where u (initial velocity) = 0 m/s, a (acceleration) = 1 m/s2, t (time) = 10s
s = 0 * 10 + 0.5 * 1 * 100 = 50 meters
🚀 Note: The importance of converting units for consistency in calculations cannot be overstated.
Problem 2: Deceleration in a Motion
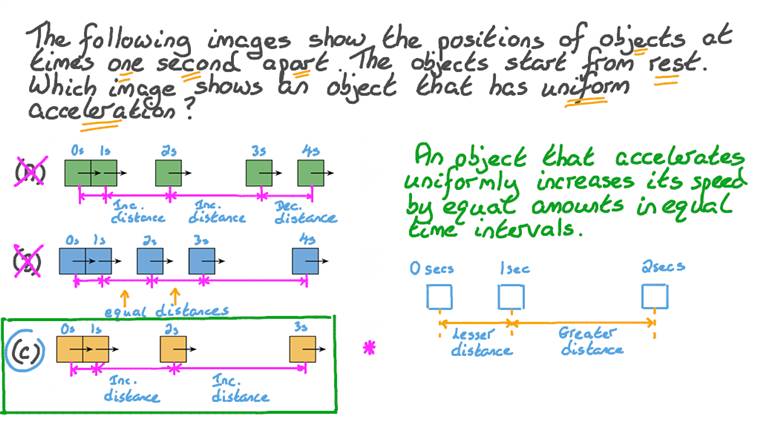
A ball is thrown vertically upward with an initial speed of 20 m/s. How long will it take for the ball to reach its highest point, and what is that maximum height?
Solution:

Step 1: Time to reach highest point: At the highest point, the final velocity (v) is 0. Using v = u + at:
0 = 20 - 9.81 * t (acceleration due to gravity is negative for upward motion)
t = 20 / 9.81 ≈ 2.04 s
Step 2: Maximum height: Using s = ut + 0.5 * a * t2
s = 20 * 2.04 + 0.5 * (-9.81) * (2.04)2 ≈ 20.4 - 10.2 ≈ 20.2 meters
Problem 3: Relative Velocity

An airplane is flying east at 120 m/s relative to the air, while the wind is blowing at 20 m/s from the west. What is the airplane’s speed relative to the ground?
Solution:

The wind velocity is subtracted from the airplane velocity because it’s against the airplane’s motion.
Speed relative to ground = 120 m/s - (-20 m/s) = 140 m/s east
🌬️ Note: Airspeed differs from ground speed due to wind's influence.
Problem 4: Circular Motion and Tangential Acceleration

A car travels around a circular track of radius 50 meters at a constant speed of 20 m/s. Calculate the tangential acceleration if the speed increases to 40 m/s over 20 seconds.
Solution:

The tangential acceleration (a) can be found using:
a = Δv / Δt
Δv = 40 m/s - 20 m/s = 20 m/s
Δt = 20 s
a = 20 / 20 = 1 m/s2
Problem 5: Position and Velocity with Constant Acceleration

A car travels from rest with an acceleration of 2 m/s2. What is its velocity and position after 5 seconds?
Solution:

Step 1: Velocity: Using v = u + at, where u = 0, a = 2 m/s2, and t = 5s
v = 0 + 2 * 5 = 10 m/s
Step 2: Position:
s = 0.5 * a * t2
s = 0.5 * 2 * 25 = 25 meters
Understanding these examples

The problems solved here have illustrated several key principles:
- The role of acceleration in changing velocity over time.
- The impact of wind or external forces on relative velocity.
- How position changes with time under constant acceleration.
- The transformation of speed into circular motion with tangential acceleration.
These principles are not just theoretical; they are essential for real-world applications, from engineering designs to understanding the natural movement of celestial bodies. They offer a deeper appreciation of how we interact with and navigate our physical environment. The solutions provided also highlight the importance of unit conversions and the correct application of physics formulas, ensuring that results are both accurate and meaningful.
What’s the difference between velocity and speed?

+
Speed is a scalar quantity that measures the rate of change of distance, whereas velocity is a vector that includes both speed and direction.
How does acceleration relate to velocity?

+
Acceleration is the rate of change of velocity over time. It tells us how quickly an object’s velocity changes, in either magnitude or direction.
Why does relative velocity matter?

+
Relative velocity matters because objects do not travel in isolation. The motion of one object relative to another can have significant implications, especially in scenarios like aviation or navigation at sea.
Can acceleration be negative?
+
Yes, acceleration can be negative, indicating deceleration or a decrease in speed, or it can imply acceleration in the opposite direction to the motion.