Proof Practice: Mastering Mathematical Proofs with Ease

Mathematics, often regarded as the language of the universe, communicates its truths through the rigorous and systematic approach of proof. For many students, the transition from problem-solving to constructing and understanding mathematical proofs can be both exhilarating and daunting. This post aims to guide you through proof practice, offering insights and strategies to master this critical skill in mathematics with ease.
The Importance of Proofs in Mathematics

Proofs serve as the foundation upon which mathematical theorems stand. They ensure that mathematical statements are not just assumptions but logical necessities:
- Establish Truth: A proof verifies a mathematical statement beyond doubt, providing a logical argument or sequence of statements showing it follows from accepted axioms and other proven results.
- Clarify Understanding: The process of proving or constructing proofs forces mathematicians to understand the underlying principles and connections within mathematics deeply.
- Facilitate Progress: New theorems are often built on previously established proofs, propelling mathematical research and development forward.
Common Types of Proofs

Understanding different proof techniques can significantly aid your proof practice:
- Direct Proof: A straightforward argument from the given hypothesis to the desired conclusion.
- Proof by Contrapositive: Proving the contrapositive of a statement, where if proving P \rightarrow Q is difficult, one might show \neg Q \rightarrow \neg P .
- Proof by Contradiction: Assuming the negation of the theorem leads to a contradiction, hence proving the theorem.
- Mathematical Induction: Used to prove a statement is true for all natural numbers through the base case and the induction step.
- Existence Proofs: Demonstrating that there exists at least one element satisfying a property.
Strategies for Effective Proof Practice

Practicing proofs is an art as much as it is a science. Here are some strategies to enhance your ability to craft proofs:
Understand the Problem

- Read Carefully: The theorem or proposition statement is key. Ensure you understand what needs to be proved.
- Break it Down: Identify the given information, the conclusion, and the logical steps you need to connect them.
- Write Down Your Initial Thoughts: Jot down any known theorems or properties that might help.
Use Visual Aids and Examples

- Visual representations can sometimes reveal patterns or provide insights into the logical structure of the proof.
- Work through specific examples to get a feel for how the proof should unfold.
Develop a Proof Strategy

Decide on a proof technique:
- Is a direct proof the best approach, or should you consider another method like proof by contradiction?
- Can you prove the theorem by constructing a mathematical model or a logical argument?
Write Clearly and Concisely

- Organize your proof as a narrative. Ensure each step logically follows the previous one.
- Use formal mathematical language and notation to avoid ambiguity.
- Review and revise your proof to ensure clarity and coherence.
Practice Regularly

Consistent practice is essential:
- Set aside time regularly to practice proofs from various areas of mathematics.
- Work on proofs that challenge you to think outside your comfort zone.
💡 Note: Learning how to read and critique other proofs can be as valuable as creating your own.
Overcoming Common Challenges in Proofs

Here are some common hurdles students face when engaging in proof practice:
Paralysis by Analysis
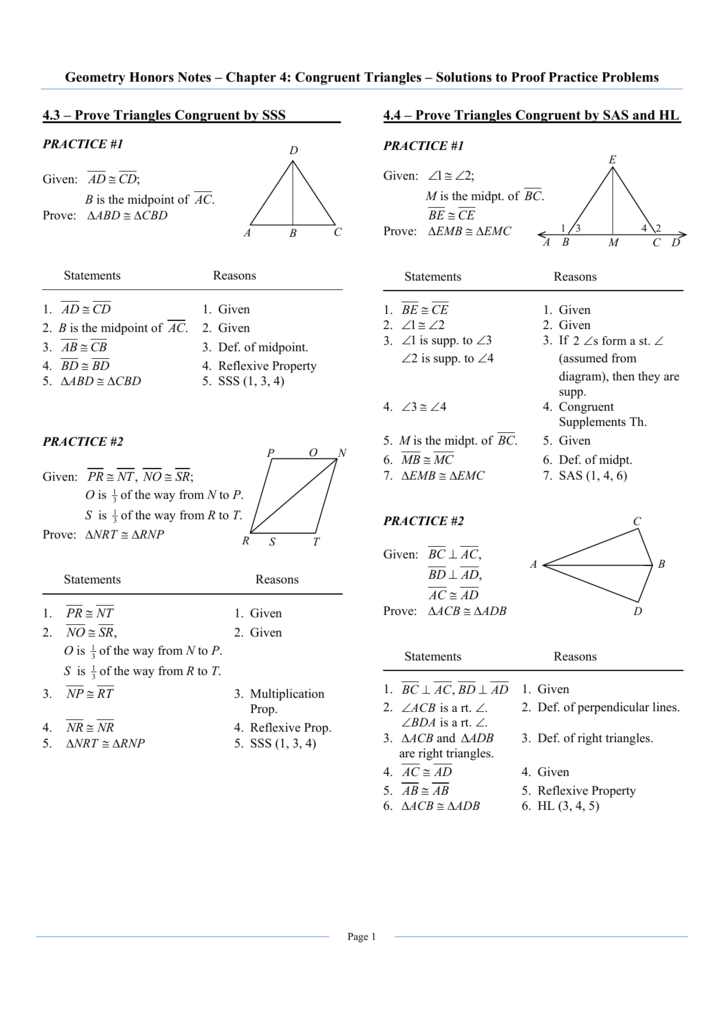
- Sometimes, the fear of making a mistake can prevent you from starting or continuing a proof.
- Approach proofs incrementally. Work through simpler problems to build confidence.
Over-Reliance on Specific Examples

- While examples are excellent for inspiration, they shouldn’t limit your proof to one method.
- Try to generalize from examples to formulate more abstract proofs.
Fear of Contradiction

- Proof by contradiction might seem counterintuitive, but practice showing that the assumption leads to an absurd result.
- Remember that contradiction is a powerful tool in logical reasoning.
Final Thoughts

Mastering mathematical proofs isn’t about memorizing sequences or formulas but rather about engaging deeply with mathematical thought. Each proof is a story of logic, connection, and discovery. By adopting the strategies above, engaging in regular proof practice, and embracing the challenges that come with it, you can not only enhance your mathematical understanding but also appreciate the beauty of mathematics as a rigorously crafted narrative. Remember, every proof, every theorem, and every mathematical insight you encounter adds to the rich tapestry of mathematical knowledge. Keep practicing, exploring, and learning, as the journey through proofs is a path to not just learning mathematics but truly understanding it.
What is the difference between a conjecture and a theorem?

+
A conjecture is a statement proposed to be true but has not yet been proven or disproven. A theorem, on the other hand, is a conjecture or statement that has been logically and rigorously proven to be true within a mathematical system.
How do I know which proof technique to use?

+
Choosing the right proof technique often comes with experience. However, consider the structure of the problem, the nature of the statement to prove, and what you already know to guide your decision. Direct proofs are often used when the relationship between premises and conclusion is clear. If the direct route is hard, consider contrapositive or contradiction proofs.
Can a proof be wrong?
+Yes, proofs can be wrong due to logical errors, incorrect premises, or flawed reasoning. Proofs are subject to peer review, and errors are often caught during this process. However, once a proof is widely accepted and stands up to scrutiny, it becomes part of the mathematical canon.
Is intuition important in proofs?
+Intuition can guide the proof-making process, especially in finding a direction or strategy. However, intuition alone isn’t enough; a proof requires rigorous logical argumentation. Intuition can be developed through experience, reading others’ proofs, and practicing constructing your own.
How do I improve my speed in writing proofs?
+Improving speed comes from practice, understanding the underlying mathematical concepts deeply, and being familiar with common proof structures. Additionally, developing a mental ‘toolkit’ of useful theorems, strategies, and techniques will allow you to construct proofs more efficiently.



