Point-Slope Form Worksheet: 5 Expert Answers

The world of algebra introduces students to various forms of linear equations, among which the Point-Slope Form holds significant importance due to its practical applications in representing lines. In this article, we delve deep into the Point-Slope Form, providing answers to common questions, offering practical examples, and enriching understanding through interactive resources.
What is Point-Slope Form?

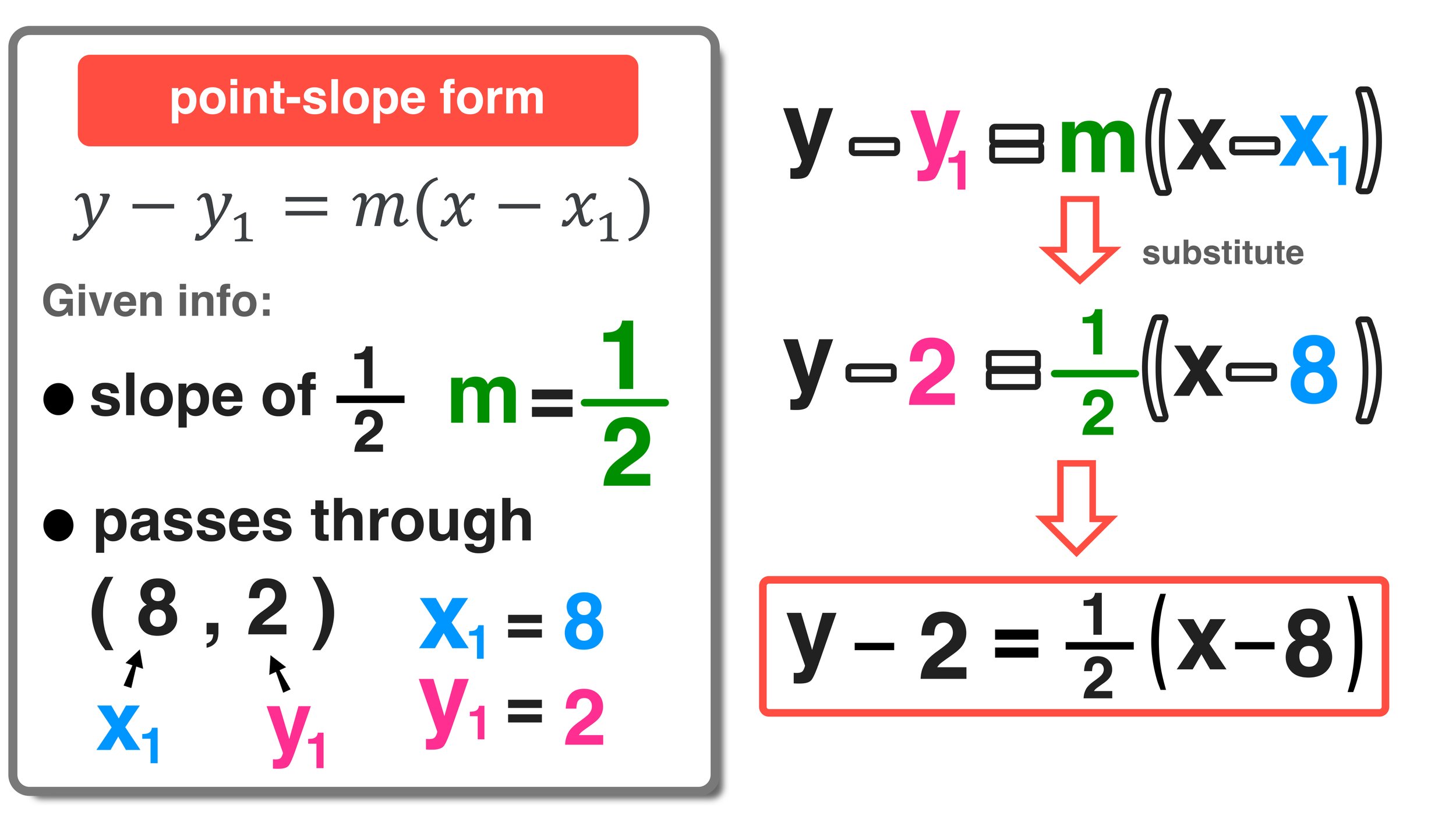
Point-Slope Form is a mathematical representation of the equation of a straight line, written as:
y - y1 = m(x - x1)
Where:
- m represents the slope of the line.
- (x1, y1) are the coordinates of any known point on the line.
Point-Slope Form is particularly useful when you know a point on the line and its slope, offering a straightforward path to the line's equation without first converting to another form.
Why is Point-Slope Form Useful?

- Simplicity: When you know the slope and a point, the equation can be derived almost instantly.
- Intuition: It directly connects the idea of slope with the change in y for a given change in x, making it intuitive for learners.
- Practical Applications: Useful in situations where you’re given real-world coordinates and slopes, like in navigation or engineering.
📌 Note: The Point-Slope Form is a stepping stone towards understanding more complex linear equations.
How to Use Point-Slope Form

Here’s a simple guide on how to use the Point-Slope Form:
- Identify the Slope (m): Either given or calculated from two points.
- Identify a Point (x1, y1): You can use any point on the line, but choosing a simpler one makes calculations easier.
- Plug into the Formula: Substitute m, x1, and y1 into the equation y - y1 = m(x - x1).
- Simplify: Optionally, simplify the equation to slope-intercept form (y = mx + b) if needed.
Real-World Applications
- Engineering: Designing slopes for roads, ramps, or pipelines.
- Economics: Analyzing the rate of change in profit, demand, or supply curves.
- Architecture: Calculating the incline of roof lines or the gradient of staircases.
- Physics: Studying the motion of objects at an incline, like a projectile’s path.
Common Mistakes in Using Point-Slope Form
Here are a few typical errors students encounter:
- Misplacing the signs: The equation should be y - y1 = m(x - x1), not the other way around.
- Substituting incorrect values for x1 and y1.
- Forgetting to simplify the equation if necessary.
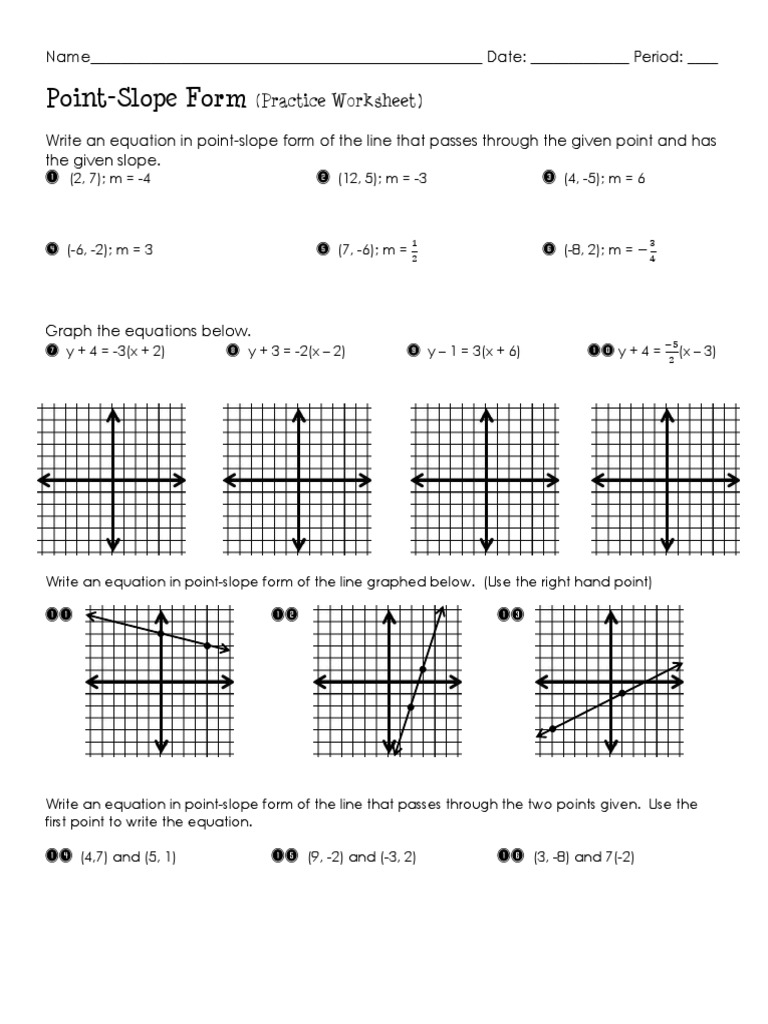
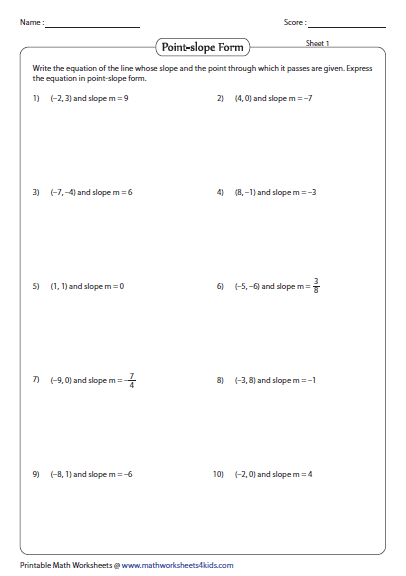
Exercise: Point-Slope Form Worksheet

Let’s practice with some exercises to solidify our understanding:
| Question | Solution |
|---|---|
| Find the equation of the line with slope m=3 passing through (2,5). | Using the formula y - 5 = 3(x - 2), we get y = 3x - 1 |
| A line passes through (-3, 4) and has a slope of -2. What is its equation? | With the formula y - 4 = -2(x + 3), we simplify to get y = -2x - 2 |

These exercises show how to apply the formula in practical scenarios.
📝 Note: Always check your work by substituting the point back into your final equation to ensure it satisfies the original point-slope information.
In wrapping up, understanding the Point-Slope Form is essential for mastering linear equations in algebra. It provides a straightforward method to find the equation of a line when given a point and a slope, making it invaluable in both theoretical and applied mathematics. By keeping the principles in mind and practicing regularly, you'll find this form to be an indispensable tool in your mathematical toolkit.
What if I only know two points on a line, no slope?

+
Calculate the slope first using the two points, then apply the Point-Slope Form.
Why do we not use a subtraction sign for x1?
+
The subtraction sign is implied in the formula y - y1 = m(x - x1); it’s just simplified to make it easier to use.
Can Point-Slope Form handle vertical lines?

+
Vertical lines have an undefined slope, so Point-Slope Form does not apply; use the equation x = constant.
Is there a way to use Point-Slope Form for horizontal lines?

+
The slope for a horizontal line is zero, so the equation simplifies to y = y1, which is a constant.
How can I transition from Point-Slope Form to Slope-Intercept Form?
+
Distribute the slope, move any constant term to the other side to isolate y, and simplify to get the equation in slope-intercept form (y = mx + b).