5 Essential Answers for Points, Lines, Planes Worksheet
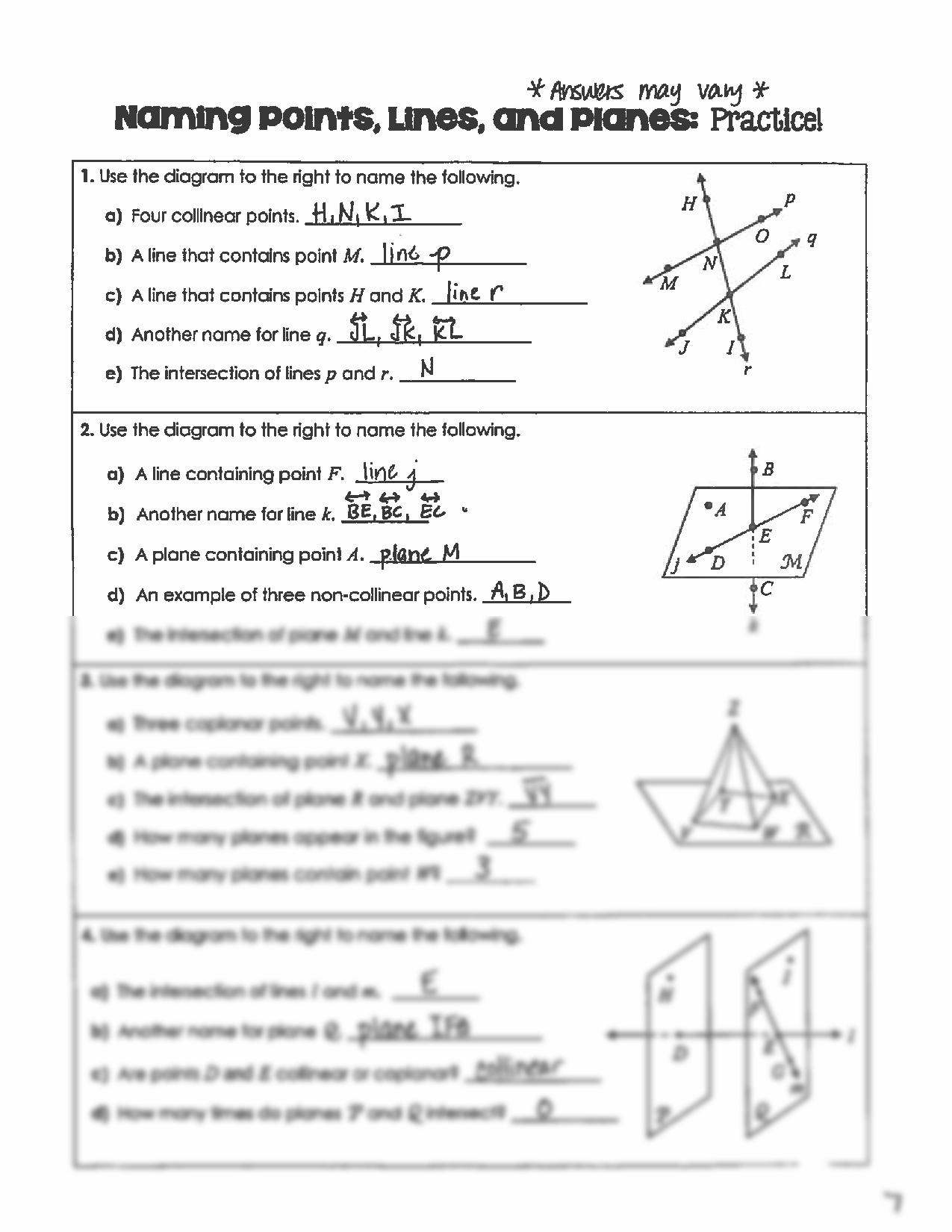
Geometry, with its rich history and practical applications, is often introduced in school with fundamental concepts like points, lines, and planes. These are not merely abstract ideas but foundational elements that form the basis of spatial understanding and more complex geometric theories. Today, we delve into five essential questions often found in Points, Lines, Planes Worksheets that can enhance your understanding of geometry:
1. What Defines a Point, Line, and Plane?

Geometry starts with the very basics:
- Point: A point in geometry has no dimension; it is simply a location in space represented by a dot (.).
- Line: A line is a one-dimensional figure with infinite length and no width. It's described by extending two points endlessly in both directions.
- Plane: A plane extends indefinitely in two dimensions. Imagine a flat surface, like a sheet of paper, but one that never ends.

📝 Note: These definitions might seem abstract, but they're the bedrock on which all geometric figures are built.
2. How Do Points, Lines, and Planes Relate to Each Other?

Understanding how these geometric elements interact is crucial:
- Two points define a line.
- Once you have two points, you can draw a line through them, extending infinitely in both directions.
- Three points not on the same line define a plane.
- If these points are not collinear, they determine a unique plane.
- Multiple lines can intersect at a single point or never meet, depending on their slopes or if they are parallel.
- A line can lie within a plane, in which case it divides the plane into two regions, or it can intersect the plane at a single point if they're not parallel.

3. What are Coplanar Points and Lines?

The concept of coplanarity is significant in geometry:
- Coplanar Points are points that lie in the same plane. If you can find three non-collinear points, they will define a plane in which all other points are coplanar.
- Coplanar Lines are lines that lie in the same plane. These lines might intersect, be parallel, or skew (if not parallel and not intersecting).
| Type of Points or Lines | Description |
|---|---|
| Coplanar Points | Points lying on the same plane. |
| Coplanar Lines | Lines that reside in the same plane. |

4. How to Identify and Classify Lines?

Lines have several classifications:
- Parallel Lines never meet and are always the same distance apart.
- Intersecting Lines cross at exactly one point.
- Perpendicular Lines intersect at a right angle (90 degrees).
- Skew Lines are lines that do not lie in the same plane and do not intersect.

5. What Role Do Axes and Coordinates Play with Points, Lines, and Planes?

The coordinate plane brings these concepts into a practical realm:
- Coordinate System helps to locate points with ordered pairs or triples (x, y), (x, y, z).
- Lines in a coordinate plane can be described by their slope and y-intercept (y = mx + b).
- Planes are often represented by equations with three variables (ax + by + cz = d), where a, b, and c are not all zero.
📝 Note: In a 3D coordinate system, planes divide space into regions, much like a line divides a 2D plane into two regions.
To understand these relationships, imagine:
- A single point, like (2, 3), can be plotted on a coordinate plane.
- A line connecting two points, say (1, 1) and (3, 3), has a slope of 1.
- A plane, such as 3x + 2y + z = 6, intersects the x-axis at (2, 0, 0), the y-axis at (0, 3, 0), and the z-axis at (0, 0, 6).
In wrapping up our exploration of points, lines, and planes, we’ve journeyed through the essential building blocks of geometry. From understanding how these elements relate to one another to seeing their practical applications through coordinates, we’ve covered a lot of ground. Recognizing the spatial relationships and classifications between these geometric constructs allows us not only to solve problems in mathematics but also to comprehend and describe the world around us. Whether you’re navigating through an actual physical space or working with abstract mathematical problems, these fundamentals provide the tools to dissect and analyze complex systems with clarity and precision.
Can two lines intersect at more than one point?

+
No, two lines in Euclidean geometry can intersect at only one point. If they intersect at multiple points, they would be considered the same line.
How can you tell if two planes are parallel?

+
Two planes are parallel if their normal vectors are parallel, or if they have the same directional coefficients in their plane equations.
What is the difference between a line and a ray?
+
A line extends infinitely in both directions, while a ray has one endpoint and extends infinitely in one direction from that point.
Are all lines in 3D space skew?

+
No, lines in 3D space can be parallel, intersecting, or skew. Skew lines do not lie in the same plane and do not intersect.



