5 Key Answers to Energy Skate Park Simulation Worksheet

Understanding the principles of physics through simulation can provide an engaging learning experience. The Energy Skate Park simulation, widely used in educational settings, allows students to explore concepts such as potential energy, kinetic energy, and conservation of energy through an interactive digital park. This post delves into five key answers related to the Energy Skate Park simulation worksheet, helping you understand the underlying physics and get the most out of your learning experience.
Understanding Energy Transfers
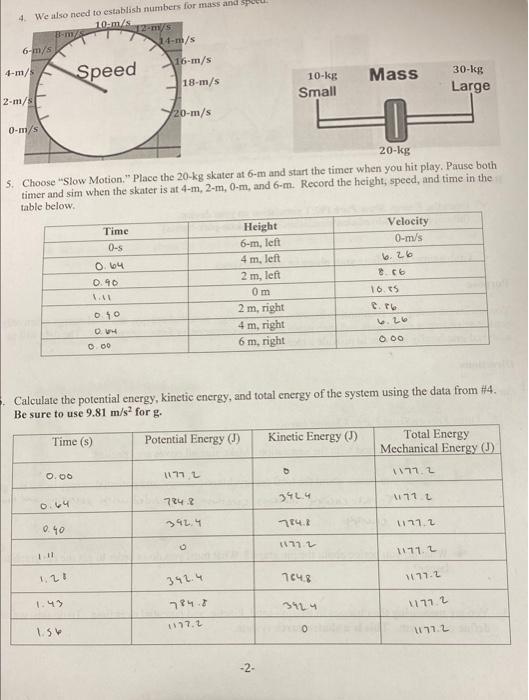
At the heart of the Energy Skate Park simulation is the principle of energy conservation. Here are the key points to understand:
- Potential Energy: The energy a skater possesses due to his or her position in the park. When the skater is at the top of a ramp, they have the most potential energy.
- Kinetic Energy: The energy of motion. When the skater descends, potential energy is converted into kinetic energy.
- Conservation of Energy: The total mechanical energy (sum of kinetic and potential energy) remains constant in the absence of friction or air resistance.

How to Measure and Analyze Skateboard Path

The simulation allows you to track the path of the skateboard:
- Use the Energy Bar Graph: This visual tool displays the distribution of energy at any given moment during the skate.
- Pie Charts: Helps to see the relative amount of potential and kinetic energy instantly.
- Track: Modify the track and observe how the energy changes affect the skateboard’s path.
Energy Loss and Real-World Scenarios

While the simulation usually assumes an ideal scenario:
- In real life, energy losses due to friction and air resistance reduce the total mechanical energy.
- Friction: Can be added in advanced settings to simulate a more realistic scenario, showing how energy is converted into heat or sound.
🔍 Note: Real-world skate parks would also experience energy loss, making perfect energy conservation impossible.
Simulating Different Skateboarders
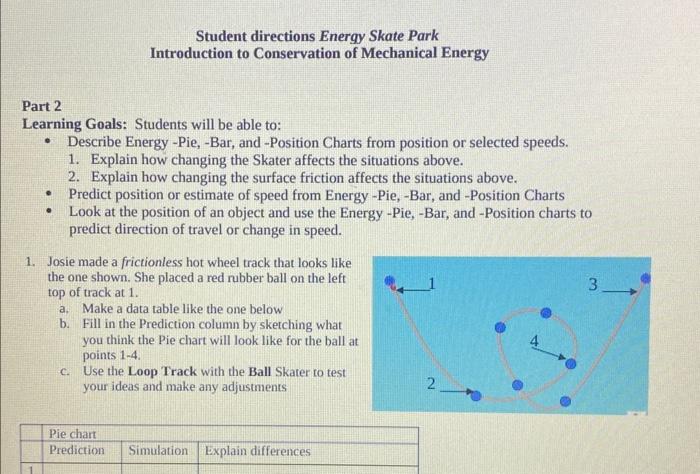
The simulation allows you to change the skateboarder’s mass:
- Heavier skateboarders have more potential energy when raised to the same height due to the equation PE = mgh.
- The skatepark’s layout, including the heights and lengths of tracks, will affect the energy profile of different mass skaters differently.
Advanced Features for Enhanced Learning

The simulation includes features to deepen understanding:
- Friction Slider: Allows you to introduce friction, which is a more realistic condition.
- Slow Motion: To observe energy transitions more clearly.
- Custom Skatepark Builder: Create various setups to explore different energy scenarios.
In this blog post, we’ve explored various aspects of the Energy Skate Park simulation worksheet. From understanding energy transfers, measuring and analyzing skateboard paths, simulating energy loss in real-world scenarios, to simulating different skateboarders, we’ve covered critical concepts that not only aid in learning physics but also make it interactive and engaging.
Remember, the beauty of physics simulations lies in their ability to visualize complex concepts, making them accessible and enjoyable. Whether you’re a student, a teacher, or just someone interested in physics, tools like the Energy Skate Park simulation provide an invaluable platform for learning and experimentation.
What does potential energy mean in the Energy Skate Park?

+
Potential energy in the skate park refers to the energy stored by the skateboarder due to their position or height. It’s the energy that will be converted into kinetic energy as the skater moves down the ramp.
Why does the skateboard slow down even when friction is set to zero?
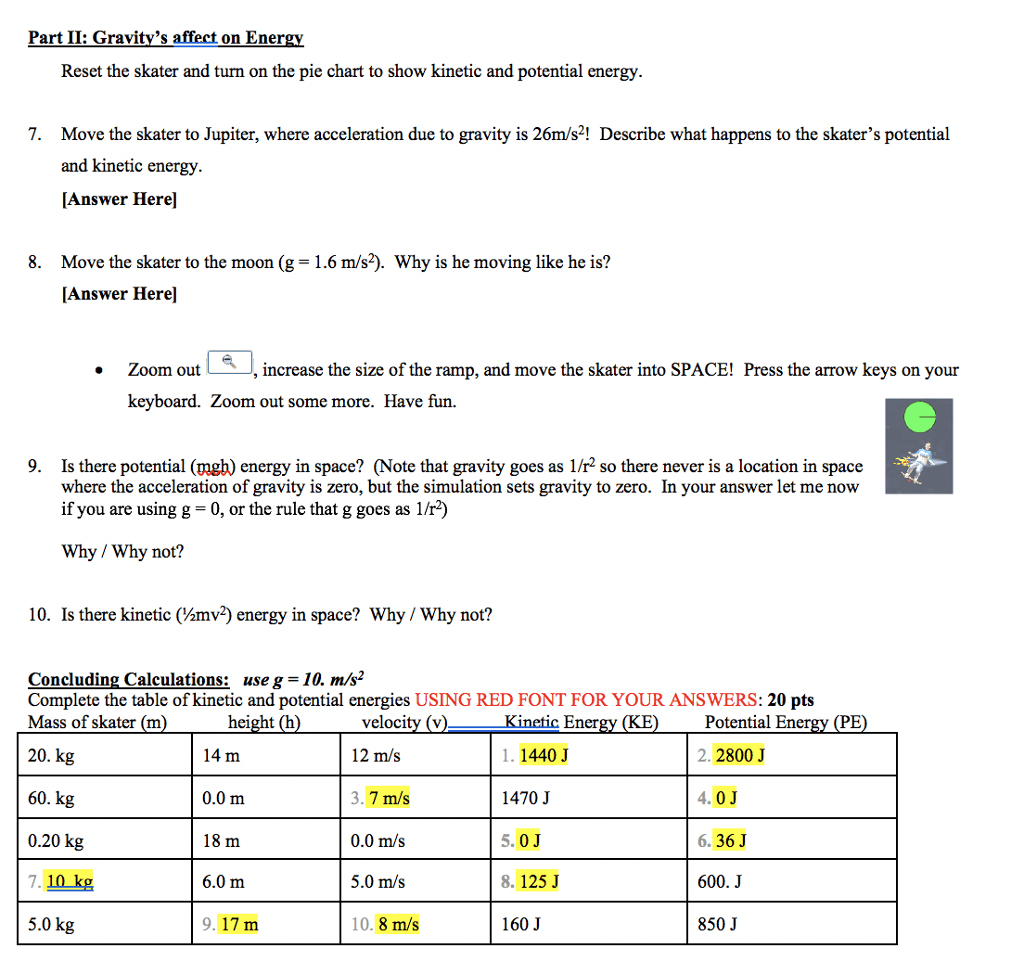
+
Setting friction to zero in the simulation assumes an ideal scenario. In reality, there would still be air resistance and other minor energy losses, but in the simulation, you can observe pure energy conservation.
How does changing the mass of the skateboarder affect the simulation?

+
Changing the mass alters the skateboarder’s potential energy since potential energy is directly proportional to mass. Heavier skateboarders will have more potential energy at a given height, affecting how they move through the skatepark.



