5 Operations With Polynomials Worksheet Tips

Polynomials are fascinating components of algebra, providing a foundational understanding necessary for higher mathematics, engineering, and many other fields. Whether you're a student preparing for an algebra exam or a math enthusiast, enhancing your skills in operations with polynomials can significantly improve your problem-solving abilities. Here, we'll dive into some useful tips and strategies to effectively tackle polynomials in worksheets, ensuring you're equipped with the knowledge to excel in your studies or everyday math challenges.
Understanding Polynomial Basics


Before we delve into the operations, let’s quickly recap what polynomials are. A polynomial is an expression consisting of:
- Variables (like x, y, z)
- Constants (numbers like 3, -5, 2.5)
- Non-negative integer exponents (no negative or fractional exponents for traditional polynomials)
📘 Note: Polynomials can have an infinite number of terms, but we commonly work with polynomials of a few degrees for simplicity.
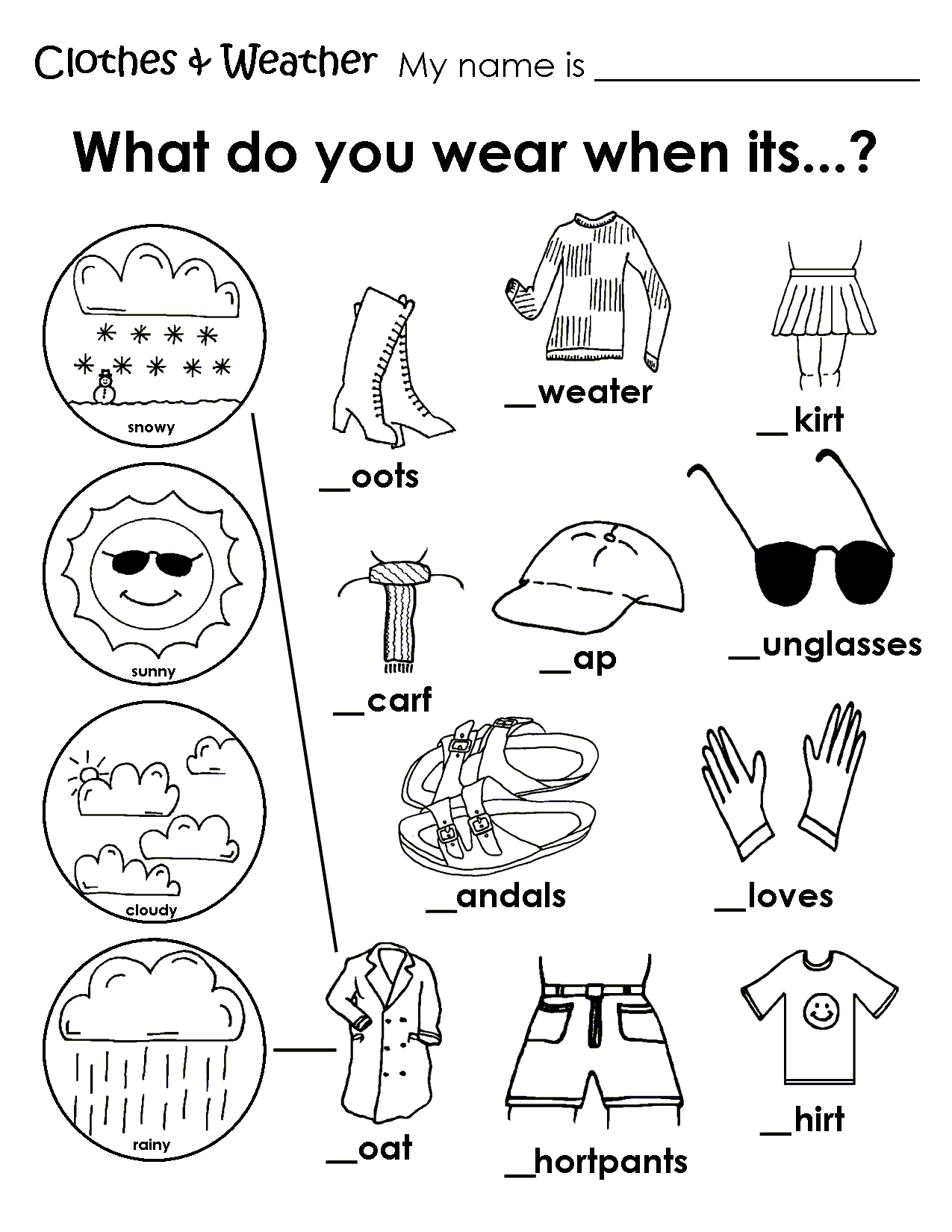
Tip 1: Simplify Before Operations

Before performing operations on polynomials, simplify them as much as possible:
- Combine like terms
- Use the distributive property to expand expressions
- Arrange terms in standard form (leading term to the smallest degree term)
| Original Polynomial | Simplified Polynomial |
|---|---|
| 3x3 + 4x - 3x + 2 + x3 | 4x3 + x + 2 |

Tip 2: Use Polynomial Identities for Faster Calculations

Leveraging polynomial identities can streamline your calculations:
- Difference of Squares: (a^2 - b^2 = (a + b)(a - b))
- Sum and Difference of Cubes: (a^3 + b^3 = (a + b)(a^2 - ab + b^2)), (a^3 - b^3 = (a - b)(a^2 + ab + b^2))
- Binomial Expansion: Use Pascal’s Triangle or the Binomial Theorem for ((a + b)^n)
📐 Note: Knowing these identities saves time and reduces the complexity of polynomial problems.
Tip 3: Practice Factoring Techniques

Factoring polynomials is an essential skill:
- Check for the greatest common factor (GCF)
- Use techniques like grouping, the difference of squares, sum or difference of cubes, and quadratic factoring
- Remember to factor completely
⚙️ Note: Factoring is not just about division; it’s about recognizing patterns and structures within polynomials.
Tip 4: Apply the Remainder and Factor Theorems

The Remainder Theorem states that for any polynomial (P(x)) and a number (c), the value of (P©) is the remainder when (P(x)) is divided by (x - c). The Factor Theorem builds on this, stating that if (P© = 0), then (x - c) is a factor of (P(x)). These theorems can:
- Help you evaluate polynomials at specific points
- Provide insight into finding factors without the need for long division
Tip 5: Explore Polynomial Graphs
Understanding how polynomials graph can:
- Show the behavior of polynomials as x approaches infinity or negative infinity
- Help visualize real roots (x-intercepts) and the number of turning points
- Provide context for sign changes and relative maxima/minima
These tips are not exhaustive but are vital starting points for anyone working with polynomials. Enhancing your proficiency in operations with polynomials will not only aid in algebraic problem-solving but also in tackling calculus, physics, and many scientific fields where polynomials are ubiquitous. Remember, like any skill, practice is key. Engage with varied types of problems, work through polynomial sheets systematically, and apply these techniques consistently to master polynomial algebra. By doing so, you'll find polynomials become less of a challenge and more of an opportunity to showcase your mathematical acumen.
How can I quickly identify the degree of a polynomial?

+
The degree of a polynomial is the highest power of the variable within it. For example, in (3x^4 + 2x^2 - 5), the degree is 4 because the term (3x^4) has the highest exponent.
Can polynomials have negative or fractional exponents?
+
In classical algebra, polynomials are defined with non-negative integer exponents. However, in advanced mathematics, you might encounter rational functions with negative or fractional exponents, which are not considered polynomials.
What’s the most effective way to practice polynomial operations?

+
Consistent practice with a variety of problems is key. Use polynomial worksheets, online resources, and work on different operations like addition, subtraction, multiplication, division, and factoring to build a comprehensive understanding.