5 Easy Steps to Find the Slope: Worksheet Guide

Introduction to Slope

Understanding slope is crucial when learning about lines and their equations in mathematics. Slope refers to how steep a line is, or more technically, the ratio of the vertical change (the rise) to the horizontal change (the run) between any two points on a line.

How to Find the Slope

Finding the slope of a line involves following these five easy steps:
1. Identify Two Points on the Line
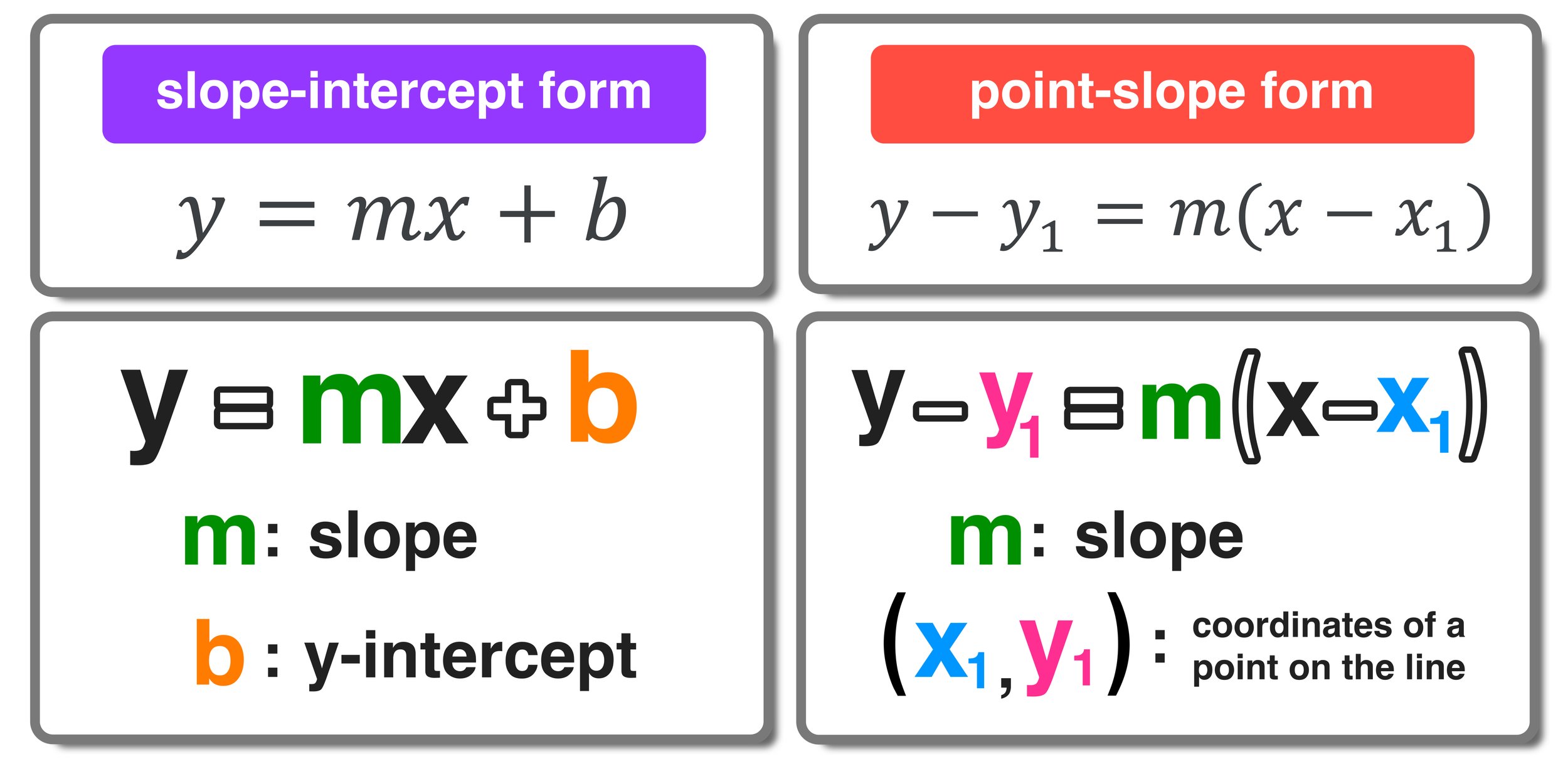
Begin by selecting any two distinct points on the line. These points are generally denoted as (x1, y1) and (x2, y2).
- Point 1: (x1, y1)
- Point 2: (x2, y2)
🔍 Note: If the line is given in a different form, like in standard form or slope-intercept form, you'll need to convert it to the point-slope form to use this method.
2. Calculate the Change in Y

The next step involves finding the vertical change between the two points. This is simply the difference in the y-coordinates of the two points:
[ \Delta y = y_2 - y_1 ]
3. Calculate the Change in X

Similarly, calculate the horizontal change by finding the difference in the x-coordinates:
[ \Delta x = x_2 - x_1 ]
4. Find the Slope

Now, use the formula for the slope:
[ \text{slope} = \frac{\Delta y}{\Delta x} ]
Here, you divide the change in y by the change in x.
5. Interpret the Slope

After calculating the slope:
- If the slope is positive, the line ascends from left to right.
- If the slope is negative, the line descends from left to right.
- A slope of zero means the line is horizontal.
- An undefined slope indicates a vertical line.
Using a Worksheet for Practice
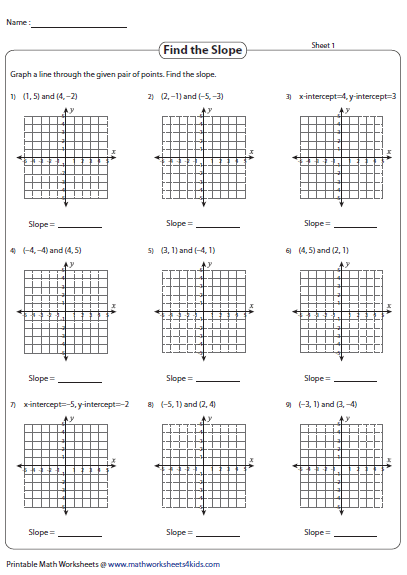
Worksheets are a great way to reinforce these steps. Here’s how you can approach them:
| Step | Action |
|---|---|
| 1 | Identify two points from the graph or equation provided in the worksheet. |
| 2 | Use the given points to calculate the change in y. |
| 3 | Calculate the change in x. |
| 4 | Find the slope by dividing Δy by Δx. |
| 5 | Interpret the result and check if it aligns with the line's visual slope. |

🧑🏫 Note: Worksheets often have lines with different slopes, so practicing with various examples will enhance your understanding.
Challenges You Might Encounter

Here are some common challenges and how to address them:
- Vertical and Horizontal Lines: You need to understand that a vertical line has an undefined slope (as Δx = 0), and a horizontal line has a slope of zero (as Δy = 0).
- Graph Interpretation: Misreading the scale on the graph or misidentifying points can lead to incorrect slope calculations. Double-check your points.
- Formulas and Miscalculation: Misapplication of the slope formula can occur; ensure you're using the correct points and performing the arithmetic correctly.
💡 Note: Practicing with real graphs or plotting lines on grid paper can help with these issues.
Final Thoughts

Finding the slope of a line involves a straightforward process once you’re familiar with the steps. It’s about understanding how to interpret points, calculate changes, and apply the slope formula. Remember, the key to mastering this concept lies in:
- Identifying points on a line accurately.
- Performing the necessary calculations with attention to detail.
- Interpreting the slope to understand the line's behavior.
By engaging with worksheets and applying these steps, you’ll gain confidence in solving slope-related problems, paving the way for more advanced topics in geometry and algebra.
What is the difference between positive and negative slope?

+
A positive slope means the line rises from left to right, indicating an increase in y as x increases. A negative slope means the line falls from left to right, showing a decrease in y as x increases.
Can a line have a slope of zero?

+
Yes, a line with a slope of zero is horizontal, meaning there is no vertical change between any two points on the line.
What does it mean if a line has an undefined slope?
+
An undefined slope indicates a vertical line where the x-coordinate remains the same, hence the change in x (Δx) is zero, making the slope calculation mathematically undefined.
How does the slope formula work if the line is not straight?

+
The slope formula works only for straight lines. For non-straight lines (curves), you would need to use calculus to find the slope (derivative) at a specific point.


