One Step Equation Worksheet: Master Math with Ease
In the realm of mathematics, one of the most foundational and practical skills to master is solving one-step equations. Whether you're a student brushing up for exams or an adult getting back into math, understanding this concept can pave the way for tackling more complex equations and mathematical problems. In this extensive guide, we'll explore why one-step equations are crucial, how they are constructed, and provide you with a detailed worksheet for practice, along with strategies to solve them effortlessly.
Why One-Step Equations Matter

At the core, one-step equations introduce you to the basic principles of algebra. Here’s why mastering them is important:
- Building Block of Algebra: They are the simplest form of algebraic equations, teaching the fundamental concept of balancing both sides of an equation.
- Mathematical Thinking: Solving these equations encourages logical thinking, which is beneficial across various fields and daily life scenarios.
- Preparation for Higher Math: Understanding one-step equations is vital for learning multi-step equations, systems of equations, and beyond.
Constructing One-Step Equations

One-step equations take the form of (x + a = b), (x - a = b), (ax = b), or (\frac{x}{a} = b). Here, (x) is the variable you are solving for, and (a) and (b) are constants or known values.
- Addition/Subtraction Equations: To solve (x + a = b), subtract (a) from both sides. Similarly, for (x - a = b), add (a).
- Multiplication/Division Equations: For (ax = b), divide by (a) on both sides. To solve (\frac{x}{a} = b), multiply both sides by (a).
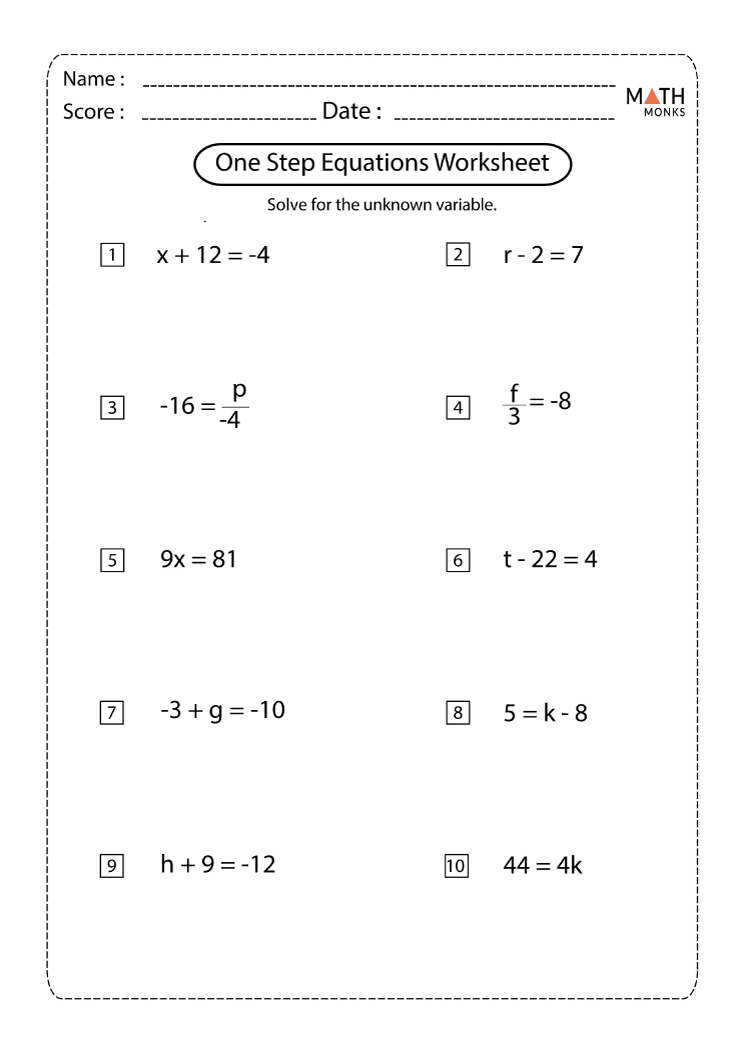
One-Step Equation Worksheet

Here’s a worksheet to practice one-step equations. Try to solve each problem:
| Equation | Solution |
|---|---|
| (x + 7 = 12) | |
| (x - 3 = 11) | |
| (4x = 24) | |
| (\frac{x}{5} = 2) | |
| (x + 0.5 = 2) |

⚠️ Note: While solving these equations, remember to keep your calculations neat and double-check your answers for accuracy.
Strategies for Solving One-Step Equations

Here are some strategies to solve one-step equations with ease:
- Keep it Balanced: Whatever you do to one side of the equation, do it to the other to maintain equality.
- Isolate the Variable: Your goal is to get the variable by itself on one side of the equation. This usually involves adding, subtracting, multiplying, or dividing both sides by the same number.
- Perform Inverse Operations: Use the inverse operation of what’s being done to the variable to cancel it out. For example, if (x) is multiplied by a number, divide by that number to isolate (x).
- Check Your Work: After solving, substitute your answer back into the original equation to ensure it works.
In mastering one-step equations, the journey is about developing a sense of understanding mathematical relationships. As you practice, you'll find that your approach to problem-solving becomes more instinctive, paving the way for more complex algebraic challenges. Keep refining your skills with constant practice, and don't shy away from seeking clarification when something seems unclear.
What’s the difference between addition and subtraction equations?

+
While they seem like two sides of the same coin, addition equations involve adding a constant to the variable, and the inverse operation to solve is subtraction. Conversely, subtraction equations have a constant subtracted from the variable, and the inverse operation to solve is addition.
How can I check my solutions?
+
To check your solution, substitute the value you found for (x) back into the original equation. If the equation holds true, your solution is correct.
Are one-step equations always linear?
+
Yes, one-step equations are linear, as they involve only one operation to solve for the variable, maintaining a linear relationship between variables and constants.