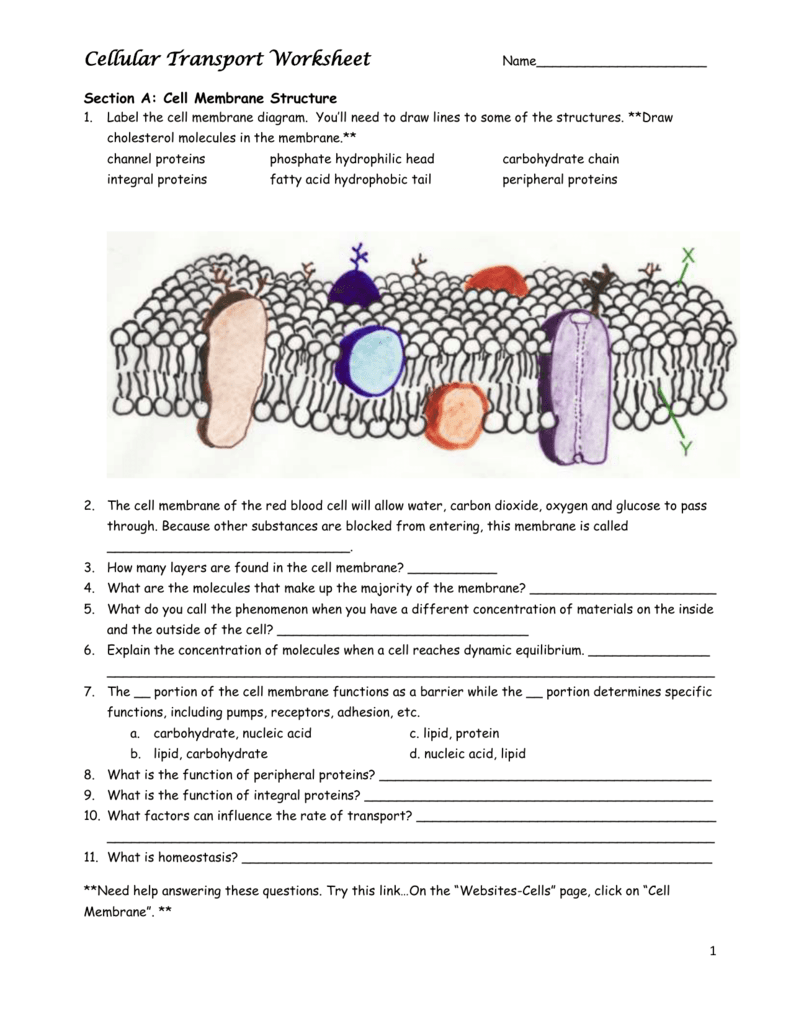
5 Essential Answers to Ohm's Law Problems

In the fascinating world of electrical engineering, physics students, and enthusiasts alike often encounter a key principle that guides the understanding of electrical circuits - Ohm's Law. This fundamental law forms the bedrock of electronics, allowing us to predict how voltage, current, and resistance interact within a circuit. This post will delve into five essential problems that illustrate the practical application of Ohm's Law, providing clarity and insight into this vital scientific principle.
Problem 1: Calculating Voltage

When you’re given the resistance (R) in ohms (Ω) and the current (I) in amperes (A), calculating the voltage (V) across a component is straightforward. Let’s look at how to do it:
- Given: R = 5 Ω, I = 0.3 A
- Equation: V = I × R
- Solution: V = 0.3 A × 5 Ω = 1.5 V
With just these two pieces of information, we can solve for the voltage, making it possible to design circuits that operate at the desired voltage level.
Problem 2: Determining Current

Sometimes, the voltage and resistance are known, and the challenge is to find the current flowing through a circuit. Here’s how it’s done:
- Given: V = 12 V, R = 30 Ω
- Equation: I = V / R
- Solution: I = 12 V / 30 Ω = 0.4 A
This calculation is essential for ensuring components are not overburdened with excessive current, which could lead to overheating or circuit failure.
Problem 3: Finding Resistance

Understanding how to calculate the resistance of a component when you have the voltage and current is another key aspect of working with electrical circuits. Here is an example:
- Given: V = 9 V, I = 0.5 A
- Equation: R = V / I
- Solution: R = 9 V / 0.5 A = 18 Ω
Knowing the resistance allows you to select or design components that will give you the desired current for a given voltage.
Problem 4: Series Circuit Analysis

In series circuits, components are connected in a single loop. Here, we’ll analyze how Ohm’s Law applies to such a setup:
| Component | Resistance (Ω) | Voltage (V) |
|---|---|---|
| Resistor 1 | 10 Ω | V1 |
| Resistor 2 | 15 Ω | V2 |
| Total | 25 Ω | 15 V |

- Given: R1 = 10 Ω, R2 = 15 Ω, Vtotal = 15 V
- Total Resistance: Rtotal = R1 + R2 = 25 Ω
- Current in the circuit: I = Vtotal / Rtotal = 15 V / 25 Ω = 0.6 A
- Voltages across each resistor:
- V1 = I × R1 = 0.6 A × 10 Ω = 6 V
- V2 = I × R2 = 0.6 A × 15 Ω = 9 V
This problem demonstrates how the total voltage in a series circuit is divided according to the resistance of each component.
Problem 5: Parallel Circuit Analysis

In parallel circuits, components are connected across the same voltage source. Here’s how to analyze such a circuit using Ohm’s Law:
| Component | Resistance (Ω) |
|---|---|
| Resistor 1 | 20 Ω |
| Resistor 2 | 30 Ω |
| Total | Req |
- Given: R1 = 20 Ω, R2 = 30 Ω, Vsource = 9 V
- Equivalent Resistance: Req = (R1 × R2) / (R1 + R2) = (20 × 30) / (20 + 30) = 12 Ω
- Total Current: I = Vsource / Req = 9 V / 12 Ω = 0.75 A
- Current through each resistor:
- I1 = Vsource / R1 = 9 V / 20 Ω = 0.45 A
- I2 = Vsource / R2 = 9 V / 30 Ω = 0.30 A
This setup showcases how the current divides in a parallel circuit, where the voltage remains constant across each component.
💡 Note: In complex circuits, both series and parallel elements may coexist, requiring a combination of these principles to analyze the circuit fully.
Throughout this journey, we've seen how Ohm's Law acts as a vital tool, allowing us to interpret the behavior of electrical components under different conditions. By understanding and applying this law, not only do we ensure the proper function of our circuits, but we also mitigate risks associated with electrical power. As we've dissected these problems, it's clear that Ohm's Law is not just about calculating voltage, current, or resistance; it's about understanding the fundamental dynamics of how electricity flows, making it an essential concept for anyone involved in the realm of electronics.
Why is Ohm’s Law important?
+
Ohm’s Law is fundamental because it provides a simple and reliable way to predict the behavior of electrical circuits, ensuring safety, efficiency, and effectiveness in design.
Can Ohm’s Law be applied to all types of circuits?

+
Yes, Ohm’s Law applies to all passive, linear components like resistors, but complex electronics with non-linear behavior may require additional laws and principles.
What if my measurements do not align with Ohm’s Law?

+
If measurements deviate from Ohm’s Law predictions, factors like temperature changes, nonlinear materials, or circuit imperfections could be influencing the measurements.