5 Easy Steps to Multiply Mixed Fractions Instantly

The art of multiplying mixed fractions may seem daunting, but with the right techniques, it can become an intuitive and manageable task. Whether you're helping your child with homework or brushing up your own math skills, understanding how to multiply mixed fractions effectively is a valuable skill. In this post, we'll explore five easy steps to get you multiplying mixed fractions in no time.
1. Convert Mixed Fractions to Improper Fractions
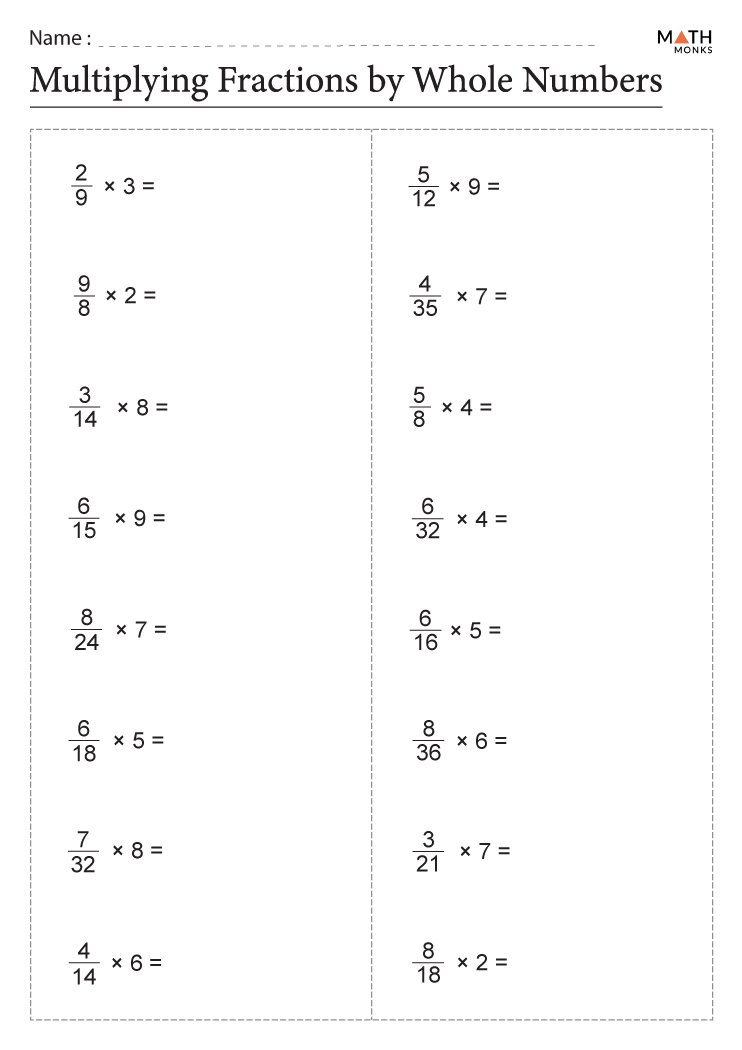
First, let’s discuss what a mixed fraction is. It’s a combination of a whole number and a fraction. Here’s how to convert:
- Multiply the whole number by the denominator of the fraction.
- Add the numerator to this product.
- Place this result over the original denominator.
For example, converting 2 \frac{3}{5} to an improper fraction:
- 2 (whole number) * 5 (denominator) = 10
- 10 + 3 (numerator) = 13
- So, 2 \frac{3}{5} becomes \frac{13}{5}
2. Multiply the Numerators and Denominators

Once you have your improper fractions, follow these steps:
- Multiply the numerators together to get the new numerator.
- Multiply the denominators together to get the new denominator.
Let's take \frac{13}{5} and multiply by another fraction, say \frac{2}{3}:
- Numerator: 13 * 2 = 26
- Denominator: 5 * 3 = 15
So, \frac{13}{5} * \frac{2}{3} = \frac{26}{15}
3. Simplify the Result

Now that you have your fraction, it’s crucial to simplify it:
- Find the greatest common divisor (GCD) of the numerator and the denominator.
- Divide both by this number.
If we take \frac{26}{15}, the GCD of 26 and 15 is 1 (since they are coprime), so the fraction can't be simplified further.
4. Convert Back to Mixed Fraction if Needed

Not all results need to remain in improper form. Here’s how to convert back:
- Divide the numerator by the denominator to find the whole number part.
- Use the remainder as the new numerator over the original denominator.
Using our example:
- 26 ÷ 15 = 1 (whole number) with a remainder of 11
- So \frac{26}{15} becomes 1 \frac{11}{15}
5. Check Your Work

Double-checking your work ensures accuracy:
- Re-convert to improper fractions and multiply them again.
- Verify that your simplified result matches this new calculation.
This step is vital, especially in real-world applications where precision matters.
📝 Note: Practice makes perfect. The more you multiply mixed fractions, the more intuitive the process becomes.
Mastering the multiplication of mixed fractions opens the door to a deeper understanding of numbers and their relationships. With these straightforward steps, you can tackle any mixed fraction multiplication problem, whether for educational purposes, personal knowledge, or real-life applications like cooking or DIY projects.
Why do we convert mixed fractions to improper fractions before multiplying?

+
Converting mixed fractions to improper fractions simplifies the multiplication process, ensuring that you can apply the rule for multiplying fractions directly, which is to multiply the numerators together and the denominators together.
Can mixed fractions be simplified?

+
Yes, after multiplication, you simplify the resulting improper fraction by finding the greatest common divisor (GCD) and dividing both the numerator and denominator by this GCD.
What’s the benefit of converting back to a mixed fraction?
+
Converting back to a mixed fraction can make the result more readable and meaningful in everyday contexts, like when you need to express a number in a whole part and a fraction part.



