5 Ways to Master Multiplying Fractions Easily
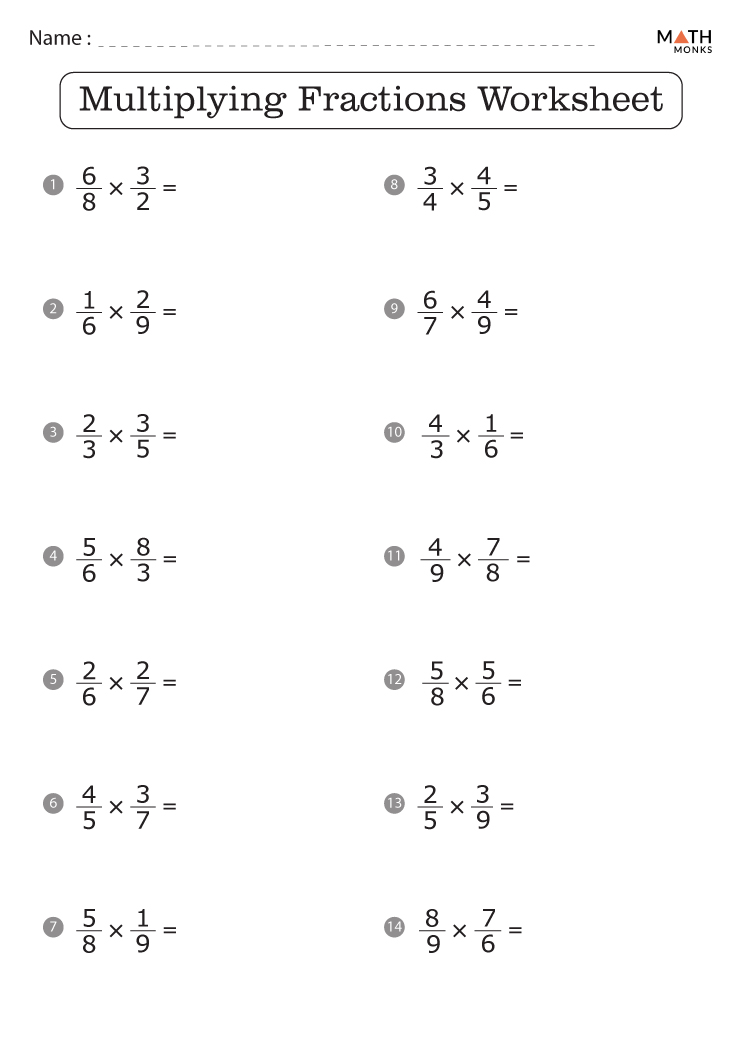
Multiplying fractions is often seen as one of the more challenging arithmetic operations due to the rules and additional steps involved. However, with some practice and understanding, mastering this skill can be both straightforward and empowering. In this blog post, we'll explore five ways to master multiplying fractions easily, ensuring you not only understand the concept but also become adept at performing the operation efficiently.
Understanding the Basics of Fraction Multiplication


Before diving into specific strategies, it's crucial to understand the foundation of multiplying fractions. Here’s a quick recap:
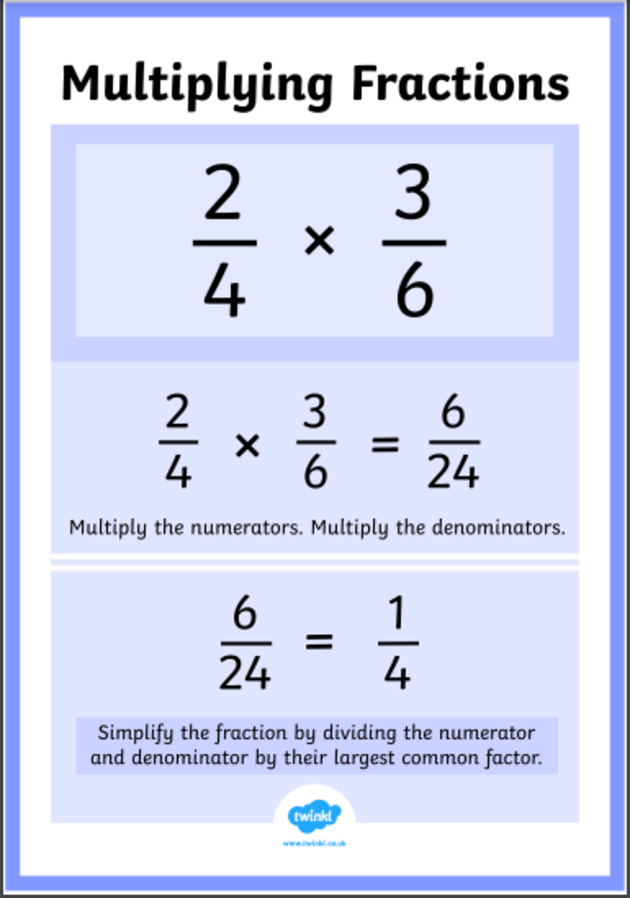
- Multiplying Two Fractions: Multiply the numerators together to get the new numerator, then multiply the denominators together to get the new denominator. The result can be further simplified if possible.
Example: 3/4 * 2/5 = (3 * 2) / (4 * 5) = 6/20 = 3/101. Use Visual Aids and Models

Visual aids can demystify the process of multiplying fractions. Here's how:
- Create a grid or rectangle with dimensions corresponding to the fractions you want to multiply. This helps in visualizing what "a part of a part" means.
- Color-coding can make the process even more intuitive. For example, if you are multiplying 1/2 by 3/4, color in half of a rectangle, then within that half, color in three parts out of four.
This visual method not only helps in understanding but also in checking your math work for accuracy.
2. Apply Cross-Cancellation
Cross-cancellation is a technique that simplifies the multiplication process by reducing the numbers before you actually multiply:
- Look at the numerators and denominators of the fractions you're about to multiply.
- If any numerator from one fraction can be divided by a denominator of the other, cross it out and write the quotient.
Example: 4/5 * 3/8
Cross-cancel: 4/5 * 3/8 = (1) / (5 * 2/3) = 3/10✏️ Note: Cross-cancellation reduces the numbers involved in the calculation, making it easier and quicker.
3. Use the Butterfly Method

The butterfly method offers a memorable way to multiply fractions:
- Multiply diagonally to the left (numerator to numerator and denominator to denominator) to get the new numerator.
- Multiply diagonally to the right to get the new denominator.
- Simplify the fraction if possible.
| Butterfly Steps | Example |
|---|---|
| Multiply the numerators together. | 2/3 * 4/5 → 2 * 4 = 8 (numerator) |
| Multiply the denominators together. | 2/3 * 4/5 → 3 * 5 = 15 (denominator) |

4. Practice with Real-life Examples

Applying math to real-life scenarios not only makes learning fun but also helps in grasping the concepts. Here are some examples:
- If you have 2/3 of a pizza and you decide to split that portion with a friend who takes 1/2 of it, how much pizza do you have left?
- Suppose you're scaling a recipe that serves 4 to serve 6; how do you adjust the ingredient quantities?
5. Digital Tools and Apps for Interactive Learning

Modern educational technology has brought us tools that can make learning math interactive:
- Online calculators with visual explanations.
- Mobile apps with gamified math problems.
- Videos and tutorials explaining multiplication of fractions in an engaging manner.
In summary, multiplying fractions can be simplified through various methods tailored to different learning styles. Whether you prefer visual aids, mathematical shortcuts like cross-cancellation, or interactive learning tools, there's a strategy out there for everyone. Remember, the key to mastering multiplication of fractions lies in understanding the basics, practicing with real-life examples, and utilizing the tools available to make the learning process enjoyable and effective.
Why is it important to simplify fractions after multiplying?

+
Simplifying fractions reduces the numbers, making calculations easier and providing a clearer understanding of the relationship between quantities. It’s also necessary for consistent comparisons and operations.
Can you multiply a whole number by a fraction?

+
Yes, you can. Convert the whole number into a fraction by placing it over 1, then follow the standard fraction multiplication rules.
What if the denominators are different?

+
When multiplying fractions with different denominators, you do not need to find a common denominator before multiplying. Simply multiply the numerators and the denominators, and then simplify if possible.



