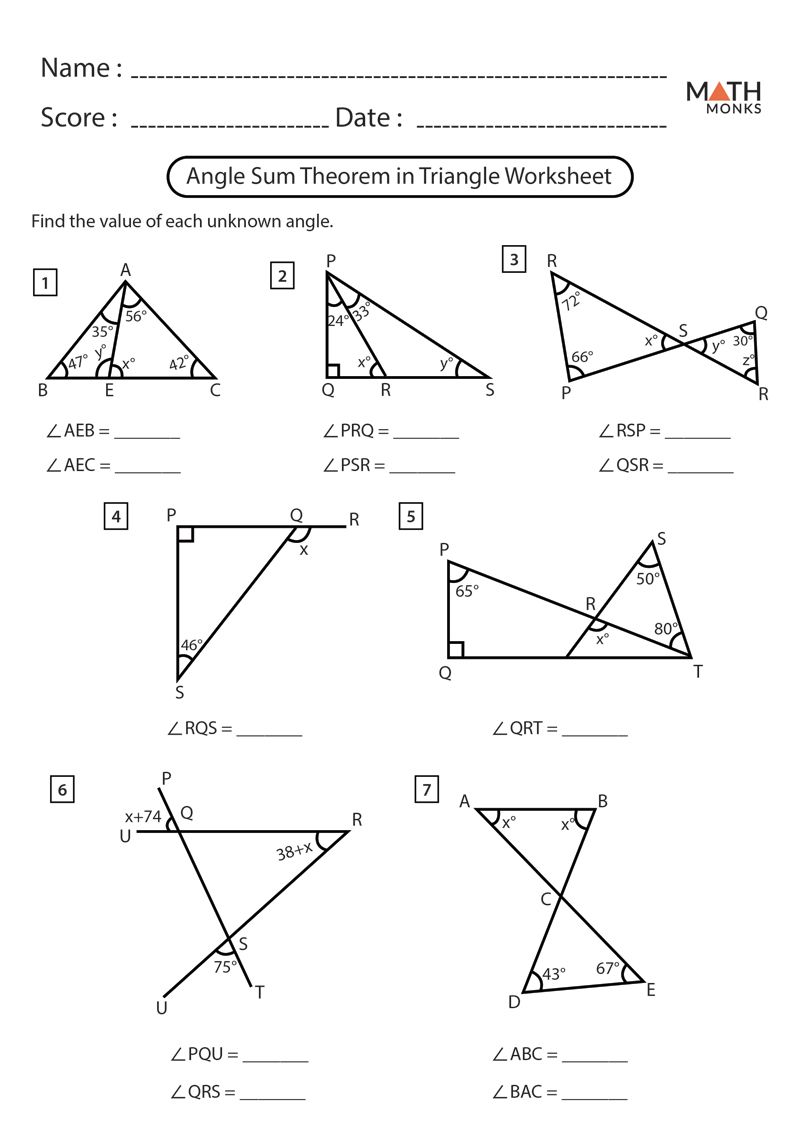
Multiplying Fractions Mixed Numbers Worksheet

Understanding how to multiply fractions, especially when mixed numbers are involved, can be a daunting task for many students. It's an essential skill, however, for tackling more complex math problems in algebra, calculus, and beyond. This comprehensive guide will walk you through the steps of multiplying fractions and mixed numbers, including how to convert mixed numbers to improper fractions, the multiplication process, and then back to mixed numbers when necessary. Let's dive in to make this math topic more approachable and perhaps even enjoyable!

What are Fractions and Mixed Numbers?

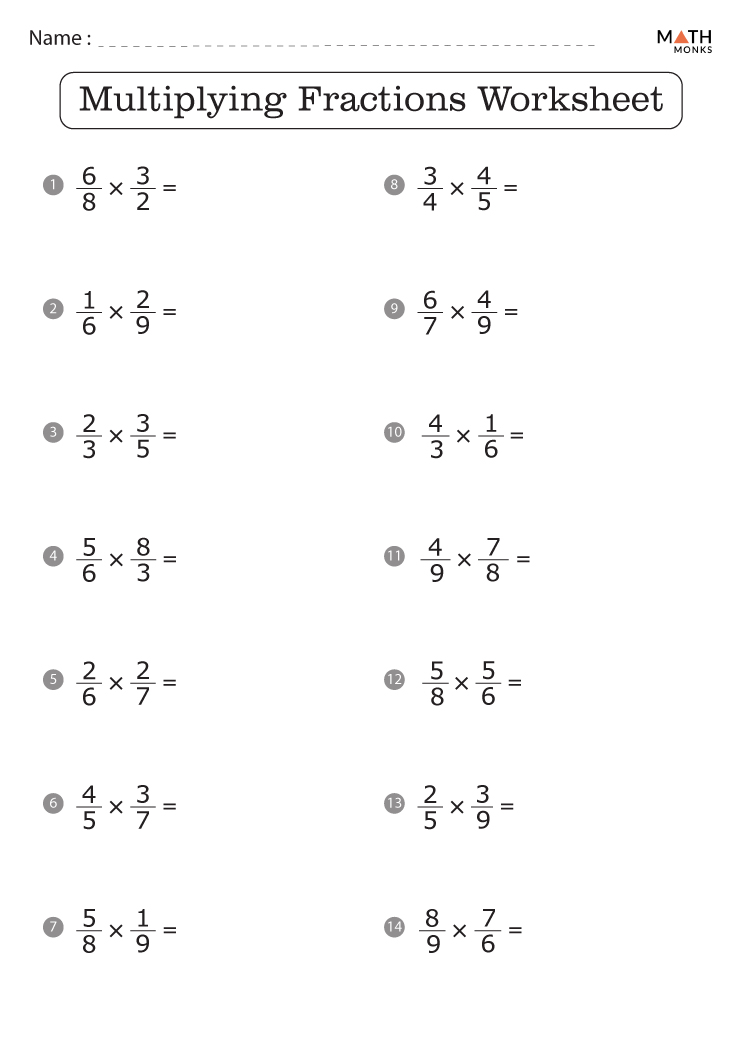
Before we delve into multiplication, let's clarify what we're working with:

• Fractions: A fraction represents a part of a whole. It consists of a numerator (top number) and a denominator (bottom number).
• Mixed Numbers: These combine a whole number with a fraction. For example, 2 1/3 is a mixed number.

📘 Note: Always ensure that you're working with the same units when performing mathematical operations with fractions and mixed numbers.

Steps to Multiply Fractions

Here are the steps to multiply fractions:

1. Convert Mixed Numbers to Improper Fractions: If your numbers are mixed, you first need to convert them to improper fractions. Here's how:
   • Multiply the whole number by the denominator.
   • Add this product to the numerator.
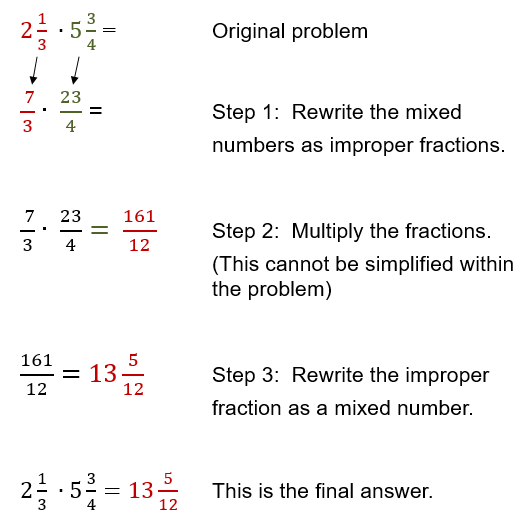
   • Place the result over the denominator. Here’s an example for 2 1/3:

             2 * 3 = 6 
             6 + 1 = 7 
             7/3
             

2. Multiply the Numerators Together: Take the numerators from both fractions and multiply them.
3. Multiply the Denominators Together: Do the same with the denominators.
4. Simplify Your Result: Reduce the fraction if possible. For example:

       (7/3) * (4/5) = (7*4) / (3*5) = 28/15
       

   Here, 28/15 is already in simplest form.

Converting Back to Mixed Numbers

Sometimes, the result of multiplication needs to be expressed as a mixed number. Here's how you do that:

1. Divide the numerator by the denominator.
2. The quotient becomes the whole number.
3. The remainder over the denominator is the fraction. For example, converting 28/15:
   • 28 ÷ 15 = 1 remainder 13
   • The mixed number is 1 13/15.

📘 Note: Remember that simplification might already have made the fraction in simplest form, so converting back to a mixed number may not always be necessary.

Examples of Multiplying Fractions and Mixed Numbers

Let's work through a couple of examples to solidify the process:

Example 1: Multiplying Two Fractions

Let’s multiply ³⁄₄ by ¹⁄₂:

• Numerator: 3 * 1 = 3
• Denominator: 4 * 2 = 8
• The result is ³⁄₈.

Example 2: Multiplying a Fraction and a Mixed Number

Now let’s multiply ³⁄₄ by 2 ¹⁄₃:

• Convert 2 ¹⁄₃ to improper fraction: 2 ¹⁄₃ = (2*3 + 1) / 3 = ⁷⁄₃
• Numerator: 3 * 7 = 21
• Denominator: 4 * 3 = 12
• Result: ²¹⁄₁₂, simplify to ⁷⁄₄
• Convert ⁷⁄₄ back to mixed number: 7 ÷ 4 = 1 remainder 3; result is 1 ³⁄₄

Practical Applications

Here are some scenarios where you might need to multiply fractions and mixed numbers:

• Cooking: Adjusting recipes, especially when scaling for more or fewer servings.
• Construction: Calculating dimensions or materials required for part of a larger project.
• Measurements: Reducing or expanding measurements for sewing or tailoring.

📘 Note: In practical applications, often rounding off to the nearest practical number might be required.

In conclusion, multiplying fractions and mixed numbers involves a few steps of conversion, multiplication, and potentially simplification back to mixed numbers. It might seem complex at first, but with practice, these steps become more intuitive, enhancing not just your math skills but also your problem-solving abilities in real-world scenarios. Whether you're cooking, building, or simply solving a math problem, mastering these techniques can significantly broaden your mathematical toolkit.

What is the difference between a fraction and a mixed number?

+

A fraction represents a part of a whole, while a mixed number is a whole number combined with a fraction. For example, ¹⁄₂ is a fraction, and 1 ¹⁄₂ is a mixed number.

Why do we convert mixed numbers to improper fractions?

+

Converting mixed numbers to improper fractions allows us to perform operations like multiplication more straightforwardly since standard fraction rules apply.

Can you multiply a fraction by a whole number?

+

Yes, you can multiply a fraction by a whole number by treating the whole number as a fraction with a denominator of 1. For example, 3 * (¹⁄₄) = ³⁄₁ * ¹⁄₄ = ³⁄₄.

What’s the benefit of knowing how to multiply mixed numbers?

+

Multiplying mixed numbers is vital for advanced calculations in areas like measurements, cooking, and engineering where quantities often involve mixed numbers or fractions.

How do you simplify a fraction?

+

To simplify a fraction, find the greatest common divisor (GCD) of the numerator and denominator, and divide both by this GCD. If there is no common divisor other than 1, the fraction is already in its simplest form.