5 Ways to Teach Multiplying Fractions by Fractions

When teaching multiplication of fractions by fractions to young students or anyone new to math, the process can seem daunting at first. However, with clear and concise teaching methods, anyone can grasp this concept effectively. This article will explore five ways to simplify and teach this essential mathematical skill.
Method 1: Understanding Through Visual Aids
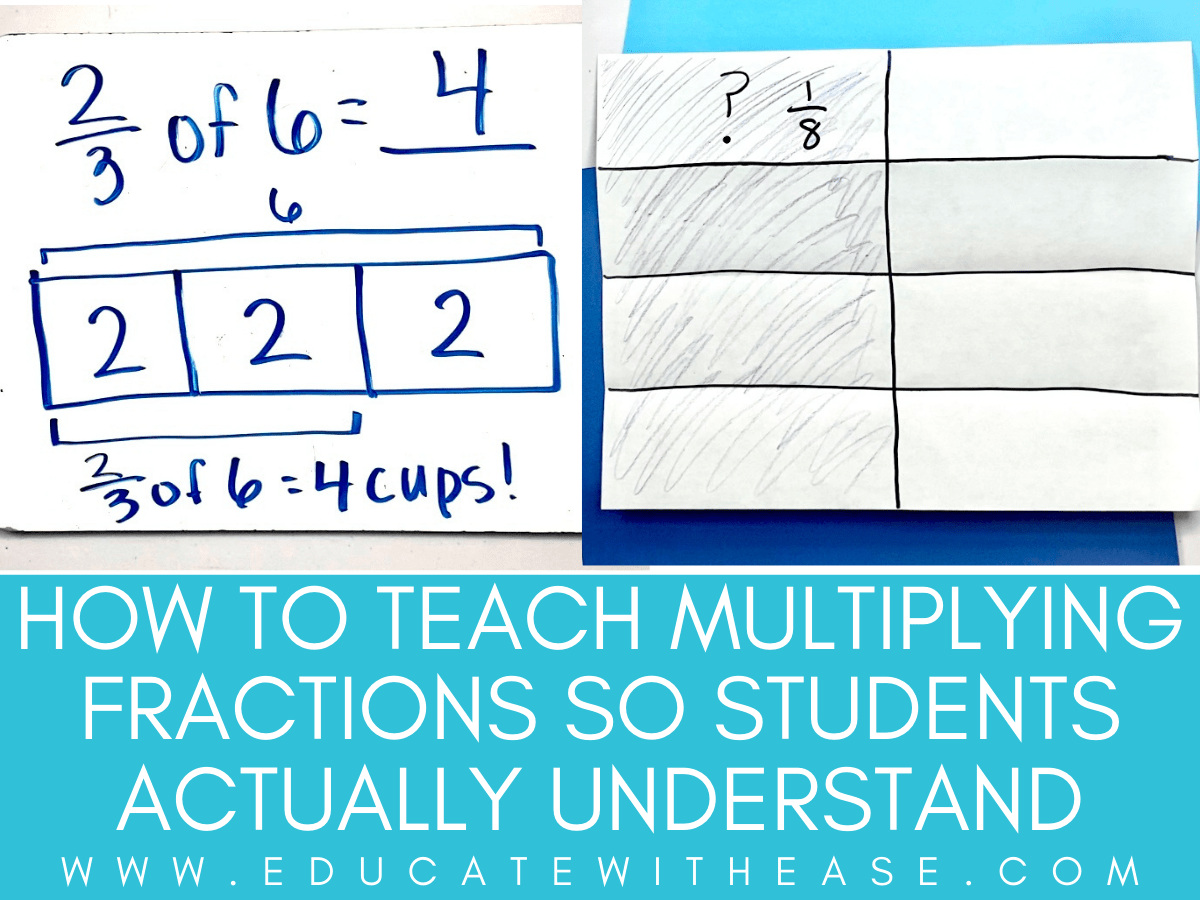
Visual learning is incredibly effective, especially when it comes to math. Here’s how to use visual aids to teach fraction multiplication:
- Using Fraction Strips: Provide students with strips that can be folded to represent different fractions. Show how overlapping these strips can visually depict multiplication.
- Pizza Models: Use diagrams of pizzas or pies where students can visualize cutting each slice further into smaller pieces, representing the multiplication of fractions.
- Area Models: This involves creating a grid to represent two fractions and shading the overlap to demonstrate the product. It’s a step-by-step visual journey through the multiplication process.

🧩 Note: Visual aids are particularly helpful for students who have difficulty with abstract math concepts.
Method 2: The Rule Method

Simplify the task by teaching the straightforward rule for multiplying fractions:
- Multiply the numerators together to get the new numerator.
- Multiply the denominators together to get the new denominator.
- Optional: Simplify the resulting fraction if possible.
This method can be directly applied to problems, showing that multiplying fractions does not always require the same extensive steps as adding or subtracting them.
Method 3: Use of Real-Life Examples

Linking math to everyday scenarios can boost comprehension. Here are a few examples:
- If a recipe calls for ½ of an ingredient, but you need ¾ of the recipe, how much of the ingredient do you use? Here, students multiply ½ by ¾.
- Discussing how much of a flag would be visible if one part is covered by a flag with another fraction of its surface.
- Explaining how much work is done if one worker completes ⅔ of a task, and another worker then does ⅖ of that portion.
Method 4: Interactive Games and Applications

Interactive learning through games can engage students in a fun and educational manner:
- Fraction Matching Games: Use cards or online applications where students match fractions to their products.
- Virtual Manipulatives: Digital tools where students can drag, drop, and adjust fractions visually.
- Online Quizzes and Competitions: Platforms where students can compete in real-time, promoting friendly competition and practice.
Method 5: Procedural Understanding with Repetition

The key here is to ensure that students understand why the steps work:
- Explain how multiplying by a fraction less than one makes the whole smaller.
- Discuss why the product of two positive fractions is always less than either of the fractions multiplied.
- Reiterate the steps with various examples, asking students to solve and explain each step.
Consistent practice through homework, quizzes, and in-class exercises can cement this understanding.
In sum, teaching the multiplication of fractions can be approached in numerous ways, each tailored to different learning styles. Whether through visual aids, straightforward rules, real-life applications, interactive games, or procedural practice, the goal is to make the process intuitive and accessible. These methods not only help in grasping the concept of multiplying fractions but also in fostering a love for math.
Why do we multiply the numerators and denominators separately when multiplying fractions?

+
This method is derived from understanding what a fraction represents. When you multiply two fractions, you are finding what portion of a portion you have, which effectively results in a new portion of the whole. Multiplying numerators gives you the part you’re interested in, while multiplying the denominators gives you the new context or total.
Can you simplify fractions before multiplying them?

+
Yes, it’s often easier to simplify fractions before multiplying to keep the numbers manageable. However, simplification before multiplication is optional, as you can simplify the resulting fraction afterwards, or even multiply first and then simplify the product.
What are some common mistakes when teaching multiplying fractions?

+
Common mistakes include forgetting to multiply both numerators and denominators, incorrectly dividing instead of multiplying, and not simplifying the result when possible. It’s also common for learners to get confused about when to simplify or cross-reduce, especially when fractions are fractions within fractions.



