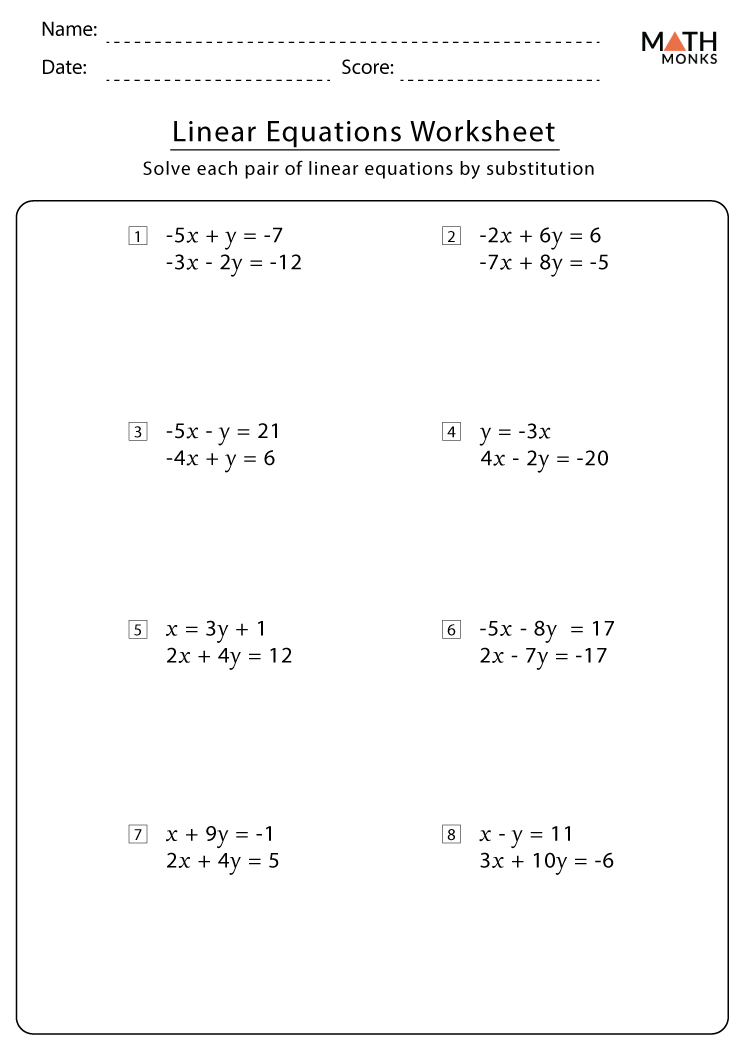
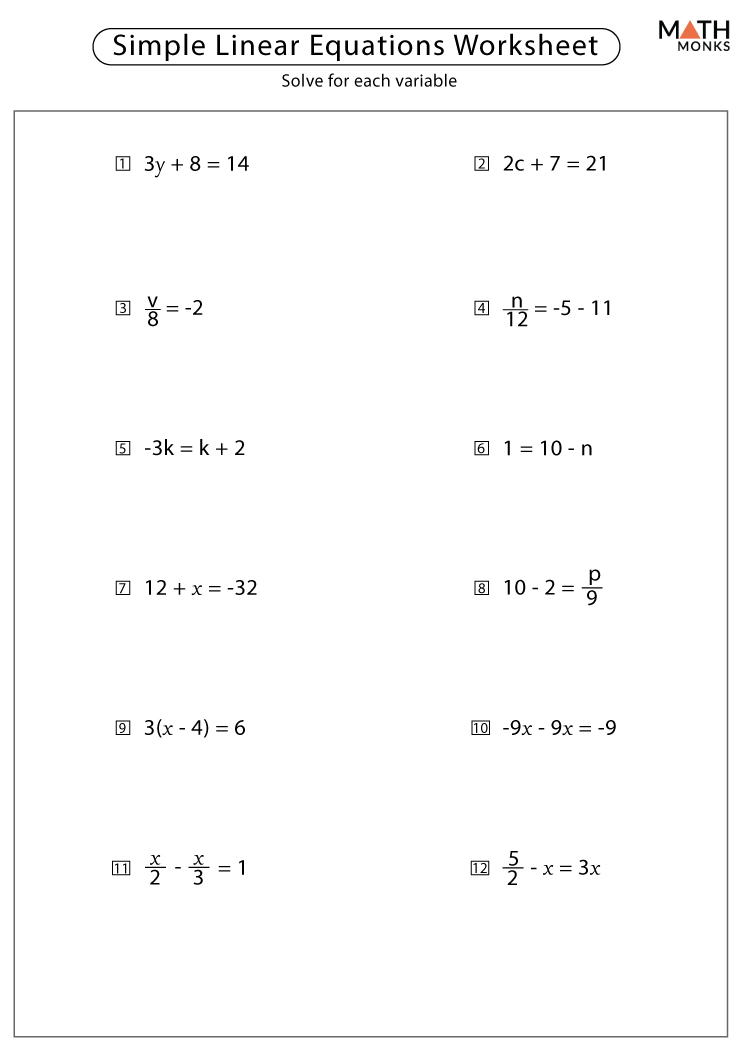
Master Multi Step Linear Equations: Free Worksheet

Learning how to solve multi-step linear equations is a critical skill in algebra that can empower you in solving a wide array of mathematical problems. These equations require a combination of algebraic techniques, including distribution, combining like terms, and isolating the variable to find its value. In this comprehensive guide, we'll delve into the techniques necessary for mastering multi-step linear equations, and we'll also provide a free worksheet to help you practice.
Why Multi-Step Linear Equations Matter

Multi-step linear equations are foundational to understanding more complex algebraic concepts. Here’s why mastering them is beneficial:
- Enhances problem-solving skills: The structured approach to solving multi-step equations improves your logical thinking and problem-solving capabilities.
- Prepares for higher mathematics: Skills in multi-step equations are essential for tackling calculus, statistics, and advanced algebra.
- Real-world applications: From financial calculations to physical modeling, multi-step equations are omnipresent in practical situations.
Solving Multi-Step Linear Equations: The Basics

The key to solving these equations is to isolate the variable by following a sequence of steps:
Step 1: Distribute
If there’s an expression outside the parentheses, distribute it inside:
- Example: 2(x + 3) = 2x + 6
Step 2: Combine Like Terms

Add or subtract terms to simplify the equation:
- Example: 2x + 6 + 4x = 6x + 6
Step 3: Move Variables to One Side

Get all variable terms on one side and constants on the other:
- Example: 6x + 6 - 6x = 6, which simplifies to 6 = 0 (contradiction indicating no solution)
Step 4: Isolate the Variable

Perform operations to isolate the variable:
- Example: 3x = 9, then divide both sides by 3 to get x = 3
Step 5: Check Your Work

Substitute the solution back into the original equation to verify:
- Example: 3(3) = 9 confirms that x = 3 is indeed a solution.
🔍 Note: Always verify your solutions to ensure they are correct.
Common Mistakes to Avoid

- Not distributing correctly: Missing terms inside parentheses can lead to incorrect solutions.
- Combining terms incorrectly: Always look for like terms to simplify.
- Isolating the variable with wrong operations: Remember to apply the same operation to both sides of the equation.
- Failing to verify solutions: A step often overlooked but crucial for accuracy.
Free Worksheet for Practice

To put these techniques into practice, we’ve prepared a free worksheet with multi-step linear equations. Here’s a sample question:
| Problem | Solution |
|---|---|
| 2(3x + 5) = 8 - 2x | Distribute: 6x + 10 = 8 - 2x |

Download the worksheet to practice more of these equations on your own. Regular practice is key to mastering multi-step linear equations.
In conclusion, solving multi-step linear equations is an essential skill that opens up avenues for deeper mathematical understanding. By breaking down these equations into manageable steps, you not only solve for the unknown but also enhance your logical reasoning. Remember, practice makes perfect, and with the provided worksheet, you have all the tools you need to excel in this area of algebra.
What is the first step in solving a multi-step linear equation?
+
The first step often involves distributing if there are any brackets or parentheses in the equation.
Why is it important to verify the solution to an equation?

+
Verification confirms that the solution works in the original equation, ensuring accuracy.
Can an equation have multiple solutions?

+
In the context of linear equations, there is typically one solution, no solution, or infinitely many solutions. Multi-step equations, in particular, tend to have one unique solution or none at all.



